Delta-function

-function, Dirac delta-function,

A function which makes it possible to describe the spatial density of a physical magnitude (mass, charge, intensity of a heat source, force, etc.) which is concentrated or applied at a point of a space . For instance, using the delta-function the density of a point mass located at a point is written as . The delta-function may be formally defined by the relation

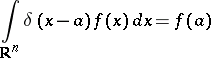for any continuous function 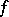. The derivatives 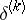of the delta-function may be defined in a similar manner:

for the class of functions 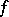that are continuous in with derivatives up to the order inclusive. The formal operator relations, which are frequently employed, and which express the following properties of the delta-function:

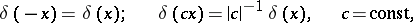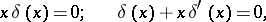etc., should be understood in the sense of the above definitions, i.e. these relations become meaningful only after having been integrated against sufficiently smooth functions. Thus, the delta-function is not an ordinary function in the sense of the classical theory of functions, and is defined in the theory of generalized functions as a singular generalized function, i.e. as the continuous linear functional in the space of infinitely-differentiable functions of compact support, assigning to its value at zero: .

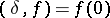Comments

The Dirac delta-function is the derivative (in the sense of distributions or generalized functions) of the Heaviside function (Heaviside distribution) 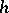, defined by for , for (the value at zero does not matter; as usual for a distribution it suffices for it to be defined apart from a set of measure zero).

References

[a1]	L. Schwartz, "Théorie des distributions" , 1–2 , Hermann (1966)
[a2]	I.M. Gel'fand, G.E. Shilov, "Generalized functions" , 1. Properties and operations , Acad. Press (1964) (Translated from Russian)