Composition series
composition series
A finite subset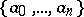 
of a partially ordered set with least element 
and greatest element 
such that
 |
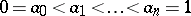and all the intervals 
are simple (elementary) (cf. Elementary interval). One can also speak of a composition series of an arbitrary interval 
of a partially ordered set. Composition series certainly do not always exists.
A composition series of a universal algebra is defined in terms of congruences. Since congruences in groups are defined by normal subgroups, a composition series of a group can be defined as a normal series of it (see Subgroup series) having no proper refinements (without repetition). A series
 |
is a composition series for the group 
if and only if every 
is a maximal normal subgroup in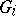 
.
All the factors 
of a composition series are simple groups. Every normal series isomorphic to a composition series is a composition series itself. The Jordan–Hölder theorem holds for composition series of groups. Composition series of rings, and more generally of 
-groups, are defined in a similar way and have similar properties (see [2]).
References
| [1] | P.M. Cohn, "Universal algebra" , Reidel (1981) |
| [2] | A.G. Kurosh, "Lectures on general algebra" , Chelsea (1963) (Translated from Russian) |
Comments
For a universal algebra the notion of a composition series is more precisely defined as follows [1]. Let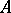 
be an 
-algebra and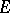 
a subalgebra. A normal chain from 
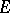to 
is then a finite chain of subalgebras of 
,
 |
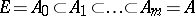together with a congruence 
on 
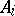for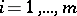 
such that 
is precisely a 
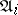-class. There is a natural notion of refinement and isomorphism of normal chains: normal chains from 
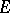to 
are isomorphic if and only if they are equally long and if there is a permutation of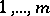 
such that 
. Then one has the Schreier refinement theorem to the effect that if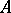 
is an 
-algebra with subalgebra 
such that on any subalgebra of 
all congruences commute, then any two normal chains from 
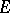to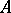 
have isomorphic refinements, and the Jordan–Hölder theorem that any two composition series from 
to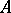 
on such an algebra are isomorphic.
A subgroup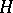 
of a group 
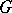is called subnormal if there is a chain of subgroups 
such that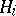 
is normal in 
, 
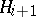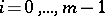. Consider the lattice of subnormal subgroups 
of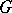 
. Then a composition series for the partially ordered set 
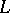defines in fact a composition series for 
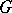, and vice versa. Something analogous can be formulated for universal algebras (These statements of course do not hold for, respectively, the lattice of normal subgroups and the lattice of congruences.)
References
| [a1] | B. Huppert, "Endliche Gruppen" , 1 , Springer (1967) |
Composition series. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Composition_series&oldid=27314