Hyperbolic trigonometry
From Encyclopedia of Mathematics
The trigonometry on the Lobachevskii plane (cf. Lobachevskii geometry). Let 
, 
, be the lengths of the sides of a triangle on the Lobachevskii plane, and let 
be the angles of this triangle. The following relationship (the cosine theorem), which relates 
with 
, is valid:
 |
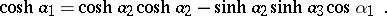All the remaining relations of hyperbolic trigonometry follow from this one, such as the so-called sine theorem:
 |
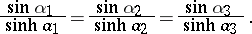Comments
References
| [a1] | H.S.M. Coxeter, "Non-Euclidean geometry" , Univ. Toronto Press (1965) pp. 224–240 |
| [a2] | H.S.M. Coxeter, "Angles and arcs in the hyperbolic plane" Math. Chronicle (New Zealand) , 9 (1980) pp. 17–33 |
How to Cite This Entry:
Hyperbolic trigonometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hyperbolic_trigonometry&oldid=27277
Hyperbolic trigonometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hyperbolic_trigonometry&oldid=27277
This article was adapted from an original article by A.B. Ivanov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article