Convergence of measures

2020 Mathematics Subject Classification: Primary: 28A33 [MSN][ZBL]

A concept in measure theory, determined by a certain topology in a space of measures that are defined on a certain -algebra of subsets of a space or, more generally, in a space of charges, i.e. countably-additive real or complex functions , defined on sets from . The following are the most commonly used topologies in the subspace consisting of bounded charges, i.e. charges for which , .

1) In the norm

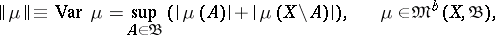called the variation of the charge 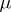, is introduced. The convergence of a sequence of charges , , to a charge 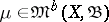in this norm is called convergence in variation.

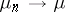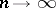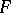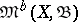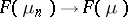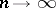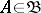2) In the ordinary weak topology is examined: Convergence of a sequence of charges , , in this topology (weak convergence) means that for any continuous linear function on , , . This convergence is equivalent to the fact that the sequence of charges is bounded, , and that for any set the sequence of values 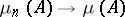, 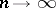. 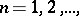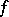Weak convergence of a sequence of charges , implies convergence of the integrals , , for any bounded function on that is measurable with respect to the 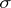-algebra .

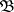3) When is a topological space and is its Borel -algebra, a topology is examined in which is also called the weak topology (or sometimes the narrow topology). It is defined as the weakest of the topologies in 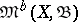relative to which all functionals of the form

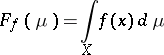are continuous, where 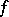is an arbitrary bounded continuous function on . This topology is weaker than the previous one, and convergence of a sequence of charges , , relative to it (weak or narrow convergence) is equivalent to the convergence of the values 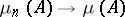, , 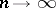for any Borel set 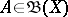for which 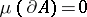, where 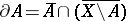and the operation of closure of a set is denoted by the bar.

4) When is a locally compact topological space (and 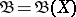is a Borel -algebra) in the so-called wide topology is examined: the convergence of a sequence of charges 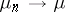, 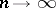(wide convergence), means convergence of the functionals , , for any continuous function 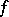with compact support. This topology is weaker than the weak topology in 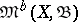. An analogous topology is defined naturally in the wider space 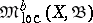of locally bounded charges 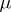, i.e. charges such that for any point there is a neighbourhood in which , , .

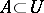References

[Bo]	N. Bourbaki, "Elements of mathematics. Integration" , Addison-Wesley (1975) pp. Chapt.6;7;8 (Translated from French) MR0583191 Zbl 1116.28002 Zbl 1106.46005 Zbl 1106.46006 Zbl 1182.28002 Zbl 1182.28001 Zbl 1095.28002 Zbl 1095.28001 Zbl 0156.06001
[DS]	N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Interscience (1958) MR0117523
[Bi]	P. Billingsley, "Convergence of probability measures" , Wiley (1968) MR0233396 Zbl 0172.21201