Karamata theory

The basic form of the theory of regular variation, a subject initiated in 1930 by the Yugoslav mathematician J. Karamata.

Viewed from a modern perspective, Karamata theory is the study of asymptotic relations of the form

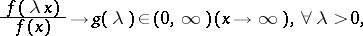(a1)

together with their consequences and ramifications. The case is particularly important; measurable functions satisfying (a1) with are called slowly varying; such slowly varying functions are often written or (for "lente" ).

Many useful and interesting properties are implied by such relations. For instance:

i) The uniform convergence theorem: for slowly varying, (a1) holds uniformly on compact -sets in . There is a topological analogue, with measurability replaced by the Baire property.

ii) The representation theorem: is slowly varying if and only if, for large enough, is of the form

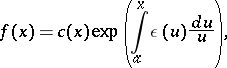where 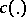, are measurable, , as .

iii) The characterization theorem: for measurable , in (a1) must be of the form for some , called the index of regular variation: . Then 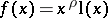with slowly varying ().

iv) Karamata's theorem: if and , then

(a2)

(That is, the in 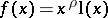"behaves asymptotically like a constant" under integration.) Conversely, (a2) implies .

Perhaps the most important application of Karamata theory to analysis is Karamata's Tauberian theorem (or the Hardy–Littlewood–Karamata theorem): if () is increasing, with Laplace–Stieltjes transform 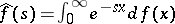, then () with , if and only if .

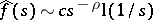
For details, background and references on these and other results, see e.g. [a1], Chap. 1.

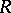The union over all of the classes gives the class of regularly varying functions. This is contained in the larger class of extended regularly varying functions, itself included in the class of -regularly varying functions: . Just as a function has an index 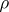of regular variation, and then , so a function has a pair of upper and lower Karamata indices (and these are equal, to 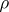say, if and only if 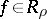), and a function has a pair of upper and lower Matuszewska indices. These larger classes , have analogues of the results above; for instance, uniform convergence and representation theorems. For details, see e.g. [a1], Chap. 2.

Karamata theory may be regarded as the "first-order" theory of regular variation. There is a corresponding "second-order" theory: de Haan theory [a1], Chap. 3.

Karamata theory has found extensive use in several areas of analysis, such as Abelian, Tauberian and Mercerian theorems ([a1], Chap. 4, 5; cf. also Tauberian theorems; Mercer theorem; Abel theorem) and the Levin–Pfluger theory of completely regular growth of entire functions ([a1], Chap. 6; cf. also Entire function), and is also useful in asymptotic questions in analytic number theory [a1], Chap. 7. It has been widely used also in probability theory, following the work of W. Feller [a2]; [a1], Chap. 8.

References

[a1]	N.H. Bingham, C.M. Goldie, J.L. Teugels, "Regular variation" , Encycl. Math. Appl. , 27 , Cambridge Univ. Press (1989) (Edition: Second)
[a2]	W. Feller, "An introduction to probability theory and its applications" , 2 , Springer (1976) (Edition: Second)