Tangent line

to a curve

A straight line representing the limiting position of the secants. Let be a point on a curve (Fig. a). A second point is chosen on and the straight line is drawn. The point is regarded as fixed, and approaches 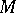along the curve . If, as goes to , the line tends to a limiting line , then is called the tangent to at .

Figure: t092170a

Figure: t092170b

Not every continuous curve has a tangent, since need not tend to a limiting position at all, or it may tend to two distinct limiting positions as tends to 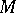from different sides of (Fig. b). If a curve in the plane with rectangular coordinates is defined by the equation 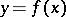and is differentiable at the point , then the slope of the tangent at 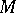is equal to the value of the derivative 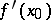at ; the equation of the tangent at this point has the form

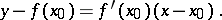The equation of the tangent to a curve in space is

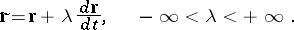By a tangent to a surface at a point 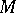one means a straight line passing through and lying in the tangent plane to at .

References

[a1]	M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) (Translated from French)
[a2]	H.S.M. Coxeter, "Introduction to geometry" , Wiley (1961)
[a3]	H.W. Guggenheimer, "Differential geometry" , McGraw-Hill (1963)
[a4]	D. Hilbert, S.E. Cohn-Vossen, "Geometry and the imagination" , Chelsea (1952) (Translated from German)
[a5]	B. O'Neill, "Elementary differential geometry" , Acad. Press (1966)