Movable singular point

A singular point of the solution of a differential equation 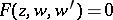( 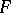is an analytic function), where 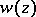is considered as a function of the complex variable , which is such that solutions to the same equation with initial data close to the original data have singular points close to but not coincident with it. The classical example of a movable singular point arises when considering the equation

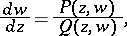where and 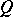are holomorphic functions in a certain region of the space 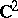. If the surface 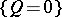is irreducible and is projected along the 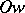-axis on a region 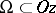, then all points in the region are movable singular points; for the solution with initial condition 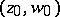, where

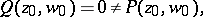the point 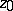is an algebraic branch point.

References

[1]	V.V. Golubev, "Vorlesungen über Differentialgleichungen im Komplexen" , Deutsch. Verlag Wissenschaft. (1958) (Translated from Russian)

Comments

For equations of the form

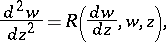where 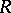is rational in 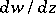and and analytic in , it is known which equations have only non-movable singularities, cf. Painlevé equation and [a1].

References

[a1]	E.L. Ince, "Ordinary differential equations" , Dover, reprint (1956)