Gevrey class

An intermediate space between the spaces of smooth (i.e. -) functions and real-analytic functions. In fact, the name is given in honour of M. Gevrey, who gave the first motivating example (see [a13], in which regularity estimates of the heat kernel are deduced).

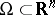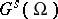Given and , the Gevrey class (of index ) is defined as the set of all functions 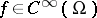such that for every compact subset 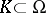there exists a 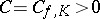satisfying

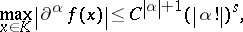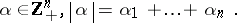For 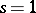one recovers the space of all real-analytic functions on , while for 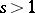, contains non-zero functions, 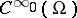being the set of all 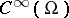-functions with compact support. There are various equivalent ways to define 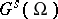(cf. [a27]). Introducing the natural inductive topology on 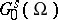, for 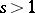, one can define the space 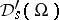of Gevrey -ultra-distributions as the dual to 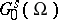. The space of -ultra-distributions contains the Schwartz distributions (cf. also Generalized functions, space of). The Gevrey classes are the most simple case of classes of ultra-differentiable functions (or Denjoy–Carleman classes; see, e.g., [a17]). Since the scale of spaces 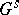starts from the analytic functions (for 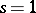) and ends in the 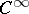-category (setting 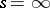), the Gevrey classes play an important role in various branches of partial and ordinary differential equations; namely, whenever the properties of certain differential operators (mappings) differ in the 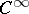and in the analytic category, it is natural to investigate the behaviour of such operators (mappings) in the scale of Gevrey classes 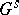and, if possible, to find the critical value(s) of , i.e. those for which a change of behaviour occurs. In particular, all weak solutions to the heat equation 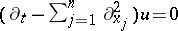are 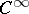, while they are not real-analytic. In the scale of Gevrey spaces, the result is sharp; namely, 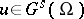for 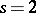(and hence for all 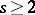), but, in general, 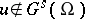if .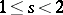

Applications.

The Gevrey classes 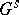, , 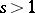have numerous applications, a few of the main applications being listed below.

Gevrey micro-local analysis.

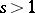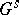For one says that a given -ultra-distribution is (micro-locally) -regular at a point 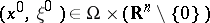if there exist a , , 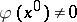an open cone 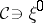in , and a positive constant such that

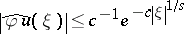for 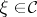, where denotes the Fourier transform of and 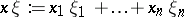. This definition is independent of the choice of 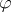. The 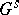wave front set 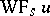of is the smallest closed conic subset of 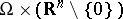such that is 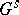-regular at each 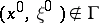. Here, being a conic subset means that implies for all 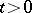. For equivalent definitions, see [a14], [a27].

Let

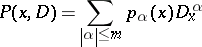be a linear partial differential operator (cf. also Linear partial differential equation), with 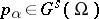, , 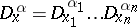. The presence of the imaginary unit 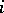allows one to define 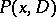via the Fourier transform, namely

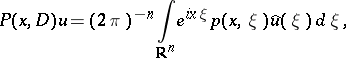with 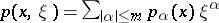. This definition is valid for pseudo-differential operators (cf. Pseudo-differential operator) as well, where 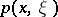is a suitable symbol from the Hörmander classes 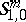or from other classes (see [a27] for more details and references). The characteristic set 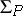of 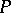is defined by

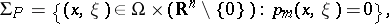with 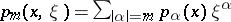standing for the principal symbol (cf. also Symbol of an operator; Principal part of a differential operator). The operator is called 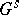-hypo-elliptic (respectively, 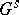-micro-locally hypo-elliptic) in an open set 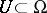(respectively, in an open conic set ) if for every 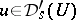satisfying 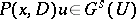(respectively, ) necessarily 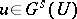(respectively, ).

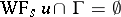Recall that 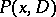is called of principal type if 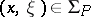implies that 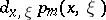is not linearly dependent on 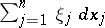. The operator is called of multiple characteristics type if there exists a 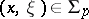such that . The properties of operators of principal type are basically the same in the analytic-Gevrey category and the 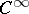-category. An essential difference occurs in the case of multiple characteristics. For operators with constant multiple real or complex characteristics, modelled by 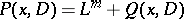, 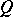being an operator of order 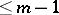while is a first-order operator modelled by 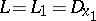or 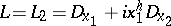, , 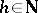the behaviour of 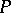in 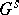, , 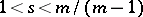is governed by the operator 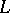, independently of the lower-order terms in . However, if 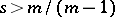, then the lower-order terms affect both the 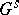-hypo-ellipticity and the propagation of 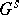singularities, cf. [a5], [a21], [a27]. In fact, often one is interested in finding a critical index such that for 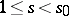and 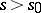certain properties are complementary. 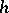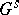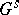In particular, if and is even, there are examples of operators analytic and Gevrey -hypo-elliptic for but not - and Gevrey -hypo-elliptic for large values of the Gevrey index (see [a21], [a27] for more details and references).

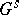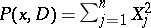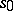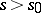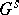As to the -hypo-ellipticity for operators of the form , being analytic vector fields satisfying the Hörmander bracket hypothesis, a typical pattern of behaviour is the following one: There is a critical index such that for , the -hypo-ellipticity of 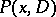holds, while for 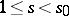it does not (cf. [a2], [a7], [a23]).

Gevrey singularities appear in the study of initial-boundary value problems for hyperbolic equations in domains with analytic diffractive boundaries (cf. [a18] and the references therein). In particular, for the wave equation, Gevrey 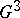-singularities along the diffractive analytic boundary appear, and this fact is used in scattering theory (cf. [a1]).

Gevrey solvability.

The operator 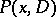is called (locally) -solvable in if for every 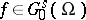there exists a 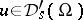such that .

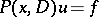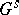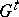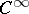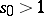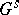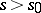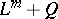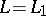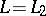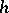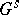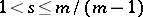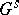Since -solvability implies -solvability for , when is not solvable in the -category one looks for an index such that the operator is -solvable for and not for . The model operators , with or and being even, are -solvable for , while for they need not be -solvable (cf. [a6], [a21], [a27]).

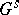-solvability for semi-linear partial differential operators, provided 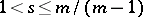, is proved in [a12].

Hyperbolic equations.

The Gevrey classes serve as a framework for the well-posedness of the Cauchy problem for weakly hyperbolic linear partial differential operators (cf. also Linear hyperbolic partial differential equation and system)

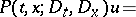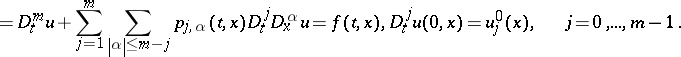Weak hyperbolicity means that the roots of 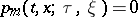with respect to are real. If 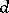is the maximal multiplicity of the real roots in , then the Cauchy problem is always well-posed in the framework of the Gevrey classes 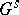, provided . If 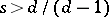, one can point out specific lower-order terms such that the existence fails. More subtle estimates for the critical Gevrey index are obtained by using the distance between the roots or via additional restrictions on the lower-order terms (so-called Levi-type conditions). See [a3], [a14], [a4], [a15], [a19], [a24], [a21] for more details and references. Local Gevrey well-posedness for weakly hyperbolic non-linear systems is shown in [a16] (see also [a12]). Goursat problems for Kirchoff-type equations in Banach spaces of Gevrey functions (cf. also Kirchhoff formula; Goursat problem) have been studied in [a10].

Divergent series and singular differential equations.

One may also define formal Gevrey spaces , e.g. the set of all formal power series

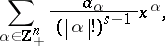where for some 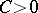the following estimates hold:

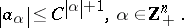Such formal Gevrey spaces are used in the study of divergent series and singular ordinary linear differential equations with Gevrey coefficients (see [a26] and the references therein). The Fredholm property in such type of Gevrey spaces of certain singular analytic partial differential operators in 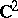has been studied by means of Toeplitz operators (cf. [a22]).

Dynamical systems.

The framework of Gevery classes is used in the study of normal forms of analytic perturbations of (non-) integrable (non-) Hamiltonian systems. Roughly speaking, one obtains normal forms modulo exponentially small error terms of the type 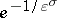, where 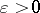is small parameter, while 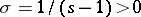is related to Gevrey-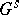-type estimates, or so-called Nekhoroshev-type estimates (see e.g. [a11] for Gevrey normal forms of billiard ball mappings and [a25] on normal forms of perturbations of Hamiltonian systems).

Evolution partial differential equations.

In the study of the analytic regularity of solutions of semi-linear evolution equations (Navier–Stokes, Kuramoto–Sivashinksi, Euler, the Ginzburg–Landau equation) with periodic boundary data for positive time, the term "Gevrey class" is used usually to denote the Banach space 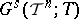, , (with 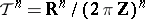being the -dimensional torus) of smooth functions on 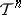with the norm defined by means of the discrete Fourier transform

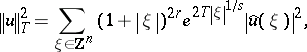for some 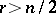. In these applications, the Gevrey index 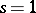(the analytic category). See [a8] for the Navier–Stokes equations; [a9] for recent results on semi-linear parabolic partial differential equations; and [a20] for a generalized Euler equation.

References

[a1]	C. Bardos, G. Lebeau, J. Rauch, "Scattering frequencies and Gevrey singularities" Invent. Math. , 90 (1987) pp. 77–114
[a2]	A. Bove, D. Tartakoff, "Optimal non-isotropic Gevrey exponents for sums of squares of vector fields" Commun. Partial Diff. Eq. , 22 (1997) pp. 1263–1282
[a3]	M.D. Bronstein, "The Cauchy problem for hyperbolic operators with characteristics of variable multiplicity" Trans. Moscow Math. Soc. , 1 (1982) pp. 87–103 (In Russian)
[a4]	F. Colombini, E. Janelli, S. Spagnolo, "Well-posedness in the Gevrey classes of the Cauchy problem for a nonstrictly hyperbolic equation with coeficients depending on time" Ann. Scuola Norm. Sup. Pisa , 10 (1983) pp. 291–312
[a5]	L. Cattabriga, L. Rodino, L. Zanghirati, "Analytic-Gevrey hypoellipticity for a class of pseudodifferential operators with multiple characteristics" Commun. Partial Diff. Eq. , 15 (1990) pp. 81–96
[a6]	M. Cicognani, L. Zanghirati, "On a class of unsolvable operators" Ann. Scuola Norm. Sup. Pisa , 20 (1993) pp. 357–369
[a7]	M. Christ, "Intermediate Gevrey exponents occur" Commun. Partial Diff. Eq. , 22 (1997) pp. 359–379
[a8]	C. Foias, R. Temam, "Gevrey class regularity for the solutions of the Navier-Stokes equations" J. Funct. Anal. , 87 (1989) pp. 359–369
[a9]	A. Ferrari, E. Titi, "Gevrey regularity for nonlinear analytic parabolic equations" Commun. Partial Diff. Eq. , 23 (1998) pp. 1–16
[a10]	M. Gourdain, M. Mechab, "Problème de Goursat non-linéaire dans les espaces de Gevrey pour les équations de Kirchoff généralisées" J. Math. Pures Appl. , 75 (1996) pp. 569–593
[a11]	T. Gramchev, G. Popov, "Nekhoroshev type estimates for billiard ball maps" Ann. Inst. Fourier (Grenoble) , 45 : 3 (1995) pp. 859–895
[a12]	T. Gramchev, L. Rodino, "Gevrey solvability for semilinear partial differential equations with multiple characteritics" Boll. Un. Mat. Ital. Sez. B (8) , 2 : 1 (1999) pp. 65–120
[a13]	M. Gevrey, "Sur la nature analytique des solutions des équations aux dérivées partielles" Ann. Ecole Norm. Sup. Paris , 35 (1918) pp. 129–190
[a14]	H. Chen, L. Rodino, "General theory of PDE and Gevrey classes" , General theory of partial differential equations and microlocal analysis (Trieste, 1995) , Pitman Res. Notes Math. , 349 , Longman (1996) pp. 6–81
[a15]	V.Ya. Ivrii, "Correctness in Gevrey classes of the Cauchy problem for certain nonstrictly hyperbolic operators" Izv. Vyš. Učebn. Zaved. Mat. , 189 (1978) pp. 26–35 (In Russian)
[a16]	K. Kajitani, "Local solutions of Cauchy problem for nonlinear hyperbolic systems in Gevrey classes" Hokkaido Math. J. , 12 (1983) pp. 434–460
[a17]	H. Komatsu, "Ultradistributions I–III" J. Fac. Sci. Univ. Tokyo Sec. IA Math. , 19; 24; 29 (1973/77/82) pp. 25–105; 607–628; 653–717
[a18]	B. Lascar, R. Lascar, "Propagation des singularités Gevrey pour la diffraction" Commun. Partial Diff. Eq. , 16 (1991) pp. 547–584
[a19]	J. Leray, Y. Ohya, "Equations et systèmes non-linèaires, hyperboliques non-strictes" Math. Ann. , 170 (1967) pp. 167–205
[a20]	C.D. Levermore, M. Oliver, "Analyticity of solutions for a generalized Euler equation" J. Diff. Eq. , 133 (1997) pp. 321–339
[a21]	M. Mascarello, L. Rodino, "Partial differential equations with multiple characteristics" , Math. Topics , 13 , Akad. (1997)
[a22]	M. Miyake, M. Yoshino, "Fredholm property of partial differential opertors of irregular singular type" Ark. Mat. , 33 (1995) pp. 323–341
[a23]	T. Matsuzawa, "Gevrey hypoellipticity for Grushin operators" Publ. Res. Inst. Math. Sci. , 33 (1997) pp. 775–799
[a24]	S. Mizohata, "On the Cauchy problem" , Acad. Press &Sci. Press Beijing (1985)
[a25]	J.-P. Ramis, R. Schäfke, "Gevrey separation of fast and slow variables" Nonlinearity , 9 (1996) pp. 353–384
[a26]	J.-P. Ramis, "Séries divergentes et théorie asymptotiques" Bull. Sci. Math. France , 121 (1993) (Panoramas et Syntheses, suppl.)
[a27]	L. Rodino, "Linear partial differential operators in Gevrey spaces" , World Sci. (1993)