Pontryagin class

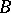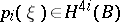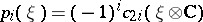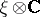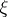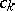A characteristic class defined for real vector bundles (cf. Vector bundle). Pontryagin classes were introduced by L.S. Pontryagin [1] in 1947. For a vector bundle with base the Pontryagin classes are denoted by the symbol and are defined to be equal to , where is the complexification of and are the Chern classes (cf. Chern class). The total Pontryagin class is the non-homogeneous characteristic class 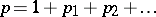. In other words, the Pontryagin classes are defined as homology classes determined by the equality , where is the mapping corresponding to the complexification of the universal vector bundle and 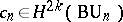are the Chern classes.

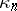Let be the real bundle of the universal vector bundle over 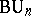. The total Pontryagin class 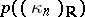of the vector bundle 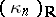coincides with 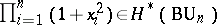, where are the Wu generators (see Characteristic class).

A partial description of the cohomology ring 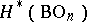can be obtained in terms of Wu generators in the following way. The mapping corresponding to the vector bundle 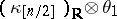, where 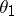is the one-dimensional trivial vector bundle, induces a ring homomorphism 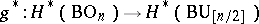, under which the subring of 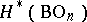generated by the Pontryagin classes 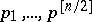is mapped monomorphically onto the subring of consisting of all even symmetric polynomials in the Wu generators. Evenness is understood in the sense that the degree of every variable 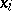in the polynomial should be even. Thus, an expression in Wu generators is obtained for any element of the ring 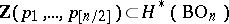. This is important for practical calculations with Pontryagin classes. The characteristic class determined by an even symmetric polynomial in the Wu generators can be expressed in Pontryagin classes as follows. First, the polynomial is written in elementary symmetric functions of the variables and then the elementary symmetric functions are replaced by Pontryagin classes.

If are two real vector bundles over a common base, then the cohomology class is of order at most two; this is due to the fact that for the first Chern class .

Let a ring 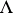, containing 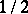, be considered as the ring of coefficients, and let 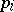be a Pontryagin class with values in 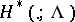. In this case the following equality is valid:

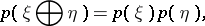or

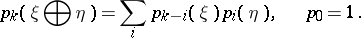The ring is monomorphically mapped into 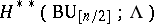and the image of this mapping coincides with the subring of all even symmetric series in Wu generators as variables. Then the total Pontryagin class is mapped to the polynomial 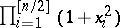, and the Pontryagin classes — to elementary symmetric functions of 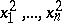. Theorem:

The cohomology ring contains, beside a Pontryagin class, also the Euler class 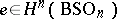. Theorem:

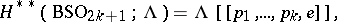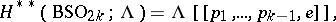for the space 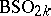the equality 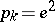holds.

The mapping 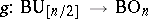can be extended to . The induced mapping maps to zero for odd, and to 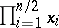for even.

Let 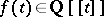be a formal power series over the field 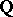. Then the series 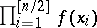determines some non-homogeneous element of the ring 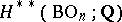, i.e. a characteristic class. Admitting a certain freedom, one can write

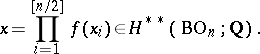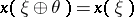The characteristic class is stable (that is , where 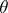is the trivial vector bundle) if and only if the constant term in 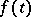is equal to one. If one assumes 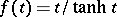, then the characteristic class constructed by the above-described method is denoted by 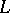and is called the Hirzebruch -class,

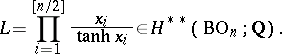The standard procedure of expressing the series 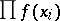in elementary symmetric functions of 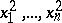leads to the representation of in the form of a series in Pontryagin classes. Another characteristic class that is important for applications is obtained if it is assumed that

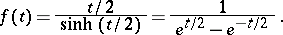The class determined by the even symmetric series

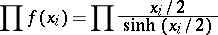is called the -class. Similarly, the -class is the characteristic class determined by the series 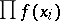where 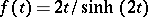. Both these classes, as well as 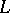, can be expressed in Pontryagin classes.

Topological invariance.

In 1965 S.P. Novikov [2] proved that the Pontryagin classes with rational coefficients coincide for two homeomorphic manifolds. It was already known that rational Pontryagin classes are piecewise-linearly invariant, i.e. coincide for two piecewise-linear homeomorphic manifolds. Moreover, rational Pontryagin classes were defined (see [4]) for piecewise-linear manifolds (possibly with a boundary). An example was given (see ) of integer Pontryagin classes which are not topological invariants.

In 1969 it was shown (see [7]) that the fibre 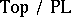of the bundle 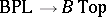has the homotopy type of the Eilenberg–MacLane space 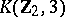. From this the topological invariance of rational Pontryagin classes follows, as well as a disproof of the fundamental hypothesis of combinatorial topology (the Hauptvermutung).

Generalized Pontryagin classes.

Let 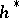be a generalized cohomology theory (cf. Generalized cohomology theories) in which Chern classes are defined. If for a one-dimensional complex vector bundle 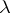the equality 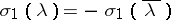holds, the Pontryagin classes with values in the theory can be defined via the above-mentioned formula 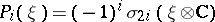. The classes thus defined will have the property 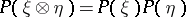, where is the total Pontryagin class considered in the theory .

However, in many generalized theories used in practice (for example, in -theory) the equality proposed for 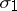does not hold. In such theories it does not make sense to define Pontryagin classes in the above-described manner, since under such a definition the usual formula for the total class of the sum of two vector bundles, even after including in the coefficients, is not valid. One can define generalized Pontryagin classes in the following way. 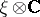Let be a multiplicative cohomology theory in which an orientation of a vector bundle , where 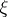is an arbitrary -dimensional real vector bundle over 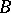, is universally given. 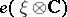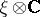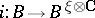Let be the Euler class of , , where is the inclusion of a zero section. Pontryagin classes in the theory 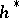are the characteristic classes , defined for real vector bundles and satisfying the following conditions:

1) 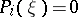if ;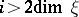

2) 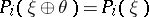, where 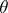is the trivial bundle;

3) 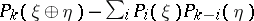is an element of order a power of two;

4) 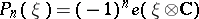, where .

The uniqueness and existence of characteristic classes with the above properties has been proved. From this point of view, Pontryagin classes lead to the notion of a two-valued formal group over the ring 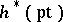corresponding to the theory .

The characteristic classes 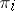in -theory are defined by the following formula:

where 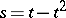; here 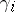are the Chern classes in -graded 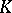-theory.

References

[1]	L.S. Pontryagin, "Characteristic classes of differentiable manifolds" Transl. Amer. Math. Soc. (1) , 7 (1962) pp. 279–331 Mat. Sb. , 21 (1947) pp. 233–284
[2]	S.P. Novikov, "Topological invariance of rational Pontrjagin classes" Soviet Math. Dokl. , 6 : 4 (1965) pp. 921–923 Dokl. Akad. Nauk SSSR , 163 (1965) pp. 298–300
[3]	V.M. Bukhshtaber, "The Chern–Dold character in cobordisms. I" Math. USSR Sb. , 12 : 4 (1970) pp. 573–594 Mat. Sb. , 83 (1970) pp. 575–595
[4]	V.A. Rokhlin, A.S. Shvarč, "The combinatorial invariance of Pontrjagin classes" Dokl. Akad. Nauk SSSR , 114 (1957) pp. 490–493 (In Russian)
[5a]	J. Milnor, Matematika , 3 : 4 (1959) pp. 3–53
[5b]	J. Milnor, Matematika , 9 : 4 (1965) pp. 3–40
[6]	R.E. Stong, "Notes on cobordism theory" , Princeton Univ. Press (1968)
[7]	R.C. Kirby, L.C. Siebenmann, "Foundational essays on topological manifolds, smoothings, and triangulations" , Princeton Univ. Press (1977)

References

[a1]	J.W. Milnor, J.D. Stasheff, "Characteristic classes" , Princeton Univ. Press (1974)
[a2]	D.W. Anderson, E.H. Brown, F.P. Peterson, "Spin cobordism" Ann. of Math. (To appear)
[a3]	J. Dieudonné, "A history of algebraic and differential topology 1900–1960" , Birkhäuser (1989)