Resolution

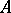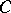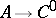In homological algebra a right resolution of a module is a complex (in homological algebra) : , defined for positive degrees and provided with a supplementary homomorphism such that the sequence 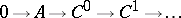is exact (cf. Exact sequence).

Comments

The supplementary homomorphism 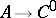can also be seen as a homomorphism of complexes , where is viewed as a complex concentrated in degree zero. The right resolution 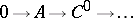is called injective if the modules are all injective (cf. Injective module). Dually, a left resolution is an exact sequence . Such a left resolution is called projective if all the modules 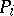are projective, free if all the 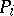are free, and flat if all the 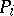are flat (cf. Projective module; Flat module).

More generally, the notion of a resolution of an object can be defined in any Abelian category in a completely similar way, [a1]. E.g., in the category of sheaves of Abelian groups on a topological space an injective resolution of a sheaf 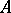is an exact sequence 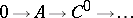of sheaves of Abelian groups with each 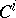an injective sheaf. In sheaf theory one often uses resolutions by flabby or soft sheaves (cf. Flabby sheaf; Soft sheaf). For the case of sheaves over a topos see [a5], [a6].

Resolutions are the main tool in the calculation of derived functors (cf. Derived functor) and in the approach to homology and cohomology as derived functors. In order to construct derived functors in a non-additive category, the technique of simplicial resolutions is used [a4].

In many cases it is useful to use resolutions of very special forms. One such is the resolution afforded by the Koszul complex, which is something like an exterior algebra pulled apart.

References

[a1]	A. Grothendieck, "Sur quelques points d'algèbre homologique" Tohôku Math. J. , 9 (1957) pp. 119–221
[a2]	S. Lang, "Algebra" , Addison-Wesley (1984)
[a3]	R. Hartshorne, "Algebraic geometry" , Springer (1977) pp. 13; 170; 316; 381
[a4]	M. André, "Méthode simpliciale en algèbre homologique et algèbre commutative" , Lect. notes in math. , 32 , Springer (1967)
[a5]	P. Berthelot, A. Ogus, "Notes on crystalline cohomology" , Princeton Univ. Press (1978)
[a6]	J.S. Milne, "Etale cohomology" , Princeton Univ. Press (1980)
[a7]	H. Cartan, S. Eilenberg, "Homological algebra" , Princeton Univ. Press (1956)
[a8]	S. MacLane, "Homology" , Springer (1963) pp. 16, 260
[a9]	R. Godement, "Théorie des faisceaux" , Hermann (1964)