Multiple point

of a planar curve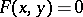

A singular point at which the partial derivatives of order up to and including vanish, but where at least one partial derivative of order 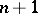does not vanish. For example, if 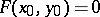, , 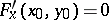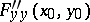does not vanish, the multiple point 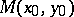is called a double point; if the first and second partial derivatives vanish at 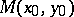, but at least one third derivative does not, the multiple point is called a triple point; etc.

References

[a1]	J.L. Coolidge, "Algebraic plane curves" , Dover, reprint (1959)
[a2]	D. Hilbert, S.E. Cohn-Vossen, "Geometry and the imagination" , Chelsea (1952) pp. 173 (Translated from German)
[a3]	P.A. Griffiths, J.E. Harris, "Principles of algebraic geometry" , Wiley (Interscience) (1978)
[a4]	W. Fulton, "Algebraic curves" , Benjamin (1969) pp. 66