Analytic space
A generalization of the concept of an analytic manifold. A local model (and, at the same time, the most important example) of an analytic space over a complete non-discretely normed field  is an analytic set
is an analytic set  in a domain
in a domain  of the
of the  -dimensional space
-dimensional space  over
over  , defined by equations
, defined by equations 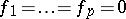 , where
, where  are analytic functions in
are analytic functions in  , which is provided with the sheaf
, which is provided with the sheaf 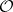 obtained by restricting the sheaf
obtained by restricting the sheaf 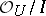 on
on 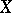 ; here
; here 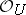 is the sheaf of germs of analytic functions in
is the sheaf of germs of analytic functions in 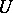 , while
, while  is the subsheaf of ideals generated by
is the subsheaf of ideals generated by  . An analytic space over
. An analytic space over 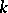 is a ringed space that is locally isomorphic to a ringed space
is a ringed space that is locally isomorphic to a ringed space  of the above type. If
of the above type. If 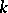 is the field of real numbers
is the field of real numbers 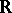 , one speaks of real-analytic spaces; if
, one speaks of real-analytic spaces; if 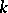 is the field of complex numbers
is the field of complex numbers  , of complex-analytic spaces or simply of complex spaces; if
, of complex-analytic spaces or simply of complex spaces; if 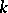 is a field of
is a field of  -adic numbers
-adic numbers 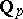 , of
, of  -adic analytic spaces.
-adic analytic spaces.
An analytic (holomorphic) mapping of one analytic space  into another
into another  is a morphism
is a morphism  in the sense of the theory of ringed spaces, i.e. a pair
in the sense of the theory of ringed spaces, i.e. a pair  , where
, where  is a continuous mapping, while
is a continuous mapping, while  is a sheaf homomorphism. A point
is a sheaf homomorphism. A point  of an analytic space
of an analytic space  is called simple (or regular, or non-singular) if
is called simple (or regular, or non-singular) if  has a neighbourhood over which
has a neighbourhood over which  is isomorphic to a space of the type
is isomorphic to a space of the type 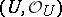 , where
, where 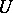 is a domain in
is a domain in  . Otherwise
. Otherwise  is known as a singular point. A space is called smooth if all of its points are simple. A smooth analytic space is identical with an analytic manifold.
is known as a singular point. A space is called smooth if all of its points are simple. A smooth analytic space is identical with an analytic manifold.
The dimension  of an analytic space
of an analytic space 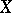 at a point
at a point 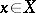 is defined as the dimension of the corresponding analytic set in a local model (cf. Analytic set). The global dimension is defined by the formula:
is defined as the dimension of the corresponding analytic set in a local model (cf. Analytic set). The global dimension is defined by the formula:
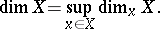 |
Let  be the maximal ideal of the local ring
be the maximal ideal of the local ring 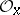 (
( ). The vector space
). The vector space  over
over 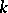 is called the tangent space to
is called the tangent space to  at the point
at the point  , while
, while  is the cotangent space. The number
is the cotangent space. The number
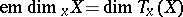 |
is called the tangent dimension or the embedding dimension at the point  (the last name is connected with the fact that
(the last name is connected with the fact that  is the smallest number
is the smallest number  such that
such that  is, in a neighbourhood of
is, in a neighbourhood of  , isomorphic to a local model in
, isomorphic to a local model in 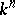 ). One has
). One has  , and the two are equal if and only if
, and the two are equal if and only if  is a simple point. One also defines the dimension
is a simple point. One also defines the dimension
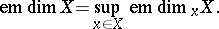 |
Each analytic mapping of analytic spaces  defines a linear mapping
defines a linear mapping 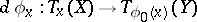 , which is called its differential at the point
, which is called its differential at the point  .
.
An analytic space 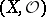 is said to be reduced if its local model in a neighbourhood of an arbitrary point has the following property:
is said to be reduced if its local model in a neighbourhood of an arbitrary point has the following property: 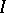 consists of all germs of holomorphic functions that vanish on
consists of all germs of holomorphic functions that vanish on  . In the case of an algebraically closed field
. In the case of an algebraically closed field  , this statement is equivalent to saying that the fibres
, this statement is equivalent to saying that the fibres 
 of the sheaf
of the sheaf  contain no nilpotent elements. All smooth spaces are reduced. If
contain no nilpotent elements. All smooth spaces are reduced. If  is reduced, it can be said that
is reduced, it can be said that  consists of the germs of certain continuous functions on
consists of the germs of certain continuous functions on 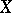 . The sections of the sheaf
. The sections of the sheaf  on the reduced space
on the reduced space 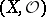 are identical with analytic functions on
are identical with analytic functions on  , i.e. with analytic mappings
, i.e. with analytic mappings  (cf. Analytic mapping). For any analytic space
(cf. Analytic mapping). For any analytic space  there exists a natural sheaf epimorphism
there exists a natural sheaf epimorphism 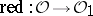 (where
(where 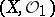 is a reduced analytic space), which is called the reduction. If
is a reduced analytic space), which is called the reduction. If  is a section of the sheaf
is a section of the sheaf  , one can speak of the value of
, one can speak of the value of  at a point
at a point  (which coincides with the value of the analytic function
(which coincides with the value of the analytic function 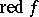 at
at  ). For this reason the algebra
). For this reason the algebra 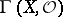 , even in the non-reduced case, is often referred to as the algebra of analytic (holomorphic) functions on
, even in the non-reduced case, is often referred to as the algebra of analytic (holomorphic) functions on  . Sheaves of
. Sheaves of 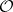 -modules on an analytic space
-modules on an analytic space  are also called analytic sheaves.
are also called analytic sheaves.
If  is an analytic space, then each open
is an analytic space, then each open  defines an open subspace
defines an open subspace  . On the other hand, one can introduce the concept of an analytic subspace of
. On the other hand, one can introduce the concept of an analytic subspace of  , which is necessarily closed. A set
, which is necessarily closed. A set  is called analytic if it is defined by a finite number of analytic equations in a neighbourhood of each point
is called analytic if it is defined by a finite number of analytic equations in a neighbourhood of each point 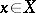 . The sheaf of ideals
. The sheaf of ideals  consisting of the germs of all analytic functions that vanish on
consisting of the germs of all analytic functions that vanish on  , is connected with such a set. Conversely, each analytic sheaf of ideals of finite type
, is connected with such a set. Conversely, each analytic sheaf of ideals of finite type  defines an analytic set
defines an analytic set 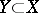 . If
. If 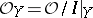 , one obtains an analytic space
, one obtains an analytic space 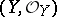 , which is called an analytic subspace of
, which is called an analytic subspace of  ; there exists a natural morphism
; there exists a natural morphism 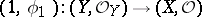 . An example of an analytic subspace of
. An example of an analytic subspace of  is the reduction of this space.
is the reduction of this space.
The concept of an analytic space originated as a generalization of the concept of an analytic manifold. Such a generalization had been suggested mainly by algebraic geometry, in which spaces with singular points had been under study for a long time. The effect of the ideas of algebraic geometry was immediately reflected in the ultimate formulation of the concept of an analytic space (for reduced complex spaces see [9]; for the general case, see ). In particular, any scheme of finite type over a complete normed field 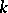 naturally determines an analytic space over
naturally determines an analytic space over  . This correspondence between schemes and analytic spaces over
. This correspondence between schemes and analytic spaces over 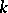 for reduced complex spaces was studied in [9], in which the theory of analytic spaces was named "analytic geometryanalytic geometry" . Subsequently, the two geometries developed in parallel, and the exchange of ideas between the two made a substantial contribution to the results achieved in both these fields.
for reduced complex spaces was studied in [9], in which the theory of analytic spaces was named "analytic geometryanalytic geometry" . Subsequently, the two geometries developed in parallel, and the exchange of ideas between the two made a substantial contribution to the results achieved in both these fields.
In the theory of functions of several complex variables, spaces with singular points appeared, in the first place, as Riemannian domains (cf. Riemannian domain), which are analogues of Riemann surfaces for functions of one variable. Using these as local models, H. Behnke and K. Stein in 1951 defined a class of ringed spaces which, as was shown in [5], coincides with the class of reduced normal analytic spaces (cf. Normal analytic space). The local geometry of analytic sets in 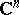 had been studied by W. Rückert as early as 1932. Finally, non-smooth analytic spaces are a natural product of the theory of automorphic functions as quotient spaces of analytic manifolds by properly discrete groups of automorphisms (cf. Discrete group of transformations).
had been studied by W. Rückert as early as 1932. Finally, non-smooth analytic spaces are a natural product of the theory of automorphic functions as quotient spaces of analytic manifolds by properly discrete groups of automorphisms (cf. Discrete group of transformations).  -adic analytic sets were first introduced in 1935 by I. Skolem in connection with certain problems in number theory.
-adic analytic sets were first introduced in 1935 by I. Skolem in connection with certain problems in number theory.
The theory of analytic spaces has two aspects: the local and the global aspect. Local analytic geometry is concerned with germs of analytic sets in the space 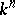 provided with sheaves of the above type. Principal stress is laid on the study of the properties of the algebra of convergent power series in
provided with sheaves of the above type. Principal stress is laid on the study of the properties of the algebra of convergent power series in  variables over
variables over  and its quotient algebras — the so-called analytic algebras, the foundations of which were laid by K. Weierstrass. The local theory comprises the theory of normalization, the study of singular points, local properties of analytic functions and mappings, etc. The most important results obtained in this field refer to the case of algebraically closed fields
and its quotient algebras — the so-called analytic algebras, the foundations of which were laid by K. Weierstrass. The local theory comprises the theory of normalization, the study of singular points, local properties of analytic functions and mappings, etc. The most important results obtained in this field refer to the case of algebraically closed fields  [1], [4], [7]. There appears the important concept of a coherent analytic sheaf, which continues to play a leading part in the global theory. In particular, the structure sheaf
[1], [4], [7]. There appears the important concept of a coherent analytic sheaf, which continues to play a leading part in the global theory. In particular, the structure sheaf  of the analytic space
of the analytic space  and the sheaf of ideals
and the sheaf of ideals 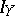 of any analytic set
of any analytic set 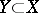 are coherent for any algebraically closed
are coherent for any algebraically closed 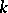 . The case
. The case 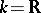 has also been thoroughly studied.
has also been thoroughly studied.
Global analytic geometry studies the properties of analytic functions, mappings and other analytic objects, defined "globally" on the entire analytic space, as well as the geometrical properties of these spaces. In the process of studying complex-analytic spaces natural classes of them were isolated. These include, first, the class of Stein spaces (cf. Stein space), which can be roughly described as the class of spaces with a sufficiently large amount of global holomorphic functions. Stein spaces are the most natural multi-dimensional generalizations of the domains of the complex plane considered in the classical theory of functions of one complex variable. This class of spaces in fact coincides with the class of analytic subspaces of the spaces  . Its algebraic analogue is the class of affine algebraic varieties (cf. Affine variety).
. Its algebraic analogue is the class of affine algebraic varieties (cf. Affine variety).
For a domain 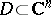 holomorphic completeness is equivalent with the fact that
holomorphic completeness is equivalent with the fact that  is a domain of holomorphy, i.e. that there exists a holomorphic function in
is a domain of holomorphy, i.e. that there exists a holomorphic function in 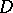 that does not extend into a larger domain. The boundary of a domain of holomorphy is pseudo-convex, i.e. it behaves with respect to local analytic submanifolds as would a convex surface with respect to real linear submanifolds. The problem of the validity of the converse theorem (cf. Levi problem) gave rise to a number of investigations and yielded a new characterization of Stein spaces.
that does not extend into a larger domain. The boundary of a domain of holomorphy is pseudo-convex, i.e. it behaves with respect to local analytic submanifolds as would a convex surface with respect to real linear submanifolds. The problem of the validity of the converse theorem (cf. Levi problem) gave rise to a number of investigations and yielded a new characterization of Stein spaces.
The class of compact spaces is, in a certain sense, the opposite case. The following generalization of the classical theorem of Liouville is valid: Functions which are holomorphic on a reduced compact space are constant on each connected component of this space and therefore form a finite-dimensional vector space. A generalization of this theorem are the finiteness theorems, which confirm the finite dimensionality of the homology groups with values in a coherent analytic sheaf. Holomorphically-convex complex spaces, 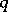 -complete,
-complete,  -pseudo-convex,
-pseudo-convex,  -pseudo-concave spaces, which are generalizations of Stein spaces, and compact spaces are also considered (cf. Holomorphically-convex complex space).
-pseudo-concave spaces, which are generalizations of Stein spaces, and compact spaces are also considered (cf. Holomorphically-convex complex space).
These classes of complex spaces have their analogues in the theory of holomorphic mappings. Thus, to compact spaces correspond proper holomorphic mappings; to holomorphically complete spaces correspond Stein mappings, etc. "Relative" analogues were found for many theorems, and the "absolute" variant of a theorem is obtained from its relative variant if the entire space is mapped into a point. The corresponding generalization of finiteness theorems are theorems of coherence of direct images of coherent analytic sheaves under holomorphic mappings, the first and most important one of which (for proper mappings) was demonstrated by H. Grauert [6a].
An important role in the theory of complex spaces is played by holomorphic mappings of a special kind — the so-called modifications (cf. Modification), i.e. mappings 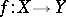 inducing an isomorphism of open subspaces
inducing an isomorphism of open subspaces 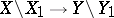 , where
, where 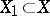 ,
, 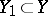 are certain analytic sets. One says that
are certain analytic sets. One says that 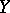 is obtained from
is obtained from 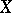 by "contracting" the subset
by "contracting" the subset 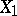 on
on  , while
, while  is obtained from
is obtained from  by "blowing up" the subset
by "blowing up" the subset 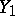 into
into 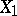 . Of special interest are analytic subsets that can be contracted into a point (exceptional analytic sets); these were characterized by H. Grauert [6b]. A natural problem in analytic geometry is the following problem of resolution of singularities: Is it possible to "blow up" an analytic space so that the entire space becomes smooth? It should be noted that modifications in algebraic geometry were studied as early as the 19th century, while modifications in analytic geometry were introduced by Behnke and Stein in 1951 in the context of the concept of a Riemannian domain.
. Of special interest are analytic subsets that can be contracted into a point (exceptional analytic sets); these were characterized by H. Grauert [6b]. A natural problem in analytic geometry is the following problem of resolution of singularities: Is it possible to "blow up" an analytic space so that the entire space becomes smooth? It should be noted that modifications in algebraic geometry were studied as early as the 19th century, while modifications in analytic geometry were introduced by Behnke and Stein in 1951 in the context of the concept of a Riemannian domain.
Another natural object of study, which is also closely connected with the ideas of algebraic geometry, are meromorphic functions on complex spaces and their generalizations — meromorphic mappings (a mapping which yields an operation inverse to a modification may serve as an example; cf. Meromorphic function; Meromorphic mapping). Meromorphic functions on a reduced compact complex space  form a field of transcendence degree
form a field of transcendence degree  (this was first demonstrated by C.L. Siegel in 1955 for the smooth case). Spaces
(this was first demonstrated by C.L. Siegel in 1955 for the smooth case). Spaces  for which
for which  (Moishezon spaces) form a class which is very close to the class of projective algebraic varieties; they are characterized by the fact that they are modifications of smooth projective algebraic varieties. Another class of analytic spaces which is very close to algebraic varieties, are Kähler manifolds (cf. Kähler manifold). A number of criteria for the projectivity of a compact complex space are known [3], [6b], [13]. Studies of automorphic functions in several complex variables have made a major contribution to the development of this subject.
(Moishezon spaces) form a class which is very close to the class of projective algebraic varieties; they are characterized by the fact that they are modifications of smooth projective algebraic varieties. Another class of analytic spaces which is very close to algebraic varieties, are Kähler manifolds (cf. Kähler manifold). A number of criteria for the projectivity of a compact complex space are known [3], [6b], [13]. Studies of automorphic functions in several complex variables have made a major contribution to the development of this subject.
The theory of deformations of analytic structures (cf. Deformation) is concerned with the problem of classification of analytic objects of a given type (e.g. all complex structures on a given real-analytic variety, all analytic subspaces in a given complex space, etc.), with the purpose of introducing the "natural" structure of a complex space on the set of these objects, and in order to describe all analytic objects "sufficiently near" to the given object. In the former case one speaks of the global moduli problem, while in the latter one speaks of the local moduli problem. An example of the global moduli problem is the problem of the classification of all complex structures on a compact Riemann surface (cf. Moduli of a Riemann surface).
The principal apparatus of global analytic geometry is formed by coherent analytic sheaves and their cohomology spaces. The first successful result of the cohomological method was Cartan's solution of the additive Cousin problem and of problems of prolongation of a holomorphic function from a closed 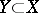 for Stein manifolds
for Stein manifolds 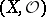 (cf. Cousin problems; [8]); it was found that the solution of these problems is obstructed by the cohomology groups
(cf. Cousin problems; [8]); it was found that the solution of these problems is obstructed by the cohomology groups 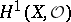 and
and 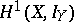 , respectively.
, respectively.
Most of the results of the global theory were initially demonstrated for complex manifolds, after which they were generalized to analytic spaces. The difficulties involved in this procedure often necessitated the development of completely new methods. Cohomology spaces of a locally free analytic sheaf on a complex manifold may be expressed in terms of differential forms (the Dolbeault–Serre theorem, cf. also Differential form), which makes it possible to study them by methods of the theory of elliptic differential equations and by other analytic methods. In the non-smooth case this approach involves major difficulties, and it is often necessary to define cohomology classes in other ways, e.g. using Čech cochains in a suitable covering. The technique of Banach analytic spaces, applied to moduli problems, proved useful in this context (cf. Banach analytic space).
See also Real-analytic space; Rigid analytic space.
References
| [1] | S.S. Abhyankar, "Local analytic geometry" , Acad. Press (1964) |
| [2] | C. Banica, O. Stanasila, "Algebraic methods in the global theory of complex spaces" , Wiley (1976) (Translated from Rumanian) |
| [3] | R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) |
| [4] | H. Grauert, R. Remmert, "Analytische Stellenalgebren" , Springer (1971) |
| [5] | H. Grauert, R. Remmert, "Komplexe Räume" Math. Ann. , 136 (1958) pp. 245–318 |
| [6a] | H. Grauert, "Ein Theorem der analytischen Garbentheorie und die Modulräume komplexer Strukturen" Publ. Math. IHES , 5 (1960) pp. 233–292 |
| [6b] | H. Grauert, "Ueber Modifikationen und exzeptionelle analytische Mengen" Math. Ann. , 146 : 4 (1962) pp. 331–368 |
| [7] | R. Narasimhan, "Introduction to the theory of analytic spaces" , Lect. notes in math. , 25 , Springer (1966) |
| [8] | H. Cartan, "Variétés analytiques complexes et cohomologie" , Colloque sur les fonctions de plusieurs variables, Brussels 1953 , G. Thone & Masson (1953) |
| [9] | J.-P. Serre, "Géométrie algébrique et géométrie analytique" Ann. Inst. Fourier (Grenoble) , 6 (1955–1956) pp. 1–42 |
| [10] | B.A. Fuks, "Special chapters in the theory of analytic functions of several complex variables" , Amer. Math. Soc. (1965) (Translated from Russian) |
| [11] | L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973) |
| [12] | F. Hirzebruch, "Topological methods in algebraic geometry" , Springer (1978) (Translated from German) |
| [13] | S.S. Chern, "Complex manifolds without potential theory" , Springer (1979) |
Comments
Instead of [8] one may also consult [a2].
The original papers in which what are now called Moishezon spaces were introduced are [a3]. They are a natural setting to examine the question of projectivity of compact complex spaces. In particular, part I contains the result that a Moishezon space is algebraic projective if and only if it carries a Kähler metric.
References
| [a1] | H. Grauert, R. Remmert, "Coherent analytic sheaves" , Springer (1984) (Translated from German) |
| [a2] | H. Cartan, "Sem. E.N.S. 1951–1952" , Ecole Norm. Sup. |
| [a3] | B. Moishezon, "On  -dimensional compact complex varieties with -dimensional compact complex varieties with  algebraically independent meromorphic functions" Amer. Math. Soc. Translations Ser. 2 , 63 (1967) pp. 51–177 algebraically independent meromorphic functions" Amer. Math. Soc. Translations Ser. 2 , 63 (1967) pp. 51–177 |
Analytic space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Analytic_space&oldid=23754