Markov chain, decomposable
From Encyclopedia of Mathematics
Revision as of 20:16, 9 March 2012 by Boris Tsirelson (talk | contribs) (MSC|60J10|60J27 Category:Markov processes)
2020 Mathematics Subject Classification: Primary: 60J10 Secondary: 60J27 [MSN][ZBL]
A Markov chain whose transition probabilities 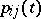 have the following property: There are states
have the following property: There are states  such that
such that 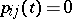 for all
for all 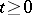 . Decomposability of a Markov chain is equivalent to decomposability of its matrix of transition probabilities
. Decomposability of a Markov chain is equivalent to decomposability of its matrix of transition probabilities 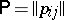 for discrete-time Markov chains, and of its matrix of transition probability densities
for discrete-time Markov chains, and of its matrix of transition probability densities 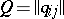 ,
, 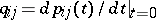 , for continuous-time Markov chains. The state space of a decomposable Markov chain consists either of inessential states or of more than one class of communicating states (cf. Markov chain).
, for continuous-time Markov chains. The state space of a decomposable Markov chain consists either of inessential states or of more than one class of communicating states (cf. Markov chain).
Comments
References
| [a1] | W. Feller, "An introduction to probability theory and its applications" , 1–2 , Wiley (1966) |
| [a2] | D. Freedman, "Markov chains" , Holden-Day (1975) |
| [a3] | M. Iosifescu, "Finite Markov processes and their applications" , Wiley (1980) |
| [a4] | J.G. Kemeny, J.L. Snell, "Finite Markov chains" , v. Nostrand (1960) |
| [a5] | J.G. Kemeny, J.L. Snell, A.W. Knapp, "Denumerable Markov chains" , Springer (1976) |
| [a6] | D. Revuz, "Markov chains" , North-Holland (1975) |
| [a7] | V.I. [V.I. Romanovskii] Romanovsky, "Discrete Markov chains" , Wolters-Noordhoff (1970) (Translated from Russian) |
| [a8] | E. Seneta, "Non-negative matrices and Markov chains" , Springer (1981) |
How to Cite This Entry:
Markov chain, decomposable. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_chain,_decomposable&oldid=23623
Markov chain, decomposable. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_chain,_decomposable&oldid=23623
This article was adapted from an original article by B.A. Sevast'yanov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article