Pseudo-Euclidean space

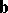A real affine space in which to any vectors and there corresponds a definite number, called the scalar product 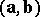(cf. also Inner product), satisfying

1) the scalar product is commutative:

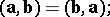2) the scalar product is distributive with respect to vector addition:

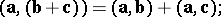3) a scalar factor can be taken out of the scalar product:

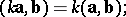4) there exist vectors 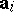such that

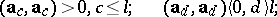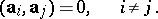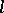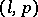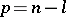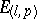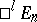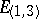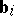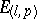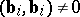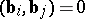The number is called the dimension of the pseudo-Euclidean space, is called the index, the pair of numbers , , is called the signature. A pseudo-Euclidean space is denoted by (or ). The space is called the Minkowski space. In any system of vectors in for which and when 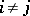, the number of vectors 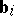for which 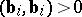is equal to and the number of vectors 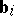for which 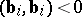is equal to 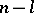(the law of inertia for a quadratic form).

The modulus 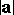of a vector in a pseudo-Euclidean space can be defined as the non-negative root 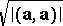. The vectors that have scalar squares equal to 1 or 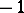are called unit and pseudo-unit vectors, respectively. The vectors for which 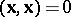have zero modulus and are called isotropic vectors. The directions of the isotropic vectors are the isotropic directions.

In a pseudo-Euclidean space there are three types of straight lines: Euclidean, having direction vector with positive scalar square 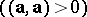, pseudo-Euclidean 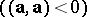and isotropic 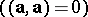. The union of all the isotropic straight lines passing through a certain point is called the isotropic cone.

In a pseudo-Euclidean space there are several types of planes: Euclidean planes 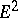, pseudo-Euclidean planes 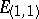and planes containing isotropic vectors, the so-called semi-Euclidean planes with signatures and 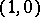and deficiency 1 (see Semi-Euclidean space) and isotropic planes, all vectors of which are isotropic.

The distance between two points 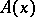and 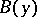is taken to be the modulus of the vector 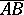and is computed from:

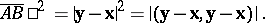A pseudo-Euclidean space is not a metric space, since the triangle inequality is not satisfied. If the vectors and 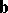belong to a Euclidean plane (or to a pseudo-Euclidean plane of index 0), then they satisfy the triangle inequality, but if they belong to a pseudo-Euclidean plane of index 1, then they satisfy the so-called inverse triangle inequality:

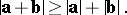In a pseudo-Euclidean space there are three types of spheres: spheres with positive radius squared, 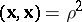, spheres with negative radius squared, 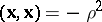, and spheres of zero radius, 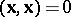, which are just the isotropic cones.

The motions of a pseudo-Euclidean space are affine transformations (cf. Affine transformation) and can be written in the form

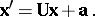The operator 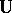satisfies the condition , that is, it preserves distances between points. The motions of a pseudo-Euclidean space form a multiplicative group; it depends on independent parameters. The motions of a pseudo-Euclidean space are called motions of the first or second kind if they are affine transformations of the corresponding kind.

Geometric transformations are called anti-motions when each vector goes to a vector 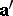for which .

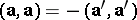The basic operations of vector and tensor algebra can be introduced into a pseudo-Euclidean space. The basic differential-geometric concepts are constructed in accordance with the rules of the geometry of pseudo-Riemannian space. The metric tensor of a pseudo-Euclidean space has the form (in a Galilean coordinate system)

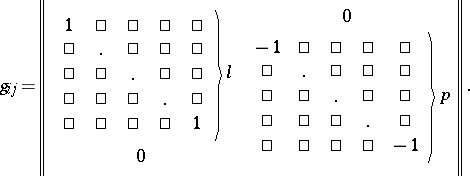A pseudo-Euclidean space is flat, that is, its Riemann tensor is zero. If the Riemann tensor of a pseudo-Riemannian space is identically zero, then it is a locally pseudo-Euclidean space.

Subsets of a pseudo-Euclidean space can carry various metrics: A positive- or negative-definite Riemannian metric, a pseudo-Riemannian metric or a degenerate metric (see Indefinite metric). For example, the spheres of a pseudo-Euclidean space carry a (generally speaking, indefinite) metric of constant curvature. In 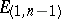a sphere with positive radius squared is an 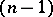-dimensional space isometric to the hyperbolic space.

The pseudo-Euclidean space 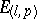(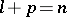) and the Euclidean space 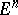can be considered as subspaces of a complex space with form 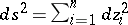. If 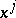are coordinates in the pseudo-Euclidean space, 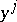are those of the real Euclidean space and 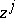those of the complex Euclidean space, then the equations of the subspaces have the form

The metric of the pseudo-Euclidean space can be formally obtained from the metric of the Euclidean space by the substitution , .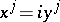

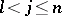References

[1]	N.V. Efimov, E.R. Rozendorn, "Linear algebra and multi-dimensional geometry" , Moscow (1970) (In Russian)
[2]	B.A. Rozenfel'd, "Multi-dimensional spaces" , Moscow (1966) (In Russian)
[3]	L.D. Landau, E.M. Lifshitz, "The classical theory of fields" , Addison-Wesley (1962) (Translated from Russian)

Comments

The concept of a pseudo-Euclidean space was generalized by E. Witt in 1937, see [a1]–[a2].

References

[a1]	E. Witt, "Theorie der quadratischen Formen in beliebigen Körpern" J. Reine Angew. Math. , 176 (1937) pp. 31–44
[a2]	J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1955)
[a3]	S.W. Hawking, G.F.R. Ellis, "The large scale structure of space-time" , Cambridge Univ. Press (1973)
[a4]	C.W. Misner, K.S. Thorne, J.A. Wheeler, "Gravitation" , Freeman (1973)
[a5]	B. O'Neill, "Semi-Riemannian geometry (with applications to relativity)" , Acad. Press (1983)