Stochastic process with independent increments

2020 Mathematics Subject Classification: Primary: 60G51 [MSN][ZBL]

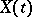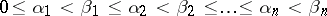A stochastic process such that for any natural number and all real , the increments

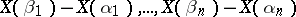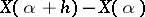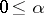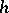are mutually-independent random variables. A stochastic process with independent increments is called homogeneous if the probability distribution of , , , depends only on and not on . Since the result of adding any non-random function to 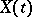is again a stochastic process with independent increments, the realizations of such processes can be arbitrarily irregular. However, by suitably "centering" the process (say by subtracting from 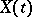the function 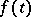defined by the relation 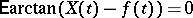), one can make more definite judgements about the structure of the "centred" process. There are at most countably-many (non-random) points 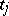at which 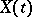has random jumps

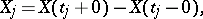and the difference

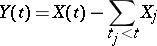is a stochastically-continuous stochastic process with independent increments: for any 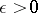and ,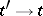

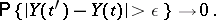A Wiener process and a Poisson process are examples of stochastically-continuous stochastic processes with independent increments (and realizations of the first are continuous with probability one, while realizations of the second are step functions with jumps equal to one). An important example of a stochastic process with independent increments is that of a stable process (cf. Stable distribution). Realizations of a stochastically-continuous stochastic process with independent increments can only have discontinuities of the first kind, with probability one. The distribution of the values of such a process for any 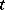is infinitely divisible (see Infinitely-divisible distribution). In studying stochastic processes with independent increments one can apply the method of characteristic functions (cf. Characteristic function). Problems on the probability of a process crossing a boundary and on the probability distribution of the first crossing time are solved using the so-called factorization identities.

References

[1]	I.I. [I.I. Gikhman] Gihman, A.V. [A.V. Skorokhod] Skorohod, "The theory of stochastic processes" , 2 , Springer (1975) (Translated from Russian) MR0375463 Zbl 0305.60027
[2]	A.V. [A.V. Skorokhod] Skorohod, "Random processes with independent increments" , Kluwer (1991) (Translated from Russian) MR1155400

Comments

For additional references see Stochastic process.