Prüfer domain

A commutative semi-hereditary integral domain (cf. also Semi-hereditary ring; Injective module). The Prüfer domains are the commutative integral domains that satisfy the following two conditions:

i) the localization (cf. also Localization in a commutative algebra) is a valuation ring (see Valuation) for every maximal ideal 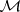of ;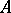

ii) an 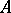-module 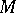is flat (cf. Flat module) if and only if it is torsion-free (cf. also Group without torsion).

A Noetherian Prüfer domain is a Dedekind domain (see Dedekind ring). Each Bezout domain (also called Bezout ring) is a Prüfer domain.

There are Prüfer domains that are not Bezout and there are Prüfer domains having finitely generated ideals requiring more than two generators [a2] (hence, these are not Dedekind rings).

References

[a1]	R. Gilmer, "Multiplicative ideal theory" , M. Dekker (1972)
[a2]	R. Heidman, L. Levy, " and -generator ideals in Prüfer domains" Rocky Mount. Math. J. , 5 (1975) pp. 361–373
[a3]	H.C. Hutchins, "Examples of commutative rings" , Polygonal (1981)