Markov chain, recurrent
2020 Mathematics Subject Classification: Primary: 60J10 [MSN][ZBL]
A Markov chain in which a random trajectory 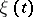 , starting at any state
, starting at any state 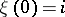 , returns to that state with probability 1. In terms of the transition probabilities
, returns to that state with probability 1. In terms of the transition probabilities 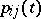 , recurrence of a discrete-time Markov chain is equivalent to the divergence for any
, recurrence of a discrete-time Markov chain is equivalent to the divergence for any 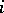 of the series
of the series
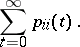 |
In a recurrent Markov chain a trajectory 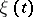 ,
, 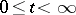 ,
, 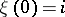 , returns infinitely often to the state
, returns infinitely often to the state 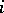 with probability 1. In a recurrent Markov chain there are no inessential states and the essential states decompose into recurrent classes. An example of a recurrent Markov chain is the symmetric random walk on the integer lattice on the line or plane. In the symmetric walk on the line a particle moves from position
with probability 1. In a recurrent Markov chain there are no inessential states and the essential states decompose into recurrent classes. An example of a recurrent Markov chain is the symmetric random walk on the integer lattice on the line or plane. In the symmetric walk on the line a particle moves from position  to
to  with probabilities
with probabilities 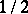 ; in the symmetric walk on the plane a particle moves from
; in the symmetric walk on the plane a particle moves from 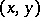 to one of the four points
to one of the four points 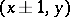 ,
, 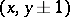 with probabilities
with probabilities 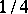 . In these examples a particle, starting the walk at an arbitrary point, returns to that point with probability 1. The symmetric walk on the integer lattice in the three-dimensional space, when the probability of transition from
. In these examples a particle, starting the walk at an arbitrary point, returns to that point with probability 1. The symmetric walk on the integer lattice in the three-dimensional space, when the probability of transition from  to a neighbouring point
to a neighbouring point 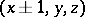 ,
, 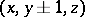 ,
, 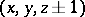 is equal to
is equal to 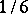 , is not recurrent. In this case the probability of return of the particle to its initial point is approximately 0.35.
, is not recurrent. In this case the probability of return of the particle to its initial point is approximately 0.35.
References
| [1] | W. Feller, "An introduction to probability theory and its applications" , 1 , Wiley (1966) MR0210154 Zbl 0138.10207 |
Comments
References
| [a1] | D. Freeman, "Markov chains" , Holden-Day (1975) |
| [a2] | M. Iosifescu, "Finite Markov processes and their applications" , Wiley (1980) MR0587116 Zbl 0436.60001 |
| [a3] | J.G. Kemeny, J.L. Snell, "Finite Markov chains" , v. Nostrand (1960) MR1531032 MR0115196 Zbl 0089.13704 |
| [a4] | J.G. Kemeny, J.L. Snell, A.W. Knapp, "Denumerable Markov chains" , Springer (1976) MR0407981 Zbl 0348.60090 |
| [a5] | D. Revuz, "Markov chains" , North-Holland (1975) MR0415773 Zbl 0332.60045 |
| [a6] | V.I. [V.I. Romanovskii] Romanovsky, "Discrete Markov chains" , Wolters-Noordhoff (1970) (Translated from Russian) MR0266312 Zbl 0201.20002 |
| [a7] | E. Seneta, "Non-negative matrices and Markov chains" , Springer (1981) MR2209438 Zbl 0471.60001 |
| [a8] | V. Spitzer, "Principles of random walk" , v. Nostrand (1964) MR0171290 Zbl 0119.34304 |
Markov chain, recurrent. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_chain,_recurrent&oldid=21655