Character of a semi-group
semi-group character
A non-zero homomorphism of a commutative semi-group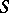 
with identity into the multiplicative semi-group consisting of all complex numbers of modulus 
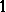, together with 0. Sometimes a character of a semi-group is understood as a non-zero homomorphism into the multiplicative semi-group of complex numbers of modulus 
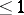. Both concepts of a character of a semi-group are equivalent if 
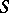is a Clifford semi-group. The set 
of all characters of a semi-group 
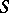forms a commutative semi-group with identity (the character semi-group) under pointwise multiplication 
,
 |
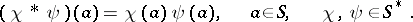An ideal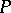 
of a semi-group 
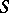is called totally isolated (prime) if 
is a sub-semi-group. The set of all totally-isolated ideals of a commutative semi-group with identity forms a semi-lattice under the operation of union. This semi-lattice is isomorphic to the semi-lattice of idempotents (see Idempotents, semi-group of) of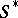 
. The characters of a commutative semi-group 
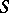separate the elements of 
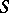if for any 
, 
, there is a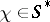 
such that 
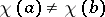. If 
has an identity, then the characters of the semi-group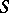 
separate the elements of 
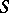if and only if 
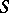is a separable semi-group. The problem of describing the character semi-group of an arbitrary commutative semi-group with identity reduces to a description of the characters of a semi-group that is a semi-lattice of groups; for a corresponding description when this semi-lattice satisfies a minimum condition see, for example, [1]. An abstract characterization of character semi-groups is in [2].
For every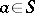 
, 
, the mapping 
, 
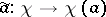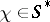, is a character of the semi-group 
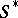, that is, 
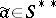. The mapping 
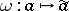is a homomorphism of 
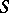into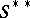 
(the so-called canonical homomorphism). If 
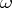is an isomorphism of 
onto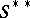 
, then one says that the duality theorem holds for 
. The duality theorem is true for a commutative semi-group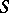 
with identity if and only if 
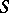is an inverse semi-group [3]. About duality problems for character semi-groups in the topological case see Topological semi-group.
References
| [1] | A.H. Clifford, G.B. Preston, "Algebraic theory of semi-groups" , 1 , Amer. Math. Soc. (1961) |
| [2] | M.M. Lesokhin, "Characters of commutative semigroups I" Izv. Vuz. Mat. , 8 (1970) pp. 67–74 (In Russian) |
| [3] | C. Austin, "Duality theorems for some commutative semigroups" Trans. Amer. Math. Soc. , 109 : 2 (1963) pp. 245–256 |
Character of a semi-group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Character_of_a_semi-group&oldid=18046