Ekeland variational principle
There are usually three ways for getting existence results in analysis, namely compactness, Hahn–Banach-type results and completeness properties (cf. Compactness; Hahn–Banach theorem; Completeness (in topology)). The Ekeland variational principle [a10] (which provides a characterization of complete metric spaces [a14], cf. also Complete metric space) illustrates the third method in the framework of optimization. Let  be a lower semi-continuous function defined on a complete metric space
be a lower semi-continuous function defined on a complete metric space  , with values in the extended line
, with values in the extended line 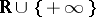 , and bounded from below. It is well known that the lower bound of
, and bounded from below. It is well known that the lower bound of  over
over  need not be attained. Ekeland's basic principle asserts that there exists a slight perturbation of
need not be attained. Ekeland's basic principle asserts that there exists a slight perturbation of  which attains its minimum on
which attains its minimum on  . More precisely, there exists a point
. More precisely, there exists a point  such that
such that  for all
for all 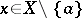 ; this says that the function
; this says that the function 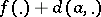 has a strict minimum on
has a strict minimum on 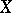 at
at  . It is interesting to observe that the conclusion of the basic principle is equivalent to the existence of a maximal element in the epigraph
. It is interesting to observe that the conclusion of the basic principle is equivalent to the existence of a maximal element in the epigraph  for the order defined on
for the order defined on  by
by 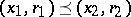 if and only if
if and only if 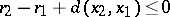 [a3].
[a3].

Figure: e110030a
From this basic principle one can deduce some variants which are in fact equivalent to the basic statement. The first one is as follows: given 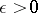 ,
,  such that
such that 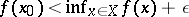 and applying the basic principle to the complete metric space
and applying the basic principle to the complete metric space  , one obtains the existence of a point
, one obtains the existence of a point 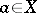 such that
such that 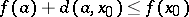 and
and  for all
for all  . In particular, this implies that
. In particular, this implies that 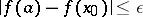 . Applying the previous result with the metric
. Applying the previous result with the metric 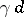 ,
, 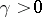 , yields the second variant: there exists an
, yields the second variant: there exists an  such that
such that
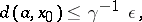 |
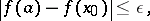 |
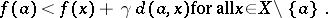 |
This variational principle has several equivalent geometric formulations. For instance, the Phelps extremization principle and the Drop theorem [a7], [a12] (see [a13] for the versions as stated here). Let  be a closed subset of a Banach space
be a closed subset of a Banach space  , let
, let  and let
and let  be a closed convex bounded subset of
be a closed convex bounded subset of  such that
such that 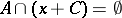 . Then there exist a
. Then there exist a  and a
and a 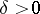 such that
such that 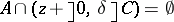 .
.
Among the great number of applications is the celebrated Bröndsted–Rockafellar theorem in convex analysis [a6]. Let 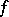 be a closed convex function defined on a real Banach space
be a closed convex function defined on a real Banach space 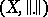 with values in
with values in 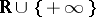 (cf. also Convex function (of a real variable)). Let
(cf. also Convex function (of a real variable)). Let  ,
, 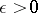 , and let
, and let  be such that
be such that 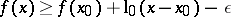 for all
for all 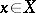 . One can apply the third version of the theorem, with
. One can apply the third version of the theorem, with 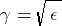 , to the function
, to the function 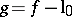 when endowing
when endowing 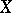 with the equivalent norm
with the equivalent norm 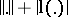 [a4]. This yields the existence of an
[a4]. This yields the existence of an 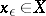 and an
and an
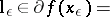 |
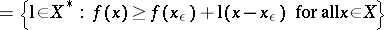 |
such that
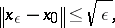 |
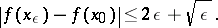 |
Hence the set
 |
is dense in 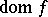 for the epigraph topology, i.e. the supremum of the norm topology on
for the epigraph topology, i.e. the supremum of the norm topology on  and of the initial topology associated to
and of the initial topology associated to  .
.
Another easy consequence of the Ekeland variational principle is a generalization to multi-functions of the Kirk–Caristi fixed-point theorem [a2].
Finally, it should be mentioned that analogous results hold in Banach spaces with the perturbation  replaced by some smooth one [a5].
replaced by some smooth one [a5].
References
| [a1] | H. Attouch, H. Riahi, "Stability results for the Ekeland's variational principle and cone extremal solutions" Math. Oper. Res. , 18 (1993) pp. 173–201 |
| [a2] | J.-P. Aubin, I. Ekeland, "Applied nonlinear analysis" , Wiley (1984) |
| [a3] | E. Bishop, R.R. Phelps, "The support functional of a convex set" P. Klee (ed.) , Convexity , Proc. Symp. Pure Math. , 7 , Amer. Math. Soc. (1963) pp. 27–35 |
| [a4] | J.M. Borwein, "A note on  -subgradients and maximal monotonicity" Pacific J. Math. , 103 (1982) pp. 307–314 -subgradients and maximal monotonicity" Pacific J. Math. , 103 (1982) pp. 307–314 |
| [a5] | J.M. Borwein, R. Preiss, "Smooth variational principle" Trans. Amer. Math. Soc. , 303 (1987) pp. 517–527 |
| [a6] | A. Bröndsted, R.T. Rockafellar, "On the subdifferentiability of convex functions" Proc. Amer. Math. Soc. , 16 (1965) pp. 605–611 |
| [a7] | F.H. Clarke, "Optimization and nonsmooth analysis" , Wiley (1983) |
| [a8] | J. Daneš, "A geometric theorem useful in nonlinear functional analysis" Boll. Un. Mat. Ital. , 4 (1972) pp. 369–375 |
| [a9] | D.G. de Figueiredo, "The Ekeland variational principle, tours and detours" , Lecture Notes Tata Inst. , Springer (1989) |
| [a10] | I. Ekeland, "On the variational principle" J. Math. Anal. Appl. , 47 (1974) pp. 324–353 |
| [a11] | I. Ekeland, "Nonconvex minimization problems" Bull. Amer. Math. Soc. (N.S.) , 1 (1979) pp. 443–474 |
| [a12] | J.-P. Penot, "The drop theorem, the petal theorem and Ekeland's variational principle" Nonlinear Anal.: Theory, Methods, Appl. , 10 (1986) pp. 813–822 |
| [a13] | J.S. Treiman, "Characterization of Clarke's tangent and normal cones in finite and infinite dimensions" Nonlinear Anal.: Theory, Methods, Appl. , 7 (1983) pp. 771–783 |
| [a14] | J.D. Weston, "A characterization of metric completeness" Proc. Amer. Math. Soc. , 64 (1977) pp. 186–188 |
Ekeland variational principle. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ekeland_variational_principle&oldid=17233