Weyl-Otsuki space

Otsuki–Weyl space

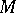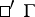An Otsuki space [a6], [a7] is a manifold endowed with two different linear connections and (cf. also Connections on a manifold) and a non-degenerate 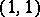tensor field 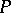of constant rank (cf. also tensor analysis), where the connection coefficients 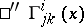, , 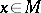are used in the computation of the contravariant, and the in the computation of the covariant, components of the invariant (covariant) differential of a tensor (vector). For a tensor field of type 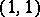, the invariant differential and the covariant differential 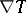have the following forms

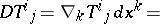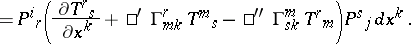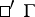and 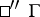are connected by the relation

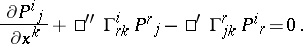Thus, 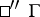and determine 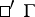. T. Otsuki calls these a general connection. For 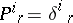one obtains 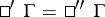and the usual invariant differential.

If 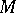is endowed also with a Riemannian metric 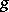, then may be the Christoffel symbol .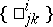

In a Weyl space 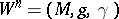one has 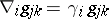. A Weyl–Otsuki space 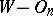[a1] is a 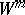endowed with an Otsuki connection. The 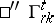are defined here as

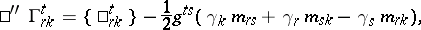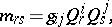where 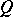is the inverse of 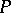. 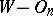spaces were studied mainly by A. Moór [a2], [a3].

He extended the Otsuki connection also to affine and metrical line-element spaces, obtaining Finsler–Otsuki spaces 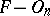[a4], [a5] with invariant differential

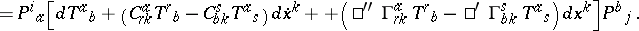Here, all objects depend on the line-element 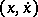, the 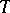, , 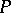, 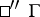are homogeneous of order , and 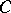is a tensor.

References

[a1]	A. Moór, "Otsukische Übertragung mit rekurrenter Maß tensor" Acta Sci. Math. , 40 (1978) pp. 129–142
[a2]	A. Moór, "Über verschiedene geodätische Abweichungen in Weyl–Otsukischen Räumen" Publ. Math. Debrecen , 28 (1981) pp. 247–258
[a3]	A. Moór, "Über Transformationsgruppen in Weyl–Otsukischen Räumen" Publ. Math. Debrecen , 29 (1982) pp. 241–250
[a4]	A. Moór, "Über die Begründung von Finsler–Otschukischen Räumen und ihre Dualität" Tensor N.S. , 37 (1982) pp. 121–129
[a5]	A. Moór, "Über spezielle Finsler–Otsukische Räume" Publ. Math. Debrecen , 31 (1984) pp. 185–196
[a6]	T. Otsuki, "On general connections. I" Math. J. Okayama Univ. , 9 (1959-60) pp. 99–164
[a7]	T. Otsuki, "On metric general connections" Proc. Japan Acad. , 37 (1961) pp. 183–188