Range (of variation of a sample)

The difference

$$w_n=x_\mathrm{max}-x_\mathrm{min}$$

between the largest $x_\mathrm{max}=x_n$ and smallest $x_\mathrm{min}=x_1$ values in the ordered sample

$$(x_1,\ldots,x_n),\quad x_1\leq\ldots\leq x_n,$$

obtained by taking $n$ independent measurements of the same random variable $X$. Let be the distribution function of the random variable $X$. Then the probability distribution for the range is

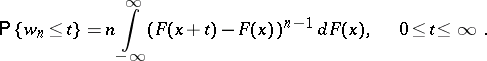References

[1]	B.L. van der Waerden, "Mathematische Statistik" , Springer (1957)
[2]	L.N. Bol'shev, N.V. Smirnov, "Tables of mathematical statistics" , Libr. math. tables , 46 , Nauka (1983) (In Russian) (Processed by L.S. Bark and E.S. Kedrova)

Comments

The range of variation of a sample is also called the sample range.

References

[a1]	D.B. Owen, "Handbook of statistical tables" , Addison-Wesley (1962)