Massless Klein-Gordon equation
The Klein–Gordon equation [a1], [a2], [a3]
 |
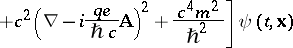 |
for the case where the mass parameter  is equal to zero. The constant
is equal to zero. The constant  stands for the speed of light,
stands for the speed of light,  is the charge of the positron,
is the charge of the positron,  where
where  is the Planck constant,
is the Planck constant,  are the time, respectively space, variables, and
are the time, respectively space, variables, and  is the imaginary unit. The (complex-valued) solution
is the imaginary unit. The (complex-valued) solution 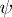 describes the wave function of a relativistic spinless and massless particle with charge
describes the wave function of a relativistic spinless and massless particle with charge 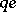 in the exterior electro-magnetic field
in the exterior electro-magnetic field  . It is a second-order, hyperbolic partial differential equation. Solutions are being studied in, e.g., [a4], [a5].
. It is a second-order, hyperbolic partial differential equation. Solutions are being studied in, e.g., [a4], [a5].
If the outer field is zero, 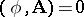 , or the coupling of the spin to the magnetic potential
, or the coupling of the spin to the magnetic potential 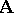 can be neglected, the massless Klein–Gordon equation also can be used for the description of massless spin-carrying particles, such as e.g. photons. In the case without outer fields the massless Klein–Gordon equation becomes equivalent to the wave equation with wave speed
can be neglected, the massless Klein–Gordon equation also can be used for the description of massless spin-carrying particles, such as e.g. photons. In the case without outer fields the massless Klein–Gordon equation becomes equivalent to the wave equation with wave speed  and is independent of the magnitude of Planck's constant
and is independent of the magnitude of Planck's constant  . This explains, why the wave nature of massless particles, such as e.g. photons ( "light" ), can also be observed on a macroscopic scale — in contrast with the wave nature of massive particles (cf.also Massless field; Massive field).
. This explains, why the wave nature of massless particles, such as e.g. photons ( "light" ), can also be observed on a macroscopic scale — in contrast with the wave nature of massive particles (cf.also Massless field; Massive field).
The interpretation of the wave function 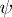 as a quantum mechanical "probability amplitude" (similarly as in the case of the Schrödinger equation), however, is not consistent, since the quantity
as a quantum mechanical "probability amplitude" (similarly as in the case of the Schrödinger equation), however, is not consistent, since the quantity  in general depends on the time parameter
in general depends on the time parameter 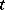 . Furthermore, the existence of negative frequency solutions is in contrast with the required lower boundedness of the energy ( "stability of matter" ). These problems are resolved through a re-interpretation of
. Furthermore, the existence of negative frequency solutions is in contrast with the required lower boundedness of the energy ( "stability of matter" ). These problems are resolved through a re-interpretation of 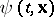 as a quantum field (cf. Quantum field theory), see e.g. [a6], [a7].
as a quantum field (cf. Quantum field theory), see e.g. [a6], [a7].
In recent time (as of 2000) solutions of the Klein–Gordon equation on Lorentzian manifolds have attracted increasing attention in connection with the theory of quantized fields on curved space-time, cf. e.g. [a8].
References
| [a1] | O. Klein, "Quantentheorie und fünfdimensionale Relativitätstheorie" Z. f. Phys. , 37 (1926) pp. 895 |
| [a2] | O. Gordon, "Der Comptoneffekt nach der Schrödingerschen Theorie" Z. f. Phys. , 40 (1926) pp. 117 |
| [a3] | E. Schrödinger, "Quantisierung als Eigenwertproblem IV" Ann. Phys. , 81 (1926) pp. 109 |
| [a4] | L. Gross, "Norm invariance of mass zero equations under the conformal group" J. Math. Phys. , 5 (1964) pp. 687–695 |
| [a5] | E.M. de Jager, "The Lorentz-invariant solutions of the Klein–Gordon equation I-II" Indag. Math. , 25 (1963) pp. 515–531; 546–558 |
| [a6] | R. Jost, "The general theory of quantised fields" , Amer. Math. Soc. (1965) |
| [a7] | S. Weinberg, "The quantum theory of fields" , I , Cambridge Univ. Press (1995) |
| [a8] | S.A. Fulling, "Aspects of quantum field theory in curved space-time" , Cambridge Univ. Press (1989) |
Massless Klein-Gordon equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Massless_Klein-Gordon_equation&oldid=14731