Ellipsoidal coordinates
spatial elliptic coordinates
The numbers  ,
,  and
and  connected with Cartesian rectangular coordinates
connected with Cartesian rectangular coordinates  ,
,  and
and  by the formulas
by the formulas
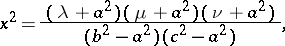 |
 |
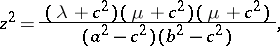 |
where  . The coordinate surfaces are (see Fig.): ellipses
. The coordinate surfaces are (see Fig.): ellipses  , one-sheet hyperbolas (
, one-sheet hyperbolas ( ), and two-sheet hyperbolas (
), and two-sheet hyperbolas ( ), with centres at the coordinate origin.
), with centres at the coordinate origin.

Figure: e035420a
The system of ellipsoidal coordinates is orthogonal. To every triple of numbers 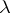 ,
,  and
and  correspond 8 points (one in each octant), which are symmetric to each other relative to the coordinate planes of the system
correspond 8 points (one in each octant), which are symmetric to each other relative to the coordinate planes of the system 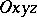 .
.
The Lamé coefficients are
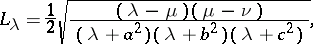 |
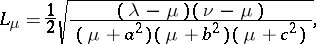 |
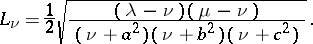 |
If one of the conditions  in the definition of ellipsoidal coordinates is replaced by an equality, then degenerate ellipsoidal coordinate systems are obtained.
in the definition of ellipsoidal coordinates is replaced by an equality, then degenerate ellipsoidal coordinate systems are obtained.
Comments
References
| [a1] | G. Darboux, "Leçons sur la théorie générale des surfaces et ses applications géométriques du calcul infinitésimal" , 1 , Gauthier-Villars (1887) pp. 1–18 |
| [a2] | Harold Jeffreys, Bertha Jeffreys, Methods of Mathematical Physics, 3rd edition, Cambridge University Press (1972) Zbl 0238.00004 |
Ellipsoidal coordinates. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ellipsoidal_coordinates&oldid=14024