Kolmogorov-Chapman equation
2020 Mathematics Subject Classification: Primary: 60J35 [MSN][ZBL]
An equation of the form
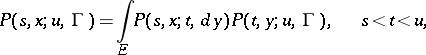 |
that is, a condition imposed on the transition function  (
( ,
,  ,
, 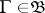 ,
, 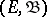 being a measurable space), enabling one (under certain conditions on
being a measurable space), enabling one (under certain conditions on 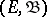 ) to construct a Markov process for which the conditional probability
) to construct a Markov process for which the conditional probability  is the same as
is the same as 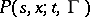 . Conversely, for a Markov process its transition function
. Conversely, for a Markov process its transition function 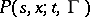 , which by definition is equal to
, which by definition is equal to 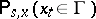 , satisfies the Kolmogorov–Chapman equation, as follows immediately from general properties of conditional probabilities. This was pointed out by S. Chapman [1] and investigated by A.N. Kolmogorov in 1931 (see [2]).
, satisfies the Kolmogorov–Chapman equation, as follows immediately from general properties of conditional probabilities. This was pointed out by S. Chapman [1] and investigated by A.N. Kolmogorov in 1931 (see [2]).
References
| [1] | S. Chapman, Proc. Roy. Soc. Ser. A , 119 (1928) pp. 34–54 |
| [2] | A. [A.N. Kolmogorov] Kolmogoroff, "Ueber die analytischen Methoden in der Wahrscheinlichkeitsrechnung" Math. Ann. , 104 (1931) pp. 415–458 |
| [3] | I.I. [I.I. Gikhman] Gihman, A.V. [A.V. Skorokhod] Skorohod, "The theory of stochastic processes" , 2 , Springer (1975) (Translated from Russian) |
Comments
In Western literature this equation is usually referred to as the Chapman–Kolmogorov equation.
See also (the editorial comments to) Einstein–Smoluchowski equation.
References
| [a1] | P. Lévy, "Processus stochastiques et mouvement Brownien" , Gauthier-Villars (1965) |
| [a2] | E.B. Dynkin, "Markov processes" , 1 , Springer (1965) pp. Sect. 5.26 (Translated from Russian) |
| [a3] | W. Feller, "An introduction to probability theory and its applications" , 1 , Wiley (1966) pp. Chapt. XV.13 |
Kolmogorov-Chapman equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kolmogorov-Chapman_equation&oldid=13649