Kerdock and Preparata codes
A most famous relation in coding theory is a discrete avatar of the Fourier transform which relates the weight enumerator  of a linear code
of a linear code  and the weight enumerator of its dual
and the weight enumerator of its dual  with respect to the standard scalar product, namely
with respect to the standard scalar product, namely
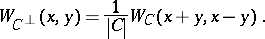 |
(Cf. MacWilliams identities.) A simple example of a dual pair of codes is the Hamming code  , a (big)
, a (big)  code whose dual is the (small) first-order Reed–Muller code
code whose dual is the (small) first-order Reed–Muller code  . The matrix
. The matrix
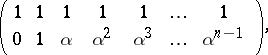 |
where  and
and 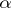 is a root of
is a root of  , is both a generator matrix for
, is both a generator matrix for 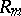 and a parity check matrix for
and a parity check matrix for 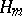 . The small code
. The small code  has the simple weight distribution
has the simple weight distribution
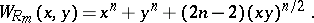 |
The MacWilliams formula now gives an explicit expression for 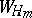 which would be cumbersome to obtain directly.
which would be cumbersome to obtain directly.
There is no Fourier transform without Abelian groups and therefore there is no MacWilliams formula for non-linear codes. The discovery first of the 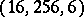 Nordstrom–Robinson code in 1967, non-linear and still formally self-dual for the MacWilliams relation, followed in 1968 and 1972 [a8] by the discovery of two infinite families of non-linear codes, the Preparata codes and Kerdock codes, respectively, whose weight enumerators are MacWilliams dual of each other, were until [a4] an unexplained phenomenon. The unsuccessful efforts of many distinguished researchers on this notoriously difficult problem [a8] led one of them to declare [a5] that it was "merely a coincidence" .
Nordstrom–Robinson code in 1967, non-linear and still formally self-dual for the MacWilliams relation, followed in 1968 and 1972 [a8] by the discovery of two infinite families of non-linear codes, the Preparata codes and Kerdock codes, respectively, whose weight enumerators are MacWilliams dual of each other, were until [a4] an unexplained phenomenon. The unsuccessful efforts of many distinguished researchers on this notoriously difficult problem [a8] led one of them to declare [a5] that it was "merely a coincidence" .
A well-known trick in modulation theory to address the  -PSK constellation consists of using a (very simple) case of the Gray mapping. This is a mapping from
-PSK constellation consists of using a (very simple) case of the Gray mapping. This is a mapping from  to
to  defined by
defined by
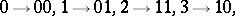 |
and extended to a mapping from 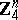 to
to  in the natural way. The key property is that the mapping
in the natural way. The key property is that the mapping  from
from  equipped with the Lee distance to
equipped with the Lee distance to  equipped with the Hamming distance is an isometric mapping of metric spaces. The trace parametrization of quaternary
equipped with the Hamming distance is an isometric mapping of metric spaces. The trace parametrization of quaternary  -sequences can be used to show that the Kerdock code as defined in [a8], p. 1107, is essentially the cyclic code associated to construction
-sequences can be used to show that the Kerdock code as defined in [a8], p. 1107, is essentially the cyclic code associated to construction  of [a1], p. 458, and the construction of [a9]. In particular, if
of [a1], p. 458, and the construction of [a9]. In particular, if 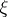 denotes a primitive root of
denotes a primitive root of  in a suitable Galois ring, [a7], then the matrix
in a suitable Galois ring, [a7], then the matrix
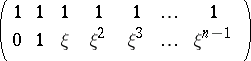 |
( , with
, with  odd) is both a generator matrix for the Kerdock code and a parity check matrix for the "Preparata" code. One sees that the Kerdock and Preparata codes are quaternary analogues of the first-order Reed–Muller code and of the extended Hamming code, respectively. The Goethals and Delsarte–Goethals codes, which are also a quaternary dual pair, are related in a less simple manner to three error-correcting BCH codes.
odd) is both a generator matrix for the Kerdock code and a parity check matrix for the "Preparata" code. One sees that the Kerdock and Preparata codes are quaternary analogues of the first-order Reed–Muller code and of the extended Hamming code, respectively. The Goethals and Delsarte–Goethals codes, which are also a quaternary dual pair, are related in a less simple manner to three error-correcting BCH codes.
Besides MacWilliams duality, the  structure also bears influence on the decoding (cf. [a4], Fig. 2) and on the coset structure of the Preparata code, which gives rise to a new distance-regular graph of diameter
structure also bears influence on the decoding (cf. [a4], Fig. 2) and on the coset structure of the Preparata code, which gives rise to a new distance-regular graph of diameter 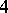 [a4].
[a4].
The article [a4], which solves a twenty-year-old riddle, was awarded the 1995 IEEE Information Theory award for best paper. Geometrical repercussions can be found in [a3], [a6].
References
| [a1] | S. Boztas, A.R. Hammons, P. V. Kumar, "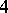 -phase sequence with near optimum correlation properties" IEEE Trans. Inform. Th. , 38 (1992) pp. 1101–1113 -phase sequence with near optimum correlation properties" IEEE Trans. Inform. Th. , 38 (1992) pp. 1101–1113 |
| [a2] | J.H. Conway, N.J.A. Sloane, "Sphere packings, lattices and groups" , Springer (1992) |
| [a3] | A.R. Calderbank, P.J. Cameron, W.M. Kantor, J.J. Seidel, "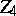 -Kerdock codes, orthogonal spreads, and extremal euclidean line sets" Proc. London Math. Soc. (to appear) -Kerdock codes, orthogonal spreads, and extremal euclidean line sets" Proc. London Math. Soc. (to appear) |
| [a4] | A.R. Hammons, P.V. Kumar, A.R. Calderbank, N.J.A. Sloane, P. Solé, "The 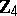 -linearity of Kerdock, Preparata, Goethals, and related codes" IEEE Trans. Information Th. , 40 (1994) pp. 301–319 -linearity of Kerdock, Preparata, Goethals, and related codes" IEEE Trans. Information Th. , 40 (1994) pp. 301–319 |
| [a5] | W.M. Kantor, "On the inequivalence of generalized Preparata codes" IEEE Inform. Th. , 29 (1983) pp. 345–348 |
| [a6] | W.M. Kantor, "Quaternionic line sets and quaternionic Kerdock codes" Linear Alg. & Its Appl. (special issue in honor of J.J. Seidel) , 226–228 (1995) pp. 749–779 |
| [a7] | B.R. MacDonald, "Finite rings with identity" , M. Dekker (1974) |
| [a8] | F.J. MacWilliams, N.J.A. Sloane, "The theory of error-correcting codes" , North-Holland (1977) |
| [a9] | P. Solé, "A quaternary cyclic code and a family of quaternary sequences with low correlation" , Lecture Notes in Computer Science , 388 , Springer (1989) pp. 193–201 |
Kerdock and Preparata codes. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kerdock_and_Preparata_codes&oldid=13222