Multiplication
of numbers
One of the basic arithmetic operations. Multiplication consists in assigning to two numbers  (called the factors) a third number
(called the factors) a third number  (called the product). Multiplication is denoted by the sign
(called the product). Multiplication is denoted by the sign  or
or  ; in notations using letters the sign is, as a rule, omitted.
; in notations using letters the sign is, as a rule, omitted.
Multiplication of positive integers is defined in the following way by means of addition: the product of a number  by a number
by a number  is the number
is the number  equal to the sum of
equal to the sum of  summands each of which is equal to
summands each of which is equal to  ; thus,
; thus,
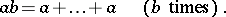 |
The number  is called the multiplicand and
is called the multiplicand and 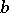 the multiplier. Multiplication of two positive rational numbers
the multiplier. Multiplication of two positive rational numbers  and
and 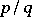 is defined by the equation
is defined by the equation
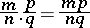 |
(cf. Fraction). The product of two negative fractions is positive, and that of a positive and a negative fraction is negative, where the modulus of the product in both cases is equal to the product of the moduli of the factors. The product of irrational numbers is defined as the limit of the products of rational approximations of them. Multiplication of two complex numbers 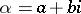 and
and 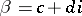 is given by the formula
is given by the formula
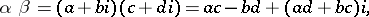 |
or, in trigonometric form  , by
, by
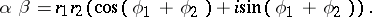 |
Multiplication of numbers is commutative, associative and distributive on the left and right relative to addition (cf. Commutativity; Associativity; Distributivity). Further,  ,
,  .
.
In general algebra, multiplication may be any algebraic operation ( -ary,
-ary, 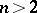 ); most frequently a binary operation (cf. Magma). In certain cases this operation is a generalization of the usual multiplication of numbers. E.g. multiplication of quaternions, multiplication of matrices and multiplication of transformations. However, properties of the multiplication of numbers (e.g. commutativity) may be lost in these cases.
); most frequently a binary operation (cf. Magma). In certain cases this operation is a generalization of the usual multiplication of numbers. E.g. multiplication of quaternions, multiplication of matrices and multiplication of transformations. However, properties of the multiplication of numbers (e.g. commutativity) may be lost in these cases.
Multiplication. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Multiplication&oldid=13209