|
|
| Line 1: |
Line 1: |
| − | ''<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201303.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201304.png" />''
| + | <!--This article has been texified automatically. Since there was no Nroff source code for this article, |
| | + | the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist |
| | + | was used. |
| | + | If the TeX and formula formatting is correct, please remove this message and the {{TEX|semi-auto}} category. |
| | | | |
| − | Spaces of analytic functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201305.png" /> on an open set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201306.png" /> in the complex plane for which
| + | Out of 111 formulas, 96 were replaced by TEX code.--> |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201307.png" /></td> </tr></table>
| + | {{TEX|semi-auto}}{{TEX|partial}} |
| | + | ''$L _ { a } ^ { p } ( G )$, $0 < p < \infty$'' |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201308.png" /> is the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b1201309.png" />-dimensional [[Lebesgue measure|Lebesgue measure]] on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013010.png" />.
| + | Spaces of analytic functions $f$ on an open set $G$ in the complex plane for which |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013011.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013012.png" /> is a [[Hilbert space|Hilbert space]] and has a reproducing kernel <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013013.png" /> satisfying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013014.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013015.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013016.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013017.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013018.png" /> (cf. also [[Bergman kernel function|Bergman kernel function]]). The strong connections between reproducing kernels, conformal mappings (cf. also [[Conformal mapping|Conformal mapping]]), [[Harmonic measure|harmonic measure]], and elliptic partial differential equations (cf. also [[Elliptic partial differential equation|Elliptic partial differential equation]]) motivated the pioneering work of S. Bergman [[#References|[a7]]]; see also, e.g., [[#References|[a6]]].
| + | \begin{equation*} \| f \| _ { p , G } ^ { p } = \int_G | f ( z ) | ^ { p } d A ( z ) < \infty , \end{equation*} |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013019.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013020.png" /> is a closed subspace of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013021.png" />; thus, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013022.png" /> it is a [[Banach space|Banach space]] with norm <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013023.png" />. Furthermore, a function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013024.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013025.png" />, has an almost-everywhere representative in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013026.png" /> if and only if
| + | where $d A ( z ) = d x d y$ is the $2$-dimensional [[Lebesgue measure|Lebesgue measure]] on $G$. |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013027.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a1)</td></tr></table>
| + | If $p = 2$, then $L _ { a } ^ { 2 } ( G )$ is a [[Hilbert space|Hilbert space]] and has a reproducing kernel $k _ { z } ( w )$ satisfying $f ( z ) = \int _ { G } f ( w ) \overline { k _ { z } ( w ) } d A ( w )$ for all $f$ in $L _ { a } ^ { 2 } ( G )$ and $z$ in $G$ (cf. also [[Bergman kernel function|Bergman kernel function]]). The strong connections between reproducing kernels, conformal mappings (cf. also [[Conformal mapping|Conformal mapping]]), [[Harmonic measure|harmonic measure]], and elliptic partial differential equations (cf. also [[Elliptic partial differential equation|Elliptic partial differential equation]]) motivated the pioneering work of S. Bergman [[#References|[a7]]]; see also, e.g., [[#References|[a6]]]. |
| | | | |
| − | for all compactly supported functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013028.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013029.png" />. It is a result of V. Havin that via the Bergman–Sobolev duality (a1) the annihilator of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013030.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013031.png" />, can be identified with the [[Sobolev space|Sobolev space]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013032.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013033.png" />, [[#References|[a11]]]. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013034.png" />, then the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013035.png" /> is non-trivial if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013036.png" /> contains at least two points. <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013037.png" /> is non-trivial if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013038.png" /> has positive [[Logarithmic capacity|logarithmic capacity]], and more generally, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013039.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013040.png" /> is non-trivial if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013041.png" /> has positive <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013042.png" />-capacity, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013043.png" />, [[#References|[a8]]], [[#References|[a12]]].
| + | If $p > 0$, then $L _ { a } ^ { p } ( G )$ is a closed subspace of $L ^ { p } ( G )$; thus, for $p \geq 1$ it is a [[Banach space|Banach space]] with norm $\| f \| _ { p , G}$. Furthermore, a function $f \in L ^ { p } ( G )$, $p \geq 1$, has an almost-everywhere representative in $L _ { a } ^ { p } ( G )$ if and only if |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013044.png" /> is bounded, then the Cauchy transform (cf. also [[Cauchy integral|Cauchy integral]]) provides many examples of functions in a Bergman space. Indeed, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013045.png" /> is a finite complex [[Measure|measure]] with compact support in the complement of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013046.png" /> and if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013047.png" />, then the Cauchy transform
| + | \begin{equation} \tag{a1} \int _ { G } f \overline { \partial } \varphi d A = 0 \end{equation} |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013048.png" /></td> </tr></table>
| + | for all compactly supported functions $\varphi$ in $C _ { c } ^ { \infty } ( G )$. It is a result of V. Havin that via the Bergman–Sobolev duality (a1) the annihilator of $L _ { a } ^ { p } ( G )$, $p > 1$, can be identified with the [[Sobolev space|Sobolev space]] $W _ { 0 } ^ { q , 1 } ( G )$, $1 / p + 1 / q = 1$, [[#References|[a11]]]. If $1 < p < 2$, then the space $L _ { a } ^ { p } ( G )$ is non-trivial if and only if $\mathbf{C} \backslash G$ contains at least two points. $L _ { a } ^ { 2 } ( G )$ is non-trivial if and only if $\mathbf{C} \backslash G$ has positive [[Logarithmic capacity|logarithmic capacity]], and more generally, for $p > 2$, $L _ { a } ^ { p } ( G )$ is non-trivial if and only if $\mathbf{C} \backslash G$ has positive $q$-capacity, $1 / p + 1 / q = 1$, [[#References|[a8]]], [[#References|[a12]]]. |
| | | | |
| − | is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013049.png" /> if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013050.png" /> has finite <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013051.png" />-energy, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013052.png" />, cf. also [[Energy of measures|Energy of measures]]. In fact, such Cauchy transforms are dense in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013053.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013054.png" /> bounded), [[#References|[a12]]]. | + | If $G$ is bounded, then the Cauchy transform (cf. also [[Cauchy integral|Cauchy integral]]) provides many examples of functions in a Bergman space. Indeed, if $\mu$ is a finite complex [[Measure|measure]] with compact support in the complement of $G$ and if $p > 1$, then the Cauchy transform |
| | | | |
| − | When <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013055.png" /> equals the unit disc <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013056.png" />, then one writes <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013057.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013058.png" />. For each <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013059.png" /> one can identify the dual <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013060.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013061.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013062.png" />, with the duality <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013063.png" /> with equivalence of norms. One also has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013064.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013065.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013066.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013067.png" /> are the Bloch and the little Bloch spaces (cf. also [[Bloch function|Bloch function]]). Furthermore, harmonic conjugation defines a bounded linear transformation on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013068.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013069.png" />, i.e. for each <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013070.png" /> there is a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013071.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013072.png" /> for every [[Harmonic function|harmonic function]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013073.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013074.png" />; here, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013075.png" /> is the harmonic conjugate of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013076.png" />, ([[#References|[a3]]]).
| + | \begin{equation*} C _ { \mu } ( z ) = \int \frac { 1 } { z - w } d \mu ( w ) \end{equation*} |
| | | | |
| − | For the [[Topological algebra|topological algebra]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013077.png" />, B. Korenblum has established a theory that parallels the theory of [[Hardy spaces|Hardy spaces]] with regard to Riesz factorization and invariant subspaces (see [[#References|[a15]]] [[#References|[a16]]]).
| + | is in $L _ { a } ^ { p } ( G )$ if and only if $\mu$ has finite $q$-energy, $1 / p + 1 / q = 1$, cf. also [[Energy of measures|Energy of measures]]. In fact, such Cauchy transforms are dense in $L _ { a } ^ { p } ( G )$ ($G$ bounded), [[#References|[a12]]]. |
| | | | |
| − | For a fixed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013078.png" /> one notes that the Hardy space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013079.png" /> is contained in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013080.png" />, and it is useful to contrast the function theory in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013081.png" /> (as known today (1998)) with the classical situation in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013082.png" />. A general statement one can make is that results for functions in a Bergman space depend on the parameter <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013083.png" />.
| + | When $G$ equals the unit disc $D = \{ z \in \mathbf{C} : | z | < 1 \}$, then one writes $L _ { a } ^ { p } ( G ) = L _ { a } ^ { p }$ or $A ^ { p }$. For each $p > 1$ one can identify the dual <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013060.png"/> with $L _ { a } ^ { q }$, $1 / p + 1 / q = 1$, with the duality $\langle f , g \rangle = \int _ { D } f \overline{g} d A$ with equivalence of norms. One also has $B _ { 0 } ^ { * } \cong L _ { a } ^ { 1 }$ and $L _ { a } ^ { 1 * } \cong B$, where $B$ and $B_0$ are the Bloch and the little Bloch spaces (cf. also [[Bloch function|Bloch function]]). Furthermore, harmonic conjugation defines a bounded linear transformation on $L ^ { p }$ for all $p \geq 1$, i.e. for each $p \geq 1$ there is a $c > 0$ such that $\| \tilde { u } \| _ { p } \leq c \| u \| _ { p }$ for every [[Harmonic function|harmonic function]] $u$ on $D$; here, $\tilde{u}$ is the harmonic conjugate of $u$, ([[#References|[a3]]]). |
| | | | |
| − | While a precise characterization of the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013085.png" />-zero sets is open (1998), certain asymptotic results are known, [[#References|[a20]]], [[#References|[a21]]]. Every function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013086.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013087.png" /> admits an "Lap-inner-Lap-outer" factorization <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013088.png" />, [[#References|[a1]]]. A function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013089.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013090.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013091.png" />, is called <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013093.png" />-inner if
| + | For the [[Topological algebra|topological algebra]] $A ^ { - \infty } = \cup _ { p > 0 } L _ { a } ^ { p }$, B. Korenblum has established a theory that parallels the theory of [[Hardy spaces|Hardy spaces]] with regard to Riesz factorization and invariant subspaces (see [[#References|[a15]]] [[#References|[a16]]]). |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013094.png" /></td> </tr></table> | + | For a fixed $p > 0$ one notes that the Hardy space $H ^ { p }$ is contained in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013080.png"/>, and it is useful to contrast the function theory in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013081.png"/> (as known today (1998)) with the classical situation in $H ^ { p }$. A general statement one can make is that results for functions in a Bergman space depend on the parameter $p > 0$. |
| | | | |
| − | for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013095.png" />, and a function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013096.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013097.png" /> is called <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013099.png" />-outer if whenever <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130100.png" /> is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130101.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130102.png" /> for all analytic polynomials <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130103.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130104.png" /> (see [[#References|[a17]]]). <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130105.png" />-outer functions are cyclic in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130106.png" />, yet, no explicit function-theoretic descriptions for either the inner or outer functions are known (1998). The inner factors are sometimes called the contractive divisors, because <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130107.png" />, whenever <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130108.png" /> is in the invariant subspace generated by the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130109.png" />-inner function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130110.png" />, [[#References|[a10]]], [[#References|[a13]]]. General invariant subspaces of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130111.png" /> do not have to be cyclic (i.e. generated by one function) (see [[#References|[a2]]], [[#References|[a14]]]), but for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130112.png" /> one knows that they are generated by a collection of inner functions, [[#References|[a1]]].
| + | While a precise characterization of the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013085.png"/>-zero sets is open (1998), certain asymptotic results are known, [[#References|[a20]]], [[#References|[a21]]]. Every function $f$ in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013087.png"/> admits an "Lap-inner-Lap-outer" factorization $f = \varphi F$, [[#References|[a1]]]. A function $\varphi$ in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013090.png"/>, $\| \varphi \| _ { p } = 1$, is called <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013093.png"/>-inner if |
| | | | |
| − | Interpolating sequences and sampling sequences for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130115.png" /> have been characterized by K. Seip [[#References|[a19]]].
| + | \begin{equation*} \int _ { D } z ^ { n } | \varphi ( z ) | ^ { p } d A ( z ) = 0 \end{equation*} |
| | | | |
| − | There also are well-developed theories of Toeplitz, Hankel, and composition operators on Bergman spaces, and work on Bergman spaces on domains in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130116.png" /> has started (see e.g. [[#References|[a22]]], [[#References|[a9]]], [[#References|[a18]]], [[#References|[a4]]], [[#References|[a5]]]).
| + | for all $n > 0$, and a function $F$ in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013097.png"/> is called <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b12013099.png"/>-outer if whenever $g$ is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130101.png"/> with $\|hF\|_p \geq \|hg\|_p$ for all analytic polynomials $h$, then $|F(0)|\geq |h(0)|$ (see [[#References|[a17]]]). <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130105.png"/>-outer functions are cyclic in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130106.png"/>, yet, no explicit function-theoretic descriptions for either the inner or outer functions are known (1998). The inner factors are sometimes called the contractive divisors, because $\| f / \varphi \| _ { p } \leq \| f \| _ { p }$, whenever $f$ is in the invariant subspace generated by the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130109.png"/>-inner function $\varphi$, [[#References|[a10]]], [[#References|[a13]]]. General invariant subspaces of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130111.png"/> do not have to be cyclic (i.e. generated by one function) (see [[#References|[a2]]], [[#References|[a14]]]), but for $p = 2$ one knows that they are generated by a collection of inner functions, [[#References|[a1]]]. |
| | + | |
| | + | Interpolating sequences and sampling sequences for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130115.png"/> have been characterized by K. Seip [[#References|[a19]]]. |
| | + | |
| | + | There also are well-developed theories of Toeplitz, Hankel, and composition operators on Bergman spaces, and work on Bergman spaces on domains in $\mathbf{C} ^ { n }$ has started (see e.g. [[#References|[a22]]], [[#References|[a9]]], [[#References|[a18]]], [[#References|[a4]]], [[#References|[a5]]]). |
| | | | |
| | ====References==== | | ====References==== |
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> A. Aleman, S. Richter, C. Sundberg, "Beurling's theorem for the Bergman space" ''Acta Math.'' , '''177''' (1996) pp. 275–310</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> C. Apostol, H. Bercovici, C. Foias, C. Pearcy, "Invariant subspaces, dilation theory, and the structure of the predual of a dual algebra" ''J. Funct. Anal.'' , '''63''' (1985) pp. 369–404</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> S. Axler, "Bergman spaces and their operators" J.B. Conway (ed.) B.B. Morrel (ed.) , ''Surveys of Some Recent Results in Operator Theory I'' , ''Res. Notes Math.'' , '''171''' , Pitman (1988) pp. 1–50</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> S. Axler, D. Zheng, "Compact operators via the Berezin transform" ''Indiana J. Math.'' , '''49''' (1998) pp. 311</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> "Holomorphic Spaces" S. Axler (ed.) J. McCarthy (ed.) D. Sarason (ed.) , Cambridge Univ. Press (1998)</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> S. Bell, "The Cauchy transform, potential theory, and conformal mapping" , ''Studies Adv. Math.'' , CRC (1992)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> S. Bergman, "The kernel function and conformal mapping" , ''Math. Surveys'' , '''5''' , Amer. Math. Soc. (1950)</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> L. Carleson, "Selected problems on exceptional sets" , v. Nostrand (1967)</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> C. Cowen, B. MacCluer, "Composition operators on spaces of analytic functions" , ''Studies Adv. Math.'' , CRC (1995)</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> P. Duren, D. Khavinson, H. Shapiro, C. Sundberg, "Invariant subspaces in Bergman spaces and the biharmonic equation" ''Michigan Math. J.'' , '''41''' (1994) pp. 247–259</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> V.P. Havin, "Approximation in the mean by analytic functions" ''Soviet Math. Dokl.'' , '''9''' (1968) pp. 245–248</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> L. Hedberg, "Non linear potentials and approximation in the mean by analytic functions" ''Math. Z.'' , '''129''' (1972) pp. 299–319</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> H. Hedenmalm, "A factorization theorem for square area integrable functions" ''J. Reine Angew. Math.'' , '''422''' (1991) pp. 45–68</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> H. Hedenmalm, "An invariant subspace of the Bergman space having the codimension two property" ''J. Reine Angew. Math.'' , '''443''' (1993) pp. 1–9</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> B. Korenblum, "An extension of the Nevanlinna theory" ''Acta Math.'' , '''135''' (1975) pp. 187–219</TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> B. Korenblum, "A Beurling type theorem" ''Acta Math.'' , '''138''' (1977) pp. 265–293</TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> B. Korenblum, "Outer functions and cyclic elements in Bergman spaces" ''J. Funct. Anal.'' , '''115''' (1993) pp. 104–118</TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> H. Li, D. Luecking, "BMO on strongly pseudoconvex domains: Hankel operators, duality and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130117.png" />-estimates" ''Trans. Amer. Math. Soc.'' , '''346''' (1994) pp. 661–691</TD></TR><TR><TD valign="top">[a19]</TD> <TD valign="top"> K. Seip, "Beurling type density theorems in the unit disc" ''Invent. Math.'' , '''113''' (1993) pp. 21–39</TD></TR><TR><TD valign="top">[a20]</TD> <TD valign="top"> K. Seip, "On a theorem of Korenblum" ''Ark. Mat.'' , '''32''' (1994) pp. 237–243</TD></TR><TR><TD valign="top">[a21]</TD> <TD valign="top"> K. Seip, "On Korenblum's density condition for the zero sets of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b120/b120130/b120130118.png" />" ''J. Anal. Math.'' , '''67''' (1995) pp. 307–322</TD></TR><TR><TD valign="top">[a22]</TD> <TD valign="top"> K. Zhu, "Operator theory in function spaces" ''Pure Appl. Math.'' , '''139''' (1990)</TD></TR></table> | + | <table><tr><td valign="top">[a1]</td> <td valign="top"> A. Aleman, S. Richter, C. Sundberg, "Beurling's theorem for the Bergman space" ''Acta Math.'' , '''177''' (1996) pp. 275–310</td></tr><tr><td valign="top">[a2]</td> <td valign="top"> C. Apostol, H. Bercovici, C. Foias, C. Pearcy, "Invariant subspaces, dilation theory, and the structure of the predual of a dual algebra" ''J. Funct. Anal.'' , '''63''' (1985) pp. 369–404</td></tr><tr><td valign="top">[a3]</td> <td valign="top"> S. Axler, "Bergman spaces and their operators" J.B. Conway (ed.) B.B. Morrel (ed.) , ''Surveys of Some Recent Results in Operator Theory I'' , ''Res. Notes Math.'' , '''171''' , Pitman (1988) pp. 1–50</td></tr><tr><td valign="top">[a4]</td> <td valign="top"> S. Axler, D. Zheng, "Compact operators via the Berezin transform" ''Indiana J. Math.'' , '''49''' (1998) pp. 311</td></tr><tr><td valign="top">[a5]</td> <td valign="top"> "Holomorphic Spaces" S. Axler (ed.) J. McCarthy (ed.) D. Sarason (ed.) , Cambridge Univ. Press (1998)</td></tr><tr><td valign="top">[a6]</td> <td valign="top"> S. Bell, "The Cauchy transform, potential theory, and conformal mapping" , ''Studies Adv. Math.'' , CRC (1992)</td></tr><tr><td valign="top">[a7]</td> <td valign="top"> S. Bergman, "The kernel function and conformal mapping" , ''Math. Surveys'' , '''5''' , Amer. Math. Soc. (1950)</td></tr><tr><td valign="top">[a8]</td> <td valign="top"> L. Carleson, "Selected problems on exceptional sets" , v. Nostrand (1967)</td></tr><tr><td valign="top">[a9]</td> <td valign="top"> C. Cowen, B. MacCluer, "Composition operators on spaces of analytic functions" , ''Studies Adv. Math.'' , CRC (1995)</td></tr><tr><td valign="top">[a10]</td> <td valign="top"> P. Duren, D. Khavinson, H. Shapiro, C. Sundberg, "Invariant subspaces in Bergman spaces and the biharmonic equation" ''Michigan Math. J.'' , '''41''' (1994) pp. 247–259</td></tr><tr><td valign="top">[a11]</td> <td valign="top"> V.P. Havin, "Approximation in the mean by analytic functions" ''Soviet Math. Dokl.'' , '''9''' (1968) pp. 245–248</td></tr><tr><td valign="top">[a12]</td> <td valign="top"> L. Hedberg, "Non linear potentials and approximation in the mean by analytic functions" ''Math. Z.'' , '''129''' (1972) pp. 299–319</td></tr><tr><td valign="top">[a13]</td> <td valign="top"> H. Hedenmalm, "A factorization theorem for square area integrable functions" ''J. Reine Angew. Math.'' , '''422''' (1991) pp. 45–68</td></tr><tr><td valign="top">[a14]</td> <td valign="top"> H. Hedenmalm, "An invariant subspace of the Bergman space having the codimension two property" ''J. Reine Angew. Math.'' , '''443''' (1993) pp. 1–9</td></tr><tr><td valign="top">[a15]</td> <td valign="top"> B. Korenblum, "An extension of the Nevanlinna theory" ''Acta Math.'' , '''135''' (1975) pp. 187–219</td></tr><tr><td valign="top">[a16]</td> <td valign="top"> B. Korenblum, "A Beurling type theorem" ''Acta Math.'' , '''138''' (1977) pp. 265–293</td></tr><tr><td valign="top">[a17]</td> <td valign="top"> B. Korenblum, "Outer functions and cyclic elements in Bergman spaces" ''J. Funct. Anal.'' , '''115''' (1993) pp. 104–118</td></tr><tr><td valign="top">[a18]</td> <td valign="top"> H. Li, D. Luecking, "BMO on strongly pseudoconvex domains: Hankel operators, duality and $\partial$-estimates" ''Trans. Amer. Math. Soc.'' , '''346''' (1994) pp. 661–691</td></tr><tr><td valign="top">[a19]</td> <td valign="top"> K. Seip, "Beurling type density theorems in the unit disc" ''Invent. Math.'' , '''113''' (1993) pp. 21–39</td></tr><tr><td valign="top">[a20]</td> <td valign="top"> K. Seip, "On a theorem of Korenblum" ''Ark. Mat.'' , '''32''' (1994) pp. 237–243</td></tr><tr><td valign="top">[a21]</td> <td valign="top"> K. Seip, "On Korenblum's density condition for the zero sets of $A _ { p , \alpha }$" ''J. Anal. Math.'' , '''67''' (1995) pp. 307–322</td></tr><tr><td valign="top">[a22]</td> <td valign="top"> K. Zhu, "Operator theory in function spaces" ''Pure Appl. Math.'' , '''139''' (1990)</td></tr></table> |
$L _ { a } ^ { p } ( G )$, $0 < p < \infty$
Spaces of analytic functions $f$ on an open set $G$ in the complex plane for which
\begin{equation*} \| f \| _ { p , G } ^ { p } = \int_G | f ( z ) | ^ { p } d A ( z ) < \infty , \end{equation*}
where $d A ( z ) = d x d y$ is the $2$-dimensional Lebesgue measure on $G$.
If $p = 2$, then $L _ { a } ^ { 2 } ( G )$ is a Hilbert space and has a reproducing kernel $k _ { z } ( w )$ satisfying $f ( z ) = \int _ { G } f ( w ) \overline { k _ { z } ( w ) } d A ( w )$ for all $f$ in $L _ { a } ^ { 2 } ( G )$ and $z$ in $G$ (cf. also Bergman kernel function). The strong connections between reproducing kernels, conformal mappings (cf. also Conformal mapping), harmonic measure, and elliptic partial differential equations (cf. also Elliptic partial differential equation) motivated the pioneering work of S. Bergman [a7]; see also, e.g., [a6].
If $p > 0$, then $L _ { a } ^ { p } ( G )$ is a closed subspace of $L ^ { p } ( G )$; thus, for $p \geq 1$ it is a Banach space with norm $\| f \| _ { p , G}$. Furthermore, a function $f \in L ^ { p } ( G )$, $p \geq 1$, has an almost-everywhere representative in $L _ { a } ^ { p } ( G )$ if and only if
\begin{equation} \tag{a1} \int _ { G } f \overline { \partial } \varphi d A = 0 \end{equation}
for all compactly supported functions $\varphi$ in $C _ { c } ^ { \infty } ( G )$. It is a result of V. Havin that via the Bergman–Sobolev duality (a1) the annihilator of $L _ { a } ^ { p } ( G )$, $p > 1$, can be identified with the Sobolev space $W _ { 0 } ^ { q , 1 } ( G )$, $1 / p + 1 / q = 1$, [a11]. If $1 < p < 2$, then the space $L _ { a } ^ { p } ( G )$ is non-trivial if and only if $\mathbf{C} \backslash G$ contains at least two points. $L _ { a } ^ { 2 } ( G )$ is non-trivial if and only if $\mathbf{C} \backslash G$ has positive logarithmic capacity, and more generally, for $p > 2$, $L _ { a } ^ { p } ( G )$ is non-trivial if and only if $\mathbf{C} \backslash G$ has positive $q$-capacity, $1 / p + 1 / q = 1$, [a8], [a12].
If $G$ is bounded, then the Cauchy transform (cf. also Cauchy integral) provides many examples of functions in a Bergman space. Indeed, if $\mu$ is a finite complex measure with compact support in the complement of $G$ and if $p > 1$, then the Cauchy transform
\begin{equation*} C _ { \mu } ( z ) = \int \frac { 1 } { z - w } d \mu ( w ) \end{equation*}
is in $L _ { a } ^ { p } ( G )$ if and only if $\mu$ has finite $q$-energy, $1 / p + 1 / q = 1$, cf. also Energy of measures. In fact, such Cauchy transforms are dense in $L _ { a } ^ { p } ( G )$ ($G$ bounded), [a12].
When $G$ equals the unit disc $D = \{ z \in \mathbf{C} : | z | < 1 \}$, then one writes $L _ { a } ^ { p } ( G ) = L _ { a } ^ { p }$ or $A ^ { p }$. For each $p > 1$ one can identify the dual 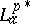 with $L _ { a } ^ { q }$, $1 / p + 1 / q = 1$, with the duality $\langle f , g \rangle = \int _ { D } f \overline{g} d A$ with equivalence of norms. One also has $B _ { 0 } ^ { * } \cong L _ { a } ^ { 1 }$ and $L _ { a } ^ { 1 * } \cong B$, where $B$ and $B_0$ are the Bloch and the little Bloch spaces (cf. also Bloch function). Furthermore, harmonic conjugation defines a bounded linear transformation on $L ^ { p }$ for all $p \geq 1$, i.e. for each $p \geq 1$ there is a $c > 0$ such that $\| \tilde { u } \| _ { p } \leq c \| u \| _ { p }$ for every harmonic function $u$ on $D$; here, $\tilde{u}$ is the harmonic conjugate of $u$, ([a3]).
with $L _ { a } ^ { q }$, $1 / p + 1 / q = 1$, with the duality $\langle f , g \rangle = \int _ { D } f \overline{g} d A$ with equivalence of norms. One also has $B _ { 0 } ^ { * } \cong L _ { a } ^ { 1 }$ and $L _ { a } ^ { 1 * } \cong B$, where $B$ and $B_0$ are the Bloch and the little Bloch spaces (cf. also Bloch function). Furthermore, harmonic conjugation defines a bounded linear transformation on $L ^ { p }$ for all $p \geq 1$, i.e. for each $p \geq 1$ there is a $c > 0$ such that $\| \tilde { u } \| _ { p } \leq c \| u \| _ { p }$ for every harmonic function $u$ on $D$; here, $\tilde{u}$ is the harmonic conjugate of $u$, ([a3]).
For the topological algebra $A ^ { - \infty } = \cup _ { p > 0 } L _ { a } ^ { p }$, B. Korenblum has established a theory that parallels the theory of Hardy spaces with regard to Riesz factorization and invariant subspaces (see [a15] [a16]).
For a fixed $p > 0$ one notes that the Hardy space $H ^ { p }$ is contained in  , and it is useful to contrast the function theory in
, and it is useful to contrast the function theory in 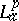 (as known today (1998)) with the classical situation in $H ^ { p }$. A general statement one can make is that results for functions in a Bergman space depend on the parameter $p > 0$.
(as known today (1998)) with the classical situation in $H ^ { p }$. A general statement one can make is that results for functions in a Bergman space depend on the parameter $p > 0$.
While a precise characterization of the 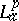 -zero sets is open (1998), certain asymptotic results are known, [a20], [a21]. Every function $f$ in
-zero sets is open (1998), certain asymptotic results are known, [a20], [a21]. Every function $f$ in 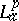 admits an "Lap-inner-Lap-outer" factorization $f = \varphi F$, [a1]. A function $\varphi$ in
admits an "Lap-inner-Lap-outer" factorization $f = \varphi F$, [a1]. A function $\varphi$ in 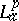 , $\| \varphi \| _ { p } = 1$, is called
, $\| \varphi \| _ { p } = 1$, is called 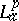 -inner if
-inner if
\begin{equation*} \int _ { D } z ^ { n } | \varphi ( z ) | ^ { p } d A ( z ) = 0 \end{equation*}
for all $n > 0$, and a function $F$ in  is called
is called 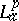 -outer if whenever $g$ is in
-outer if whenever $g$ is in 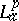 with $\|hF\|_p \geq \|hg\|_p$ for all analytic polynomials $h$, then $|F(0)|\geq |h(0)|$ (see [a17]).
with $\|hF\|_p \geq \|hg\|_p$ for all analytic polynomials $h$, then $|F(0)|\geq |h(0)|$ (see [a17]).  -outer functions are cyclic in
-outer functions are cyclic in 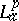 , yet, no explicit function-theoretic descriptions for either the inner or outer functions are known (1998). The inner factors are sometimes called the contractive divisors, because $\| f / \varphi \| _ { p } \leq \| f \| _ { p }$, whenever $f$ is in the invariant subspace generated by the
, yet, no explicit function-theoretic descriptions for either the inner or outer functions are known (1998). The inner factors are sometimes called the contractive divisors, because $\| f / \varphi \| _ { p } \leq \| f \| _ { p }$, whenever $f$ is in the invariant subspace generated by the 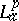 -inner function $\varphi$, [a10], [a13]. General invariant subspaces of
-inner function $\varphi$, [a10], [a13]. General invariant subspaces of  do not have to be cyclic (i.e. generated by one function) (see [a2], [a14]), but for $p = 2$ one knows that they are generated by a collection of inner functions, [a1].
do not have to be cyclic (i.e. generated by one function) (see [a2], [a14]), but for $p = 2$ one knows that they are generated by a collection of inner functions, [a1].
Interpolating sequences and sampling sequences for  have been characterized by K. Seip [a19].
have been characterized by K. Seip [a19].
There also are well-developed theories of Toeplitz, Hankel, and composition operators on Bergman spaces, and work on Bergman spaces on domains in $\mathbf{C} ^ { n }$ has started (see e.g. [a22], [a9], [a18], [a4], [a5]).
References
| [a1] | A. Aleman, S. Richter, C. Sundberg, "Beurling's theorem for the Bergman space" Acta Math. , 177 (1996) pp. 275–310 |
| [a2] | C. Apostol, H. Bercovici, C. Foias, C. Pearcy, "Invariant subspaces, dilation theory, and the structure of the predual of a dual algebra" J. Funct. Anal. , 63 (1985) pp. 369–404 |
| [a3] | S. Axler, "Bergman spaces and their operators" J.B. Conway (ed.) B.B. Morrel (ed.) , Surveys of Some Recent Results in Operator Theory I , Res. Notes Math. , 171 , Pitman (1988) pp. 1–50 |
| [a4] | S. Axler, D. Zheng, "Compact operators via the Berezin transform" Indiana J. Math. , 49 (1998) pp. 311 |
| [a5] | "Holomorphic Spaces" S. Axler (ed.) J. McCarthy (ed.) D. Sarason (ed.) , Cambridge Univ. Press (1998) |
| [a6] | S. Bell, "The Cauchy transform, potential theory, and conformal mapping" , Studies Adv. Math. , CRC (1992) |
| [a7] | S. Bergman, "The kernel function and conformal mapping" , Math. Surveys , 5 , Amer. Math. Soc. (1950) |
| [a8] | L. Carleson, "Selected problems on exceptional sets" , v. Nostrand (1967) |
| [a9] | C. Cowen, B. MacCluer, "Composition operators on spaces of analytic functions" , Studies Adv. Math. , CRC (1995) |
| [a10] | P. Duren, D. Khavinson, H. Shapiro, C. Sundberg, "Invariant subspaces in Bergman spaces and the biharmonic equation" Michigan Math. J. , 41 (1994) pp. 247–259 |
| [a11] | V.P. Havin, "Approximation in the mean by analytic functions" Soviet Math. Dokl. , 9 (1968) pp. 245–248 |
| [a12] | L. Hedberg, "Non linear potentials and approximation in the mean by analytic functions" Math. Z. , 129 (1972) pp. 299–319 |
| [a13] | H. Hedenmalm, "A factorization theorem for square area integrable functions" J. Reine Angew. Math. , 422 (1991) pp. 45–68 |
| [a14] | H. Hedenmalm, "An invariant subspace of the Bergman space having the codimension two property" J. Reine Angew. Math. , 443 (1993) pp. 1–9 |
| [a15] | B. Korenblum, "An extension of the Nevanlinna theory" Acta Math. , 135 (1975) pp. 187–219 |
| [a16] | B. Korenblum, "A Beurling type theorem" Acta Math. , 138 (1977) pp. 265–293 |
| [a17] | B. Korenblum, "Outer functions and cyclic elements in Bergman spaces" J. Funct. Anal. , 115 (1993) pp. 104–118 |
| [a18] | H. Li, D. Luecking, "BMO on strongly pseudoconvex domains: Hankel operators, duality and $\partial$-estimates" Trans. Amer. Math. Soc. , 346 (1994) pp. 661–691 |
| [a19] | K. Seip, "Beurling type density theorems in the unit disc" Invent. Math. , 113 (1993) pp. 21–39 |
| [a20] | K. Seip, "On a theorem of Korenblum" Ark. Mat. , 32 (1994) pp. 237–243 |
| [a21] | K. Seip, "On Korenblum's density condition for the zero sets of $A _ { p , \alpha }$" J. Anal. Math. , 67 (1995) pp. 307–322 |
| [a22] | K. Zhu, "Operator theory in function spaces" Pure Appl. Math. , 139 (1990) |
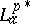 with $L _ { a } ^ { q }$, $1 / p + 1 / q = 1$, with the duality $\langle f , g \rangle = \int _ { D } f \overline{g} d A$ with equivalence of norms. One also has $B _ { 0 } ^ { * } \cong L _ { a } ^ { 1 }$ and $L _ { a } ^ { 1 * } \cong B$, where $B$ and $B_0$ are the Bloch and the little Bloch spaces (cf. also Bloch function). Furthermore, harmonic conjugation defines a bounded linear transformation on $L ^ { p }$ for all $p \geq 1$, i.e. for each $p \geq 1$ there is a $c > 0$ such that $\| \tilde { u } \| _ { p } \leq c \| u \| _ { p }$ for every harmonic function $u$ on $D$; here, $\tilde{u}$ is the harmonic conjugate of $u$, ([a3]).
with $L _ { a } ^ { q }$, $1 / p + 1 / q = 1$, with the duality $\langle f , g \rangle = \int _ { D } f \overline{g} d A$ with equivalence of norms. One also has $B _ { 0 } ^ { * } \cong L _ { a } ^ { 1 }$ and $L _ { a } ^ { 1 * } \cong B$, where $B$ and $B_0$ are the Bloch and the little Bloch spaces (cf. also Bloch function). Furthermore, harmonic conjugation defines a bounded linear transformation on $L ^ { p }$ for all $p \geq 1$, i.e. for each $p \geq 1$ there is a $c > 0$ such that $\| \tilde { u } \| _ { p } \leq c \| u \| _ { p }$ for every harmonic function $u$ on $D$; here, $\tilde{u}$ is the harmonic conjugate of $u$, ([a3]).
 , and it is useful to contrast the function theory in
, and it is useful to contrast the function theory in 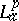 (as known today (1998)) with the classical situation in $H ^ { p }$. A general statement one can make is that results for functions in a Bergman space depend on the parameter $p > 0$.
(as known today (1998)) with the classical situation in $H ^ { p }$. A general statement one can make is that results for functions in a Bergman space depend on the parameter $p > 0$.
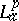 -zero sets is open (1998), certain asymptotic results are known, [a20], [a21]. Every function $f$ in
-zero sets is open (1998), certain asymptotic results are known, [a20], [a21]. Every function $f$ in 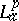 admits an "Lap-inner-Lap-outer" factorization $f = \varphi F$, [a1]. A function $\varphi$ in
admits an "Lap-inner-Lap-outer" factorization $f = \varphi F$, [a1]. A function $\varphi$ in 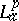 , $\| \varphi \| _ { p } = 1$, is called
, $\| \varphi \| _ { p } = 1$, is called 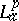 -inner if
-inner if
 is called
is called 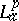 -outer if whenever $g$ is in
-outer if whenever $g$ is in 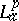 with $\|hF\|_p \geq \|hg\|_p$ for all analytic polynomials $h$, then $|F(0)|\geq |h(0)|$ (see [a17]).
with $\|hF\|_p \geq \|hg\|_p$ for all analytic polynomials $h$, then $|F(0)|\geq |h(0)|$ (see [a17]).  -outer functions are cyclic in
-outer functions are cyclic in 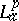 , yet, no explicit function-theoretic descriptions for either the inner or outer functions are known (1998). The inner factors are sometimes called the contractive divisors, because $\| f / \varphi \| _ { p } \leq \| f \| _ { p }$, whenever $f$ is in the invariant subspace generated by the
, yet, no explicit function-theoretic descriptions for either the inner or outer functions are known (1998). The inner factors are sometimes called the contractive divisors, because $\| f / \varphi \| _ { p } \leq \| f \| _ { p }$, whenever $f$ is in the invariant subspace generated by the 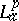 -inner function $\varphi$, [a10], [a13]. General invariant subspaces of
-inner function $\varphi$, [a10], [a13]. General invariant subspaces of  do not have to be cyclic (i.e. generated by one function) (see [a2], [a14]), but for $p = 2$ one knows that they are generated by a collection of inner functions, [a1].
do not have to be cyclic (i.e. generated by one function) (see [a2], [a14]), but for $p = 2$ one knows that they are generated by a collection of inner functions, [a1].
 have been characterized by K. Seip [a19].
have been characterized by K. Seip [a19].