Difference between revisions of "Buchstab function"
From Encyclopedia of Mathematics
(Importing text file) |
m (Richard Pinch moved page Bukhstab function to Buchstab function: More common term in the literature) |
(No difference)
| |
Revision as of 06:41, 22 September 2017
The continuous solution of the system
 |
 |
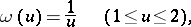This function occurs in number theory as the limit
 |
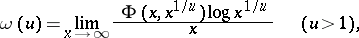where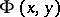 
denotes the number of positive integers not exceeding 
that are free of prime factors smaller than 
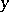; see [a1].
The function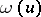 
is positive-valued and converges to the constant 
as 
, where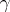 
is the Euler constant. The difference 
behaves asymptotically like a trigonometric function with period 
and decaying amplitudes of size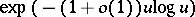 
. These and similar results have been exploited in the study of irregularities in the distribution of prime numbers; see [a2], [a3].
References
| [a1] | A.A. Bukhstab, "Asymptotic estimates of a general number-theoretic function" Mat. Sb. , 44 (1937) pp. 1239–1246 (In Russian) |
| [a2] | J. Friedlander, A. Granville, A. Hildebrand, H. Maier, "Oscillation theorems for primes in arithmetic progressions and for sifting functions" J. Amer. Math. Soc. , 4 (1991) pp. 25–86 |
| [a3] | H. Maier, "Primes in short intervals" Michigan Math. J. , 32 (1985) pp. 221–225 |
How to Cite This Entry:
Buchstab function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Buchstab_function&oldid=41917
Buchstab function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Buchstab_function&oldid=41917
This article was adapted from an original article by A. Hildebrand (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article