Difference between revisions of "Finite-dimensional representation"
(Importing text file) |
m (link) |
||
| Line 1: | Line 1: | ||
A linear [[Representation of a topological group|representation of a topological group]] in a finite-dimensional vector space. The theory of finite-dimensional representations is one of the most important and most developed parts of the representation theory of groups. An irreducible finite-dimensional representation is completely irreducible (see [[Schur lemma|Schur lemma]]), but an operator-irreducible finite-dimensional representation can be reducible. A measurable finite-dimensional representation of a locally compact group locally coincides almost-everywhere with a continuous finite-dimensional representation. A bounded finite-dimensional representation of a locally compact group is equivalent to a unitary representation. A locally compact group having a faithful finite-dimensional representation is a Lie group [[#References|[7]]]. | A linear [[Representation of a topological group|representation of a topological group]] in a finite-dimensional vector space. The theory of finite-dimensional representations is one of the most important and most developed parts of the representation theory of groups. An irreducible finite-dimensional representation is completely irreducible (see [[Schur lemma|Schur lemma]]), but an operator-irreducible finite-dimensional representation can be reducible. A measurable finite-dimensional representation of a locally compact group locally coincides almost-everywhere with a continuous finite-dimensional representation. A bounded finite-dimensional representation of a locally compact group is equivalent to a unitary representation. A locally compact group having a faithful finite-dimensional representation is a Lie group [[#References|[7]]]. | ||
| − | A unitary finite-dimensional representation is a direct sum of irreducible unitary finite-dimensional representations. The intersection of the kernels of the continuous homomorphisms of a topological group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402501.png" /> coincides with the intersection of the kernels of the irreducible unitary finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402502.png" />; if this set contains only the identity of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402503.png" />, then there is a continuous monomorphism from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402504.png" /> into some compact group, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402505.png" /> is said to be imbeddable in a compact group, or to be a maximally almost-periodic group (a MAP-group). If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402506.png" /> is a MAP-group, then the family of matrix entries of irreducible finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402507.png" /> separates points in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402508.png" />. Commutative groups and compact groups are MAP-groups; a connected locally compact group is a MAP-group if and only if it is the direct product of a connected compact group and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402509.png" /> (see [[#References|[5]]]). A MAP-group can have infinite-dimensional irreducible unitary representations and need not be a group of type 1. Every continuous irreducible unitary representation of a locally compact group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025010.png" /> is finite-dimensional if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025011.png" /> is a projective limit of finite extensions of groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025012.png" /> of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025013.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025014.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025015.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025016.png" /> are closed subgroups of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025017.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025018.png" /> is isomorphic to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025019.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025020.png" /> is compact and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025021.png" /> is a discrete group that is central in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025022.png" /> [[#References|[8]]]; a sufficient condition is that the quotient group of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025023.png" /> by its centre be compact. Moreover, for many locally compact groups (in particular, for non-compact simple Lie groups), the only irreducible unitary finite-dimensional representation is the trivial representation. | + | A unitary finite-dimensional representation is a direct sum of irreducible unitary finite-dimensional representations. The intersection of the kernels of the continuous homomorphisms of a topological group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402501.png" /> coincides with the intersection of the kernels of the irreducible unitary finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402502.png" />; if this set contains only the identity of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402503.png" />, then there is a continuous monomorphism from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402504.png" /> into some compact group, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402505.png" /> is said to be imbeddable in a compact group, or to be a maximally almost-periodic group (a MAP-group). If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402506.png" /> is a MAP-group, then the family of matrix entries of irreducible finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402507.png" /> separates points in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402508.png" />. Commutative groups and compact groups are MAP-groups; a connected locally compact group is a MAP-group if and only if it is the direct product of a connected compact group and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f0402509.png" /> (see [[#References|[5]]]). A MAP-group can have infinite-dimensional irreducible unitary representations and need not be a group of type 1. Every continuous irreducible unitary representation of a locally compact group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025010.png" /> is finite-dimensional if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025011.png" /> is a projective limit of finite extensions of groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025012.png" /> of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025013.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025014.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025015.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025016.png" /> are closed subgroups of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025017.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025018.png" /> is isomorphic to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025019.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025020.png" /> is compact and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025021.png" /> is a discrete group that is central in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025022.png" /> [[#References|[8]]]; a sufficient condition is that the quotient group of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025023.png" /> by its centre be compact. Moreover, for many locally compact groups (in particular, for non-compact simple Lie groups), the only irreducible unitary finite-dimensional representation is the [[trivial representation]]. |
Non-unitary finite-dimensional representations of topological groups have been classified (up to equivalence) only for special groups; in particular, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025024.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025025.png" />, where the problem of describing finite-dimensional representations is solved by reducing matrices to Jordan form, and — in the case of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025026.png" /> — is related to the theory of ordinary differential equations with constant coefficients, in that the solution space of such an equation is a finite-dimensional invariant subspace of the regular representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025027.png" /> in the space of continuous functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025028.png" />. In addition, the finite-dimensional representations of connected semi-simple Lie groups are known. More precisely, these are direct sums of irreducible finite-dimensional representations that can be described as follows. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025029.png" /> is a semi-simple complex Lie group and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025030.png" /> is a maximal compact subgroup, then every continuous irreducible unitary representation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025031.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025032.png" /> in a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025033.png" /> can be extended: 1) to an irreducible representation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025034.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025035.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025036.png" /> whose matrix entries are analytic functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025037.png" />; and 2) to an irreducible representation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025038.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025039.png" /> whose matrix entries are complex conjugates of analytic functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025040.png" />; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025041.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025042.png" /> are determined uniquely by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025043.png" />. The tensor product <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025044.png" /> is an irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025045.png" /> for arbitrary irreducible unitary finite-dimensional representations <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025046.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025047.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025048.png" />, and every irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025049.png" /> is equivalent to a representation of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025050.png" />. A description of the finite-dimensional representations of a simply-connected connected complex semi-simple Lie group can also be given in terms of the exponentials of finite-dimensional representations of its Lie algebra, and also by using the [[Gauss decomposition|Gauss decomposition]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025051.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025052.png" />: Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025053.png" /> be a continuous function on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025054.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025055.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025056.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025057.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025058.png" />, and suppose that the linear hull <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025059.png" /> of the functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025060.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025061.png" />, is finite-dimensional; then the formula <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025062.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025063.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025064.png" />, defines an irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025065.png" />, and all irreducible finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025066.png" /> can be obtained in this way. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025067.png" /> is a real semi-simple Lie group having complex form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025068.png" />, then every irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025069.png" /> is the restriction to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025070.png" /> of some unique irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025071.png" /> whose matrix entries are analytic on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025072.png" /> (so that the theory of finite-dimensional representations of semi-simple connected Lie groups reduces essentially to that of compact Lie groups). A finite-dimensional representation of an arbitrary Lie group is real analytic; if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025073.png" /> is a simply-connected Lie group, then there is a one-to-one correspondence between the finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025074.png" /> and of its Lie algebras (associating to a representation of the Lie group its differential; the inverse mapping associates to a representation of the Lie algebra its exponential). However, the classification of finite-dimensional representations of arbitrary Lie groups is far from being completely solved (1988), even for the special cases of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025075.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025076.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025077.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025078.png" /> (see [[#References|[6]]]). On the other hand, the irreducible finite-dimensional representations <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025079.png" /> of a connected Lie group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025080.png" /> are known [[#References|[2]]]: They have the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025081.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025082.png" /> is a one-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025083.png" /> (that is, essentially of its commutative quotient group by the commutator subgroup), and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025084.png" /> is a finite-dimensional representation of the semi-simple quotient group of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025085.png" /> by the maximal connected solvable normal subgroup of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025086.png" /> (see [[Levi–Mal'tsev decomposition|Levi–Mal'tsev decomposition]]). | Non-unitary finite-dimensional representations of topological groups have been classified (up to equivalence) only for special groups; in particular, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025024.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025025.png" />, where the problem of describing finite-dimensional representations is solved by reducing matrices to Jordan form, and — in the case of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025026.png" /> — is related to the theory of ordinary differential equations with constant coefficients, in that the solution space of such an equation is a finite-dimensional invariant subspace of the regular representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025027.png" /> in the space of continuous functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025028.png" />. In addition, the finite-dimensional representations of connected semi-simple Lie groups are known. More precisely, these are direct sums of irreducible finite-dimensional representations that can be described as follows. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025029.png" /> is a semi-simple complex Lie group and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025030.png" /> is a maximal compact subgroup, then every continuous irreducible unitary representation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025031.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025032.png" /> in a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025033.png" /> can be extended: 1) to an irreducible representation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025034.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025035.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025036.png" /> whose matrix entries are analytic functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025037.png" />; and 2) to an irreducible representation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025038.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025039.png" /> whose matrix entries are complex conjugates of analytic functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025040.png" />; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025041.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025042.png" /> are determined uniquely by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025043.png" />. The tensor product <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025044.png" /> is an irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025045.png" /> for arbitrary irreducible unitary finite-dimensional representations <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025046.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025047.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025048.png" />, and every irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025049.png" /> is equivalent to a representation of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025050.png" />. A description of the finite-dimensional representations of a simply-connected connected complex semi-simple Lie group can also be given in terms of the exponentials of finite-dimensional representations of its Lie algebra, and also by using the [[Gauss decomposition|Gauss decomposition]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025051.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025052.png" />: Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025053.png" /> be a continuous function on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025054.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025055.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025056.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025057.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025058.png" />, and suppose that the linear hull <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025059.png" /> of the functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025060.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025061.png" />, is finite-dimensional; then the formula <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025062.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025063.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025064.png" />, defines an irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025065.png" />, and all irreducible finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025066.png" /> can be obtained in this way. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025067.png" /> is a real semi-simple Lie group having complex form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025068.png" />, then every irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025069.png" /> is the restriction to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025070.png" /> of some unique irreducible finite-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025071.png" /> whose matrix entries are analytic on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025072.png" /> (so that the theory of finite-dimensional representations of semi-simple connected Lie groups reduces essentially to that of compact Lie groups). A finite-dimensional representation of an arbitrary Lie group is real analytic; if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025073.png" /> is a simply-connected Lie group, then there is a one-to-one correspondence between the finite-dimensional representations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025074.png" /> and of its Lie algebras (associating to a representation of the Lie group its differential; the inverse mapping associates to a representation of the Lie algebra its exponential). However, the classification of finite-dimensional representations of arbitrary Lie groups is far from being completely solved (1988), even for the special cases of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025075.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025076.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025077.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025078.png" /> (see [[#References|[6]]]). On the other hand, the irreducible finite-dimensional representations <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025079.png" /> of a connected Lie group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025080.png" /> are known [[#References|[2]]]: They have the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025081.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025082.png" /> is a one-dimensional representation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025083.png" /> (that is, essentially of its commutative quotient group by the commutator subgroup), and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025084.png" /> is a finite-dimensional representation of the semi-simple quotient group of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025085.png" /> by the maximal connected solvable normal subgroup of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040250/f04025086.png" /> (see [[Levi–Mal'tsev decomposition|Levi–Mal'tsev decomposition]]). | ||
Revision as of 18:24, 30 November 2016
A linear representation of a topological group in a finite-dimensional vector space. The theory of finite-dimensional representations is one of the most important and most developed parts of the representation theory of groups. An irreducible finite-dimensional representation is completely irreducible (see Schur lemma), but an operator-irreducible finite-dimensional representation can be reducible. A measurable finite-dimensional representation of a locally compact group locally coincides almost-everywhere with a continuous finite-dimensional representation. A bounded finite-dimensional representation of a locally compact group is equivalent to a unitary representation. A locally compact group having a faithful finite-dimensional representation is a Lie group [7].
A unitary finite-dimensional representation is a direct sum of irreducible unitary finite-dimensional representations. The intersection of the kernels of the continuous homomorphisms of a topological group 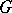 coincides with the intersection of the kernels of the irreducible unitary finite-dimensional representations of
coincides with the intersection of the kernels of the irreducible unitary finite-dimensional representations of 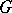 ; if this set contains only the identity of
; if this set contains only the identity of 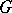 , then there is a continuous monomorphism from
, then there is a continuous monomorphism from  into some compact group, and
into some compact group, and  is said to be imbeddable in a compact group, or to be a maximally almost-periodic group (a MAP-group). If
is said to be imbeddable in a compact group, or to be a maximally almost-periodic group (a MAP-group). If 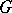 is a MAP-group, then the family of matrix entries of irreducible finite-dimensional representations of
is a MAP-group, then the family of matrix entries of irreducible finite-dimensional representations of 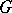 separates points in
separates points in 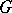 . Commutative groups and compact groups are MAP-groups; a connected locally compact group is a MAP-group if and only if it is the direct product of a connected compact group and
. Commutative groups and compact groups are MAP-groups; a connected locally compact group is a MAP-group if and only if it is the direct product of a connected compact group and 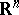 (see [5]). A MAP-group can have infinite-dimensional irreducible unitary representations and need not be a group of type 1. Every continuous irreducible unitary representation of a locally compact group
(see [5]). A MAP-group can have infinite-dimensional irreducible unitary representations and need not be a group of type 1. Every continuous irreducible unitary representation of a locally compact group 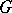 is finite-dimensional if and only if
is finite-dimensional if and only if  is a projective limit of finite extensions of groups
is a projective limit of finite extensions of groups 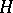 of the form
of the form 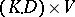 , where
, where  ,
,  and
and 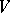 are closed subgroups of
are closed subgroups of 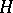 such that
such that 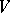 is isomorphic to
is isomorphic to 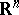 ,
,  is compact and
is compact and  is a discrete group that is central in
is a discrete group that is central in 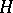 [8]; a sufficient condition is that the quotient group of
[8]; a sufficient condition is that the quotient group of 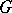 by its centre be compact. Moreover, for many locally compact groups (in particular, for non-compact simple Lie groups), the only irreducible unitary finite-dimensional representation is the trivial representation.
by its centre be compact. Moreover, for many locally compact groups (in particular, for non-compact simple Lie groups), the only irreducible unitary finite-dimensional representation is the trivial representation.
Non-unitary finite-dimensional representations of topological groups have been classified (up to equivalence) only for special groups; in particular, for 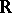 and
and 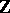 , where the problem of describing finite-dimensional representations is solved by reducing matrices to Jordan form, and — in the case of
, where the problem of describing finite-dimensional representations is solved by reducing matrices to Jordan form, and — in the case of 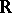 — is related to the theory of ordinary differential equations with constant coefficients, in that the solution space of such an equation is a finite-dimensional invariant subspace of the regular representation of
— is related to the theory of ordinary differential equations with constant coefficients, in that the solution space of such an equation is a finite-dimensional invariant subspace of the regular representation of 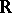 in the space of continuous functions on
in the space of continuous functions on 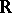 . In addition, the finite-dimensional representations of connected semi-simple Lie groups are known. More precisely, these are direct sums of irreducible finite-dimensional representations that can be described as follows. If
. In addition, the finite-dimensional representations of connected semi-simple Lie groups are known. More precisely, these are direct sums of irreducible finite-dimensional representations that can be described as follows. If 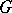 is a semi-simple complex Lie group and
is a semi-simple complex Lie group and 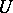 is a maximal compact subgroup, then every continuous irreducible unitary representation
is a maximal compact subgroup, then every continuous irreducible unitary representation 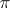 of
of 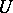 in a space
in a space  can be extended: 1) to an irreducible representation
can be extended: 1) to an irreducible representation 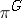 of
of  in
in 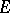 whose matrix entries are analytic functions on
whose matrix entries are analytic functions on 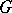 ; and 2) to an irreducible representation
; and 2) to an irreducible representation 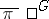 of
of 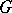 whose matrix entries are complex conjugates of analytic functions on
whose matrix entries are complex conjugates of analytic functions on 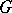 ;
; 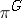 and
and  are determined uniquely by
are determined uniquely by 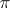 . The tensor product
. The tensor product 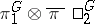 is an irreducible finite-dimensional representation of
is an irreducible finite-dimensional representation of 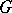 for arbitrary irreducible unitary finite-dimensional representations
for arbitrary irreducible unitary finite-dimensional representations 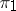 and
and 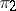 of
of 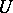 , and every irreducible finite-dimensional representation of
, and every irreducible finite-dimensional representation of 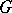 is equivalent to a representation of the form
is equivalent to a representation of the form 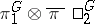 . A description of the finite-dimensional representations of a simply-connected connected complex semi-simple Lie group can also be given in terms of the exponentials of finite-dimensional representations of its Lie algebra, and also by using the Gauss decomposition
. A description of the finite-dimensional representations of a simply-connected connected complex semi-simple Lie group can also be given in terms of the exponentials of finite-dimensional representations of its Lie algebra, and also by using the Gauss decomposition 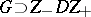 of
of 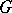 : Let
: Let 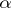 be a continuous function on
be a continuous function on  such that
such that 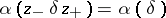 for all
for all  ,
, 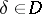 ,
, 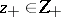 , and suppose that the linear hull
, and suppose that the linear hull 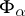 of the functions
of the functions 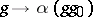 ,
, 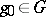 , is finite-dimensional; then the formula
, is finite-dimensional; then the formula 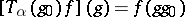 ,
, 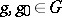 ,
, 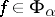 , defines an irreducible finite-dimensional representation of
, defines an irreducible finite-dimensional representation of 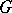 , and all irreducible finite-dimensional representations of
, and all irreducible finite-dimensional representations of 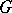 can be obtained in this way. If
can be obtained in this way. If  is a real semi-simple Lie group having complex form
is a real semi-simple Lie group having complex form 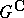 , then every irreducible finite-dimensional representation of
, then every irreducible finite-dimensional representation of  is the restriction to
is the restriction to 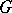 of some unique irreducible finite-dimensional representation of
of some unique irreducible finite-dimensional representation of 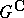 whose matrix entries are analytic on
whose matrix entries are analytic on 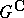 (so that the theory of finite-dimensional representations of semi-simple connected Lie groups reduces essentially to that of compact Lie groups). A finite-dimensional representation of an arbitrary Lie group is real analytic; if
(so that the theory of finite-dimensional representations of semi-simple connected Lie groups reduces essentially to that of compact Lie groups). A finite-dimensional representation of an arbitrary Lie group is real analytic; if  is a simply-connected Lie group, then there is a one-to-one correspondence between the finite-dimensional representations of
is a simply-connected Lie group, then there is a one-to-one correspondence between the finite-dimensional representations of 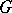 and of its Lie algebras (associating to a representation of the Lie group its differential; the inverse mapping associates to a representation of the Lie algebra its exponential). However, the classification of finite-dimensional representations of arbitrary Lie groups is far from being completely solved (1988), even for the special cases of
and of its Lie algebras (associating to a representation of the Lie group its differential; the inverse mapping associates to a representation of the Lie algebra its exponential). However, the classification of finite-dimensional representations of arbitrary Lie groups is far from being completely solved (1988), even for the special cases of 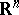 ,
, 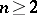 , and
, and 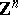 ,
, 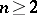 (see [6]). On the other hand, the irreducible finite-dimensional representations
(see [6]). On the other hand, the irreducible finite-dimensional representations 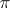 of a connected Lie group
of a connected Lie group 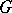 are known [2]: They have the form
are known [2]: They have the form 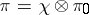 , where
, where 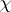 is a one-dimensional representation of
is a one-dimensional representation of  (that is, essentially of its commutative quotient group by the commutator subgroup), and
(that is, essentially of its commutative quotient group by the commutator subgroup), and 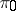 is a finite-dimensional representation of the semi-simple quotient group of
is a finite-dimensional representation of the semi-simple quotient group of 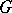 by the maximal connected solvable normal subgroup of
by the maximal connected solvable normal subgroup of 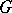 (see Levi–Mal'tsev decomposition).
(see Levi–Mal'tsev decomposition).
References
| [1] | A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian) |
| [2] | D.P. Zhelobenko, "Compact Lie groups and representations" , Amer. Math. Soc. (1973) (Translated from Russian) |
| [3] | M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian) |
| [4] | J. Dixmier, " algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
| [5] | A. Weil, "l'Intégration dans les groupes topologiques et ses applications" , Hermann (1940) |
| [6] | I.M. Gel'fand, V.A. Ponomarev, "Remarks on the classification of a pair of commuting linear transformations in a finite-dimensional space" Funct. Anal. Appl. , 3 : 4 (1969) pp. 325–326 Funktsional. Anal. i Prilozhen. , 3 : 4 (1969) pp. 81–82 |
| [7] | V.M. Glushkov, "The structure of locally compact groups and Hilbert's fifth problem" Transl. Amer. Math. Soc. , 15 (1960) pp. 55–93 Uspekhi Mat. Nauk , 12 : 2 (1957) pp. 3–41 |
| [8] | A.I. Shtern, "Locally bicompact groups with finite-dimensional irreducible representations" Math. USSR Sb. , 19 : 1 (1973) pp. 85–94 Mat. Sb. , 90 : 1 (1973) pp. 86–95 |
Comments
References
| [a1] | J.E. Humphreys, "Introduction to Lie algebras and representation theory" , Springer (1972) |
Finite-dimensional representation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Finite-dimensional_representation&oldid=39848