Difference between revisions of "D'Alembert operator"
From Encyclopedia of Mathematics
(Importing text file) |
m (→Comments: link) |
||
| Line 26: | Line 26: | ||
====Comments==== | ====Comments==== | ||
| − | In the last equation above, the Einstein | + | In the last equation above, the Einstein [[summation convention]] applies to the right-hand side (i.e. there is a summation involved over all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030080/d03008011.png" />). |
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , '''2''' , Interscience (1965) (Translated from German)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> F. John, "Partial differential equations" , Springer (1968)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , '''2''' , Interscience (1965) (Translated from German)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> F. John, "Partial differential equations" , Springer (1968)</TD></TR></table> | ||
Revision as of 19:13, 21 January 2016
wave operator, d'Alembertian
The second-order differential operator which in Cartesian coordinates assumes the following form:
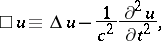 |
where  is the Laplace operator and
is the Laplace operator and  is a constant. Its form in spherical coordinates is:
is a constant. Its form in spherical coordinates is:
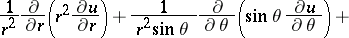 |
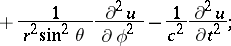 |
in cylindrical coordinates:
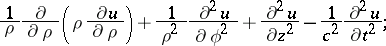 |
in general curvilinear coordinates:
 |
where  is the determinant of the matrix
is the determinant of the matrix  formed from the coefficients of the metric tensor
formed from the coefficients of the metric tensor  .
.
Named after J. d'Alembert (1747), who considered its simplest form when solving the one-dimensional wave equation.
Comments
In the last equation above, the Einstein summation convention applies to the right-hand side (i.e. there is a summation involved over all  ).
).
References
| [a1] | R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) |
| [a2] | F. John, "Partial differential equations" , Springer (1968) |
How to Cite This Entry:
D'Alembert operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=D%27Alembert_operator&oldid=37604
D'Alembert operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=D%27Alembert_operator&oldid=37604
This article was adapted from an original article by A.B. Ivanov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article