Difference between revisions of "Lie algebra, free"
(Importing text file) |
m (→Comments: link) |
||
| Line 17: | Line 17: | ||
for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841034.png" />. Friedrichs' theorem says for the case of finite <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841035.png" /> that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841036.png" /> belongs to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841037.png" /> if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841038.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841039.png" /> is the homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841040.png" /> defined by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841041.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841042.png" />. | for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841034.png" />. Friedrichs' theorem says for the case of finite <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841035.png" /> that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841036.png" /> belongs to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841037.png" /> if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841038.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841039.png" /> is the homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841040.png" /> defined by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841041.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l058/l058410/l05841042.png" />. | ||
| − | Free Lie algebras are the best context for the formulation of the Campbell–Baker–Hausdorff formula in its most general form. | + | Free Lie algebras are the best context for the formulation of the [[Campbell–Baker–Hausdorff]] formula in its most general form. |
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N. Jacobson, "Lie algebras" , Interscience (1962) ((also: Dover, reprint, 1979))</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N. Jacobson, "Lie algebras" , Interscience (1962) ((also: Dover, reprint, 1979))</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French)</TD></TR></table> | ||
Revision as of 12:25, 6 December 2015
over a ring 
A Lie algebra 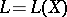 over
over 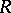 in which one can distinguish a free generating set
in which one can distinguish a free generating set  , a mapping from which into an arbitrary algebra
, a mapping from which into an arbitrary algebra 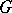 over
over 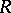 can be be extended to a homomorphism from
can be be extended to a homomorphism from  into
into  . The cardinality of
. The cardinality of 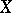 completely determines
completely determines 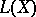 and is called its rank. A free Lie algebra is a free
and is called its rank. A free Lie algebra is a free  -module (for bases of it see Basic commutator). A subalgebra
-module (for bases of it see Basic commutator). A subalgebra  of a free Lie algebra over a field is itself a free Lie algebra (Shirshov's theorem, [1]). If
of a free Lie algebra over a field is itself a free Lie algebra (Shirshov's theorem, [1]). If 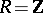 , then this is true only under the condition that
, then this is true only under the condition that  is a free Abelian group [2]. The finitely-generated subalgebras of a free Lie algebra over a field form a sublattice of the lattice of all subalgebras [3]. W. Magnus [4] established canonical connections between free Lie algebras and both free groups and free associative algebras.
is a free Abelian group [2]. The finitely-generated subalgebras of a free Lie algebra over a field form a sublattice of the lattice of all subalgebras [3]. W. Magnus [4] established canonical connections between free Lie algebras and both free groups and free associative algebras.
References
| [1] | A.I. Shirshov, "Subalgebras of free Lie algebras" Mat. Sb. , 33 : 2 (1953) pp. 441–452 (In Russian) |
| [2] | E. Witt, "Die Unterringe der freien Lieschen Ringe" Math. Z. , 64 (1956) pp. 195–216 |
| [3] | G.P. Kukin, "Intersection of subalgebras of a free Lie algebra" Algebra and Logic , 16 (1977) pp. 387–394 Algebra i Logika , 16 (1977) pp. 577–587 |
| [4] | W. Magnus, "Ueber Beziehungen zwischen höheren Kommutatoren" J. Reine Angew. Math. , 177 (1937) pp. 105–115 |
| [5] | Yu.A. Bakhturin, "Identical relations in Lie algebras" , VNU , Utrecht (1987) (Translated from Russian) |
Comments
To construct  one can start from the free associative algebra
one can start from the free associative algebra 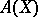 generated by
generated by  , which is made into a Lie algebra
, which is made into a Lie algebra 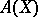 by taking as Lie product
by taking as Lie product  . Then
. Then 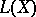 is the Lie subalgebra of
is the Lie subalgebra of  generated by
generated by 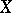 , and
, and 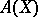 is the universal enveloping algebra of
is the universal enveloping algebra of 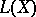 .
.
In case 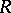 is a field of characteristic 0, more precise results on which elements of
is a field of characteristic 0, more precise results on which elements of  belong to
belong to  are given by the Specht–Wever theorem and the Friedrichs theorem, respectively. The first one says that a homogeneous element
are given by the Specht–Wever theorem and the Friedrichs theorem, respectively. The first one says that a homogeneous element  of degree
of degree 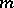 belongs to
belongs to  if and only if
if and only if  , where
, where  is the linear mapping defined by
is the linear mapping defined by
 |
for 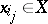 . Friedrichs' theorem says for the case of finite
. Friedrichs' theorem says for the case of finite 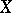 that
that  belongs to
belongs to 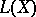 if and only if
if and only if 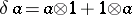 , where
, where 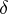 is the homomorphism
is the homomorphism 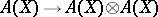 defined by
defined by 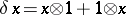 for
for 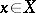 .
.
Free Lie algebras are the best context for the formulation of the Campbell–Baker–Hausdorff formula in its most general form.
References
| [a1] | N. Jacobson, "Lie algebras" , Interscience (1962) ((also: Dover, reprint, 1979)) |
| [a2] | N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) |
Lie algebra, free. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_algebra,_free&oldid=36854