Resolvent

A resolvent of an algebraic equation 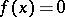of degree is an algebraic equation 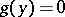, with coefficients that rationally depend on the coefficients of 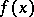, such that, if the roots of this equation are known, the roots of the given equation 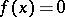can be found by solving simpler equations of degrees not exceeding . A rational expression itself is sometimes called a resolvent.

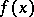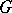Let be a separable polynomial over a field with Galois group , and let be a normal subgroup of . Let 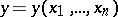be a rational expression in that remains invariant under all permutations of the roots belonging to , and let 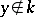. Then 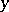is a root of some equation 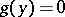with coefficients from , the Galois group of which is a proper quotient group of 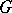. Thus, solving the equation 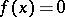reduces to solving the equation 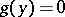and solving the equation 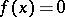over the field .

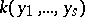For example, in order to solve an equation of degree :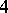

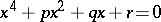(every equation of degree 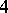is reducible to this form), the following cubic resolvent is used:

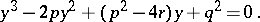Its roots 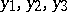are related to the roots 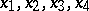by the relations 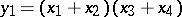, , 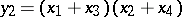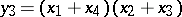. The roots 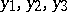are determined by the Cardano formula, which also makes it possible to determine .

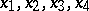Successive application of the resolvent method permits one to solve any equation with a solvable Galois group by reduction to solving a chain of equations with cyclic Galois groups. Lagrange's resolvent is used in solving the latter.

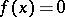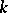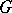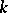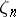Let be an equation over a field with a cyclic Galois group of order , and let contain a primitive -th root of unity . For an element 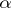which belongs to the splitting field of the polynomial (cf. Splitting field of a polynomial), and for a character from 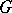into the group of -th roots of unity, Lagrange's resolvent 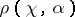is defined by the formula:

(*)

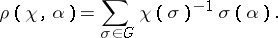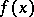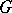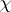Let be one of the roots of the polynomial and let run through the characters of . Then for the system of linear equations (*) the roots can be determined if the Lagrange resolvents are known for all characters of .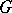

For 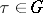the relation

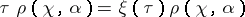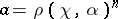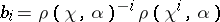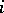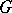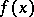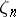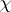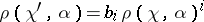is fulfilled, showing that the elements and , for any integer , are invariant under and are therefore uniquely defined rational expressions in the coefficients of the polynomial and the root . If generates the group of characters of , then the following equalities hold: and for .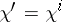

Any algebraic equation 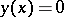that is irreducible over a given field (see Galois theory) and that is such that as a result of the adjunction of one of its roots to this field a field is obtained that contains all roots of the equation 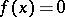, is called a Galois resolvent of .

References

[1]	B.L. van der Waerden, "Algebra" , 1–2 , Springer (1967–1971) (Translated from German)

L.V. Kuz'min

The resolvent (resolvent kernel) of an integral equation

(**)

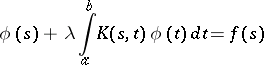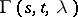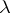is understood to be a function of the variables and the parameter with the aid of which the solution of equation (**) can be represented in the form:

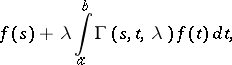provided 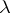is not an eigenvalue of (**). For example, for the kernel 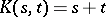the resolvent is the function

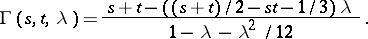BSE-3

The resolvent of an operator 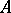is an operator 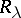inverse to . Here 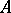is a closed linear operator defined on a dense set 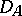of a Banach space 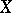with values in the same space and 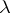is such that 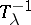is a continuous linear operator on 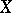. The points for which the resolvent exists are called regular points of , and the collection of all regular points is the resolvent set of this operator. The set 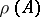is open and on each of its connected components the operator 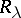is an analytic function of the parameter .

Properties of a resolvent are:

1) for any two points ;

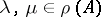2) 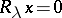implies ;

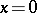3) if 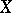is a Hilbert space, then .

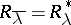References

[1]	K. Yosida, "Functional analysis" , Springer (1980)
[2]	N.I. [N.I. Akhiezer] Achieser, I.M. [I.M. Glaz'man] Glasman, "Theorie der linearen Operatoren im Hilbert Raum" , Akademie Verlag (1954) (Translated from Russian)
[3]	L.V. Kantorovich, G.P. Akilov, "Functional analysis in normed spaces" , Pergamon (1964) (Translated from Russian)