Difference between revisions of "Siegel theorem"
m (section headings) |
|||
| Line 1: | Line 1: | ||
===Siegel's theorem on Dirichlet L-functions=== | ===Siegel's theorem on Dirichlet L-functions=== | ||
| − | For any | + | For any $\epsilon > 0$ there exists a $c = c(\epsilon) > 0$ such that for any non-principal real [[Dirichlet character|Dirichlet character]] $\chi$ of modulus $k$, |
| − | + | $$ | |
| − | + | L(1,\chi) > \frac{c(\epsilon)}{k^\epsilon} \ . | |
| − | + | $$ | |
| − | First proved by C.L. Siegel [[#References|[1]]]. An equivalent formulation is concerned with real zeros of | + | First proved by C.L. Siegel [[#References|[1]]]. An equivalent formulation is concerned with real zeros of L-functions: For any $\epsilon > 0$ there exists a $c_1 = c_1(\epsilon) > 0$ such that $L(z,\chi) > c_1(\epsilon) \neq 0$ for $z > 1 - c_1/k^\epsilon$ for any non-principal real Dirichlet character $\chi$. The constants $c(\epsilon)$ and $c_1(\epsilon)$ are non-effective, in the sense that for no $0 < \epsilon < 1/2$ there is a way to estimate them from below. For this reason, applications of Siegel's theorem have a non-constructive character. For example, if $h(-D)$ is the divisor class number of a quadratic field of discriminant $-D$, it follows from the theorem that |
| − | + | $$ | |
| − | + | h(-D) > c_2(\epsilon) D^{1/2-\epsilon} | |
| − | + | $$ | |
| − | with a non-effective constant | + | with a non-effective constant $c_2(\epsilon)$ for $\epsilon < 1/2$. Similarly, the following estimate, which is uniform for $1 \le k \le \log x$, $(k,l) = 1$, |
| − | + | $$ | |
| − | + | \pi(x;k,l) - \frac{1}{\phi(k)} \int_2^x \frac{dt}{\log t} = O\left({ x e^{-c_3 \sqrt{\log x}} }\right) | |
| − | + | $$ | |
| − | where | + | where $\pi(x;k,l)$ is the number of primes $< x$ representable as $kn + l$, involves a non-effective constant $c_3$ (cf. also [[Distribution of prime numbers|Distribution of prime numbers]]). |
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> C.L. Siegel, "Ueber die Klassenzahl quadratischen Zahlkorper" ''Acta Arithmetica'' , '''1''' (1935) pp. 83–86</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> H. Davenport, "Multiplicative number theory" , Springer (1981)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A.A. Karatsuba, "Fundamentals of analytic number theory" , Moscow (1975) pp. Chapt. 9 (In Russian)</TD></TR></table> | + | <table> |
| + | <TR><TD valign="top">[1]</TD> <TD valign="top"> C.L. Siegel, "Ueber die Klassenzahl quadratischen Zahlkorper" ''Acta Arithmetica'' , '''1''' (1935) pp. 83–86</TD></TR> | ||
| + | <TR><TD valign="top">[2]</TD> <TD valign="top"> H. Davenport, "Multiplicative number theory" , Springer (1981)</TD></TR> | ||
| + | <TR><TD valign="top">[3]</TD> <TD valign="top"> A.A. Karatsuba, "Fundamentals of analytic number theory" , Moscow (1975) pp. Chapt. 9 (In Russian)</TD></TR> | ||
| + | </table> | ||
''S.M. Voronin'' | ''S.M. Voronin'' | ||
Revision as of 20:32, 18 October 2014
Siegel's theorem on Dirichlet L-functions
For any $\epsilon > 0$ there exists a $c = c(\epsilon) > 0$ such that for any non-principal real Dirichlet character $\chi$ of modulus $k$, $$ L(1,\chi) > \frac{c(\epsilon)}{k^\epsilon} \ . $$ First proved by C.L. Siegel [1]. An equivalent formulation is concerned with real zeros of L-functions: For any $\epsilon > 0$ there exists a $c_1 = c_1(\epsilon) > 0$ such that $L(z,\chi) > c_1(\epsilon) \neq 0$ for $z > 1 - c_1/k^\epsilon$ for any non-principal real Dirichlet character $\chi$. The constants $c(\epsilon)$ and $c_1(\epsilon)$ are non-effective, in the sense that for no $0 < \epsilon < 1/2$ there is a way to estimate them from below. For this reason, applications of Siegel's theorem have a non-constructive character. For example, if $h(-D)$ is the divisor class number of a quadratic field of discriminant $-D$, it follows from the theorem that $$ h(-D) > c_2(\epsilon) D^{1/2-\epsilon} $$ with a non-effective constant $c_2(\epsilon)$ for $\epsilon < 1/2$. Similarly, the following estimate, which is uniform for $1 \le k \le \log x$, $(k,l) = 1$, $$ \pi(x;k,l) - \frac{1}{\phi(k)} \int_2^x \frac{dt}{\log t} = O\left({ x e^{-c_3 \sqrt{\log x}} }\right) $$ where $\pi(x;k,l)$ is the number of primes $< x$ representable as $kn + l$, involves a non-effective constant $c_3$ (cf. also Distribution of prime numbers).
References
| [1] | C.L. Siegel, "Ueber die Klassenzahl quadratischen Zahlkorper" Acta Arithmetica , 1 (1935) pp. 83–86 |
| [2] | H. Davenport, "Multiplicative number theory" , Springer (1981) |
| [3] | A.A. Karatsuba, "Fundamentals of analytic number theory" , Moscow (1975) pp. Chapt. 9 (In Russian) |
S.M. Voronin
Siegel's theorem on integral points
A theorem stating that Diophantine equations of a certain class have finitely many integral solutions. In its simplest form, the theorem states that if 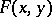 is a polynomial with integer coefficients, defining an irreducible curve
is a polynomial with integer coefficients, defining an irreducible curve 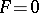 of genus
of genus 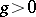 , then the equation
, then the equation  has finitely many integer solutions. This result (actually in a more general form, for algebraic integers) was established in 1929 by C.L. Siegel [1], who used the theories of Diophantine approximations and Abelian varieties. It was the culmination of a line of research in the theory of Diophantine equations initiated in 1908 by A. Thue (see [2], [3]). The theorem was subsequently generalized to the case of arbitrary affine curves of genus
has finitely many integer solutions. This result (actually in a more general form, for algebraic integers) was established in 1929 by C.L. Siegel [1], who used the theories of Diophantine approximations and Abelian varieties. It was the culmination of a line of research in the theory of Diophantine equations initiated in 1908 by A. Thue (see [2], [3]). The theorem was subsequently generalized to the case of arbitrary affine curves of genus 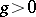 defined over subrings of finite type in global fields (see [5]). In particular, the above equation
defined over subrings of finite type in global fields (see [5]). In particular, the above equation  has finitely many solutions of the form
has finitely many solutions of the form
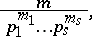 |
where 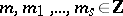 and
and 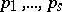 are fixed prime numbers. Till recently, the proofs of this theorem suffered from a common drawback — while stating that the number of solutions is finite, they provided no quantitative estimates (see Height, in Diophantine geometry), so that one had no algorithm for an explicit construction of solutions. The first result free of this shortcoming was due to A. Baker (1967). Effective proofs of Siegel's theorem have been obtained for various classes of Diophantine equations, but in the general case the problem is still open (see [4]). Another important problem is to generalize Siegel's theorem to varieties of dimension greater than one.
are fixed prime numbers. Till recently, the proofs of this theorem suffered from a common drawback — while stating that the number of solutions is finite, they provided no quantitative estimates (see Height, in Diophantine geometry), so that one had no algorithm for an explicit construction of solutions. The first result free of this shortcoming was due to A. Baker (1967). Effective proofs of Siegel's theorem have been obtained for various classes of Diophantine equations, but in the general case the problem is still open (see [4]). Another important problem is to generalize Siegel's theorem to varieties of dimension greater than one.
References
| [1] | C.L. Siegel, "Ueber einige Anwendungen diophantischer Approximationen" Abh. Preuss. Akad. Wiss. Phys.-Math. Kl. , 1 (1929) pp. 41–69 |
| [2] | A.O. Gel'fond, "The solution of equations in integers" , Noordhoff (1960) |
| [3] | H. Davenport, "The higher arithmetic" , Hutchinson (1952) |
| [4] | , Problems in the theory of Diophantine approximations , Moscow (1974) (In Russian; translated from English) |
| [5] | S. Lang, "Diophantine geometry" , Interscience (1962) |
A.N. Parshin
Comments
The Mordell conjecture states that for any number field and any smooth curve 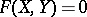 of genus
of genus 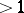 defined over
defined over 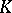 there are only finitely many
there are only finitely many 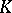 -rational solutions. This was proved by G. Faltings in 1983.
-rational solutions. This was proved by G. Faltings in 1983.
References
| [a1] | B. Mazur, "On some of the mathematical contributions of Gerd Faltings" , Proc. Internat. Congress Mathematicians (Berkeley, 1986) , Amer. Math. Soc. (1987) pp. 7–12 |
| [a2] | G. Faltings, "Endlichkeitssätze für abelschen Varietäten über Zahlkörper" Invent. Math. , 73 (1983) pp. 349–366 |
Siegel theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Siegel_theorem&oldid=33856