Difference between revisions of "Riemann integral"
m |
m (some tex) |
||
| Line 3: | Line 3: | ||
\sigma = f(\xi_1)\Delta x_1+\dots+f(\xi x_i)\Delta x_i +\dots +f(x_n)\Delta x_n, | \sigma = f(\xi_1)\Delta x_1+\dots+f(\xi x_i)\Delta x_i +\dots +f(x_n)\Delta x_n, | ||
\end{equation} | \end{equation} | ||
| − | where $x_{i-1}\leq\xi_i\leq x_i$, is called the Riemann sum corresponding to the given partition of $[a,b]$ by the points $x_i$ and to the sample of points $\xi_i$. The number $I$ is called the limit of the Riemann sums \ | + | where $x_{i-1}\leq\xi_i\leq x_i$, is called the Riemann sum corresponding to the given partition of $[a,b]$ by the points $x_i$ and to the sample of points $\xi_i$. The number $I$ is called the limit of the Riemann sums \eqref{eq:1} as $\max_i \Delta x_i \to 0$ if for any $\varepsilon>0$ a $\delta>0$ can be found such that $\max_i \Delta x_i < \delta$ implies the inequality $|\sigma - I|<\varepsilon$. If the Riemann sums have a finite limit $I$ as $\max_i \Delta x_i \to 0$, then the function $f$ is called Riemann integrable over $[a,b]$, where $a< b$. The limit is known as the definite Riemann integral of $f$ over $[a,b]$, and is written as |
| − | + | \begin{equation}\label{eq:2} | |
| − | + | \int\limits_a^bf(x)\,dx. | |
| − | + | \end{equation} | |
| − | When | + | When $a=b$ then, by definition, |
| − | + | \begin{equation} | |
| − | + | \int\limits_a^af(x)\,dx = 0, | |
| − | + | \end{equation} | |
| − | and when | + | and when $a>b$ the integral \eqref{eq:2} is defined using the equation |
| − | + | \begin{equation} | |
| − | + | \int\limits_a^bf(x)\,dx = -\int\limits_b^af(x)\,dx. | |
| − | + | \end{equation} | |
| − | A necessary and sufficient condition for the Riemann integrability of | + | A necessary and sufficient condition for the Riemann integrability of $f$ over $[a,b]$ is the boundedness of $f$ on this interval and the zero value of the [[Lebesgue measure|Lebesgue measure]] of the set of all points of discontinuity of $f$ contained in $[a,b]$. |
==Properties of the Riemann integral.== | ==Properties of the Riemann integral.== | ||
Revision as of 04:34, 23 December 2012
A generalization of the concept of a Cauchy integral to a certain class of discontinuous functions; introduced by B. Riemann (1853). Consider a function $f$ which is given on an interval $[a,b]$. Let $a=x_0<x_1<\dots<x_n=b$ is a partition (subdivision) of the interval $[a,b]$ and $\Delta x_i = x_i-x_{i-1}$, where $i=1,\dots,n$. The sum \begin{equation}\label{eq:1} \sigma = f(\xi_1)\Delta x_1+\dots+f(\xi x_i)\Delta x_i +\dots +f(x_n)\Delta x_n, \end{equation} where $x_{i-1}\leq\xi_i\leq x_i$, is called the Riemann sum corresponding to the given partition of $[a,b]$ by the points $x_i$ and to the sample of points $\xi_i$. The number $I$ is called the limit of the Riemann sums \eqref{eq:1} as $\max_i \Delta x_i \to 0$ if for any $\varepsilon>0$ a $\delta>0$ can be found such that $\max_i \Delta x_i < \delta$ implies the inequality $|\sigma - I|<\varepsilon$. If the Riemann sums have a finite limit $I$ as $\max_i \Delta x_i \to 0$, then the function $f$ is called Riemann integrable over $[a,b]$, where $a< b$. The limit is known as the definite Riemann integral of $f$ over $[a,b]$, and is written as \begin{equation}\label{eq:2} \int\limits_a^bf(x)\,dx. \end{equation} When $a=b$ then, by definition, \begin{equation} \int\limits_a^af(x)\,dx = 0, \end{equation} and when $a>b$ the integral \eqref{eq:2} is defined using the equation \begin{equation} \int\limits_a^bf(x)\,dx = -\int\limits_b^af(x)\,dx. \end{equation} A necessary and sufficient condition for the Riemann integrability of $f$ over $[a,b]$ is the boundedness of $f$ on this interval and the zero value of the Lebesgue measure of the set of all points of discontinuity of $f$ contained in $[a,b]$.
Properties of the Riemann integral.
1) Every Riemann-integrable function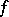 
on 
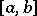is also bounded on this interval (the converse is not true: The Dirichlet function is an example of a bounded and non-integrable function on 
).
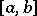2) The linearity property: For any constants 
and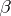 
, the integrability over 
of both functions 
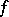and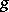 
implies that the function 
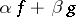is integrable over this interval, and the equation
 |
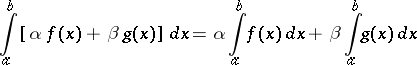holds.
3) The integrability over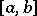 
of both functions 
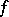and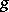 
implies that their product 
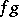is integrable over this interval.
4) Additivity: The integrability of a function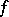 
over both intervals 
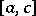and 
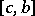implies that 
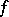is integrable over 
, and
 |
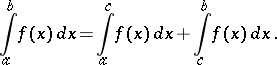5) If two functions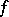 
and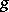 
are integrable over 
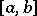and if 
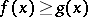for every 
in this interval, then
 |
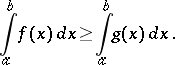6) The integrability of a function 
over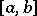 
implies that the function 
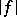is integrable over this interval, and the estimate
 |
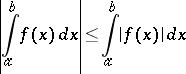holds.
7) The mean-value formula: If two real-valued functions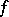 
and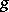 
are integrable over 
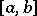, if the function 
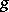is non-negative or non-positive everywhere on this interval, and if 
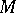and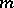 
are the least upper and greatest lower bounds of 
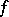on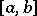 
, then a number 
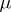can be found, 
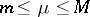, such that the formula
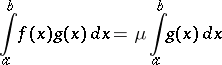 | (3) |
holds. If, in addition,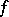 
is continuous on 
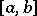, then this interval will contain a point 
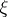such that in formula (3),
 |
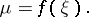8) The second mean-value formula (Bonnet's formula): If a function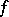 
is real-valued and integrable over 
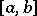and if a function 
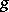is real-valued and monotone on this interval, then a point 
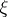can be found in 
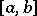such that the formula
 |
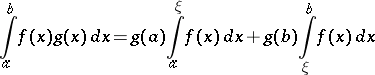holds.
References
| [1] | B. Riemann, "Ueber die Darstellbarkeit einer Function durch eine trigonometrische Reihe" H. Weber (ed.) , B. Riemann's Gesammelte Mathematische Werke , Dover, reprint (1953) pp. 227–271 ((Original: Göttinger Akad. Abh.  (1868))) |
| [2] | V.A. Il'in, E.G. Poznyak, "Fundamentals of mathematical analysis" , 1–2 , MIR (1982) (Translated from Russian) |
| [3] | L.D. Kudryavtsev, "A course in mathematical analysis" , 1–2 , Moscow (1988) (In Russian) |
| [4] | S.M. Nikol'skii, "A course of mathematical analysis" , 1–2 , MIR (1977) (Translated from Russian) |
Comments
References
| [a1] | G.E. Shilov, "Mathematical analysis" , 1–2 , M.I.T. (1974) (Translated from Russian) |
| [a2] | I.N. Pesin, "Classical and modern integration theories" , Acad. Press (1970) (Translated from Russian) |
| [a3] | K.R. Stromberg, "Introduction to classical real analysis" , Wadsworth (1981) |
| [a4] | W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1976) pp. 75–78 |
Riemann integral. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemann_integral&oldid=29263