Difference between revisions of "Lewy operator and Mizohata operator"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 11: | Line 11: | ||
Both operator have clear connections with complex analysis (cf. [[Functions of a complex variable, theory of|Functions of a complex variable, theory of]]). | Both operator have clear connections with complex analysis (cf. [[Functions of a complex variable, theory of|Functions of a complex variable, theory of]]). | ||
| − | The Lewy operator is the tangential Cauchy–Riemann operator (cf. [[Bergman kernel function|Bergman kernel function]]) on the | + | The Lewy operator is the tangential Cauchy–Riemann operator (cf. [[Bergman kernel function|Bergman kernel function]]) on the "Heisenberg group" , the hypersurface in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008019.png" /> parametrized by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008020.png" />. This simply means that the functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008021.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008022.png" /> are in the kernel of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008023.png" />. So, in other coordinates, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008024.png" /> is the Cauchy–Riemann vector field on the unit sphere in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008025.png" /> (the tangential operator characterizing holomorphic functions). An earlier paper by Lewy was [[#References|[a4]]]! |
The Mizohata operator can be obtained (up to a factor) by pulling back the standard Cauchy–Riemann operator on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008026.png" /> via the singular change of variables: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008027.png" />. Notice that indeed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008028.png" />. | The Mizohata operator can be obtained (up to a factor) by pulling back the standard Cauchy–Riemann operator on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008026.png" /> via the singular change of variables: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008027.png" />. Notice that indeed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008028.png" />. | ||
| Line 19: | Line 19: | ||
If one decomposes <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008029.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008030.png" /> into real part and imaginary part <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008031.png" />, the commutator of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008032.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008033.png" /> does not belong to the linear span of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008034.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008035.png" /> (for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008036.png" /> in case of the Mizohata operator). L.V. Hörmander understood that this fact was crucial and he showed that, more generally, a similar condition on the commutator of the higher-order terms for general linear differential operators implies non-local solvability. See [[#References|[a3]]], Chap. 6. | If one decomposes <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008029.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008030.png" /> into real part and imaginary part <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008031.png" />, the commutator of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008032.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008033.png" /> does not belong to the linear span of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008034.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008035.png" /> (for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008036.png" /> in case of the Mizohata operator). L.V. Hörmander understood that this fact was crucial and he showed that, more generally, a similar condition on the commutator of the higher-order terms for general linear differential operators implies non-local solvability. See [[#References|[a3]]], Chap. 6. | ||
| − | However, real operators may also be | + | However, real operators may also be "without solutions" , since as pointed out by F. Treves in 1962, the operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008037.png" /> is a real operator (and so is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008038.png" />). |
The question of local solvability for vector fields is totally understood thanks to L. Nirenberg and Treves (condition P), [[#References|[a8]]], [[#References|[a11]]], Chap. VIII. In <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008039.png" />, the operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008040.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008041.png" />, is locally solvable at <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008042.png" /> if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008043.png" /> is even. Again, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008044.png" /> (see [[#References|[a6]]], Appendix) is related to the Cauchy–Riemann operator via the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008045.png" /> which is one-to-one if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008046.png" /> is even. The kernel of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008047.png" /> consists of functions which are holomorphic functions of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008048.png" />, and this can be taken as the beginning of a whole theory, the theory of hypo-analytic structures initiated by M.S. Baouendi and Treves [[#References|[a1]]], [[#References|[a11]]]. | The question of local solvability for vector fields is totally understood thanks to L. Nirenberg and Treves (condition P), [[#References|[a8]]], [[#References|[a11]]], Chap. VIII. In <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008039.png" />, the operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008040.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008041.png" />, is locally solvable at <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008042.png" /> if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008043.png" /> is even. Again, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008044.png" /> (see [[#References|[a6]]], Appendix) is related to the Cauchy–Riemann operator via the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008045.png" /> which is one-to-one if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008046.png" /> is even. The kernel of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008047.png" /> consists of functions which are holomorphic functions of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120080/l12008048.png" />, and this can be taken as the beginning of a whole theory, the theory of hypo-analytic structures initiated by M.S. Baouendi and Treves [[#References|[a1]]], [[#References|[a11]]]. | ||
| Line 30: | Line 30: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> M.S Baouendi, F. Treves, "A property of the functions and distributions annihilated by a locally integrable system of complex vector fields" ''Ann. of Math.'' , '''113''' (1981) pp. 387–421 {{MR|0607899}} {{ZBL|0491.35036}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> L. Boutet de Monvel, "A course on pseudo differential operators and their applications" , Duke Univ. (1976) {{MR|}} {{ZBL|0348.35002}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> L. Hörmander, "Linear partial differential operators" , ''Grundl. Math. Wissenschaft.'' , '''116''' , Springer (1966) {{MR|1533716}} {{ZBL|1178.35003}} {{ZBL|1115.35005}} {{ZBL|1062.35004}} {{ZBL|1028.35001}} {{ZBL|0712.35001}} {{ZBL|0687.35002}} {{ZBL|0619.35002}} {{ZBL|0619.35001}} {{ZBL|0612.35001}} {{ZBL|0601.35001}} {{ZBL|0521.35002}} {{ZBL|0521.35001}} {{ZBL|0321.35001}} {{ZBL|0175.39201}} {{ZBL|0131.31804}} {{ZBL|0108.09301}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H. Lewy, "On the local character of the solutions of an atypical linear differential equation in three variables and a related problem for regular functions of two complex variables" ''Ann. of Math.'' , '''64''' (1956) pp. 514–522</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> H. Lewy, "An example of a smooth linear partial differential equation without solution" ''Ann. of Math.'' , '''66''' (1957) pp. 155–158 {{MR|0088629}} {{ZBL|0078.08104}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> S. Mizohata, "Solutions nulles et solutions non analytiques" ''J. Math. Kyoto Univ.'' , '''1''' (1962) pp. 271–302 {{MR|0142873}} {{ZBL|0106.29601}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> L. Nirenberg, "Lectures on linear partial differential equations" , ''CBMS Reg. Conf.'' , '''17''' , Amer. Math. Soc. (1973) {{MR|0450755}} {{ZBL|0267.35001}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> L. Nirenberg, F. Treves, "Solvability of a first-order linear partial differential equation" ''Commun. Pure Appl. Math.'' , '''16''' (1963) pp. 331–351 {{MR|0163045}} {{ZBL|0117.06104}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> J.P. Rosay, "CR functions vanishing on open sets. (Almost) complex structures and Cohen's example" ''Indag. Math.'' , '''9''' (1998) pp. 289–303 {{MR|1691491}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> F. Treves, "Introduction to pseudodifferential and Fourier integral operators" , Plenum (1980) {{MR|0597145}} {{MR|0597144}} {{ZBL|0453.47027}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> F. Treves, "Hypoanalytic structures, local theory" , Princeton Univ. Press (1992)</TD></TR></table> |
Revision as of 16:59, 15 April 2012
In the mid 1950s, B. Malgrange and L. Ehrenpreis showed that one can solve all linear constant-coefficients partial differential equations on  (cf. also Malgrange–Ehrenpreis theorem; Linear partial differential equation). The question of solvability for variable coefficients was posed (avoiding singular points, as needed even in the theory of ordinary differential equations). H. Lewy [a5] found the following example, which at the time (1956) was astonishing. In
(cf. also Malgrange–Ehrenpreis theorem; Linear partial differential equation). The question of solvability for variable coefficients was posed (avoiding singular points, as needed even in the theory of ordinary differential equations). H. Lewy [a5] found the following example, which at the time (1956) was astonishing. In  variables
variables  , let
, let  be the operator (called the Lewy operator):
be the operator (called the Lewy operator):
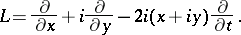 |
The Cauchy–Kovalevskaya theorem applies to this operator. So, if  is a real-analytic function (cf. Class of differentiability) defined (say) near
is a real-analytic function (cf. Class of differentiability) defined (say) near  , then the equation
, then the equation  can be solved near
can be solved near  , with
, with  real analytic. But, there exists a
real analytic. But, there exists a  -function
-function 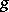 such that the equation
such that the equation 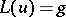 cannot be solved in any neighbourhood of (say)
cannot be solved in any neighbourhood of (say)  , even with
, even with  a distribution (cf. also Generalized function).
a distribution (cf. also Generalized function).
Later, it was noticed that the same phenomenon occurs, near any point  , for the even simpler (embarrassingly simple!) operator, in
, for the even simpler (embarrassingly simple!) operator, in  variables (called the Mizohata operator):
variables (called the Mizohata operator):
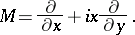 |
Both operator have clear connections with complex analysis (cf. Functions of a complex variable, theory of).
The Lewy operator is the tangential Cauchy–Riemann operator (cf. Bergman kernel function) on the "Heisenberg group" , the hypersurface in  parametrized by
parametrized by  . This simply means that the functions
. This simply means that the functions  and
and 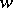 are in the kernel of
are in the kernel of 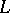 . So, in other coordinates,
. So, in other coordinates,  is the Cauchy–Riemann vector field on the unit sphere in
is the Cauchy–Riemann vector field on the unit sphere in  (the tangential operator characterizing holomorphic functions). An earlier paper by Lewy was [a4]!
(the tangential operator characterizing holomorphic functions). An earlier paper by Lewy was [a4]!
The Mizohata operator can be obtained (up to a factor) by pulling back the standard Cauchy–Riemann operator on 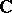 via the singular change of variables:
via the singular change of variables:  . Notice that indeed
. Notice that indeed  .
.
This connection with complex analysis allows simple, and transparent, proofs of the non-local solvability (in particular, via the obvious non-propagation of singularities for the adjoint operators).
If one decomposes 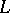 or
or  into real part and imaginary part
into real part and imaginary part  , the commutator of
, the commutator of  and
and  does not belong to the linear span of
does not belong to the linear span of  and
and  (for
(for 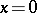 in case of the Mizohata operator). L.V. Hörmander understood that this fact was crucial and he showed that, more generally, a similar condition on the commutator of the higher-order terms for general linear differential operators implies non-local solvability. See [a3], Chap. 6.
in case of the Mizohata operator). L.V. Hörmander understood that this fact was crucial and he showed that, more generally, a similar condition on the commutator of the higher-order terms for general linear differential operators implies non-local solvability. See [a3], Chap. 6.
However, real operators may also be "without solutions" , since as pointed out by F. Treves in 1962, the operator 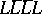 is a real operator (and so is
is a real operator (and so is  ).
).
The question of local solvability for vector fields is totally understood thanks to L. Nirenberg and Treves (condition P), [a8], [a11], Chap. VIII. In  , the operator
, the operator 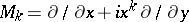 ,
, 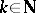 , is locally solvable at
, is locally solvable at  if and only if
if and only if 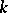 is even. Again,
is even. Again,  (see [a6], Appendix) is related to the Cauchy–Riemann operator via the mapping
(see [a6], Appendix) is related to the Cauchy–Riemann operator via the mapping  which is one-to-one if
which is one-to-one if  is even. The kernel of
is even. The kernel of 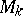 consists of functions which are holomorphic functions of
consists of functions which are holomorphic functions of  , and this can be taken as the beginning of a whole theory, the theory of hypo-analytic structures initiated by M.S. Baouendi and Treves [a1], [a11].
, and this can be taken as the beginning of a whole theory, the theory of hypo-analytic structures initiated by M.S. Baouendi and Treves [a1], [a11].
The Lewy operator and the Mizohata operator are much more similar than it may look. Indeed, they are micro-locally equivalent ([a2], Sect. 9, [a10], Chap. IX), and in fact they serve as micro-local model for non-Levi degenerate CR-structures.
Perturbations of the Lewy operator and of the Mizohata operator are of interest. See [a7], Chap. 1. One can construct arbitrary small perturbations of these operators, still as complex vector fields, in  and
and 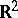 respectively, in such a way that the only functions annihilated by these operators will be those which are constant. This gives Nirenberg's example of a strictly pseudo-convex non-embeddable CR-structure (real dimension
respectively, in such a way that the only functions annihilated by these operators will be those which are constant. This gives Nirenberg's example of a strictly pseudo-convex non-embeddable CR-structure (real dimension  , CR dimension
, CR dimension  ).
).
However the Lewy and the Mizohata operators differ strikingly from the point of view of their small perturbations, for the uniqueness in the Cauchy problem (references, and an elementary exposition, can be found in [a9]).
References
| [a1] | M.S Baouendi, F. Treves, "A property of the functions and distributions annihilated by a locally integrable system of complex vector fields" Ann. of Math. , 113 (1981) pp. 387–421 MR0607899 Zbl 0491.35036 |
| [a2] | L. Boutet de Monvel, "A course on pseudo differential operators and their applications" , Duke Univ. (1976) Zbl 0348.35002 |
| [a3] | L. Hörmander, "Linear partial differential operators" , Grundl. Math. Wissenschaft. , 116 , Springer (1966) MR1533716 Zbl 1178.35003 Zbl 1115.35005 Zbl 1062.35004 Zbl 1028.35001 Zbl 0712.35001 Zbl 0687.35002 Zbl 0619.35002 Zbl 0619.35001 Zbl 0612.35001 Zbl 0601.35001 Zbl 0521.35002 Zbl 0521.35001 Zbl 0321.35001 Zbl 0175.39201 Zbl 0131.31804 Zbl 0108.09301 |
| [a4] | H. Lewy, "On the local character of the solutions of an atypical linear differential equation in three variables and a related problem for regular functions of two complex variables" Ann. of Math. , 64 (1956) pp. 514–522 |
| [a5] | H. Lewy, "An example of a smooth linear partial differential equation without solution" Ann. of Math. , 66 (1957) pp. 155–158 MR0088629 Zbl 0078.08104 |
| [a6] | S. Mizohata, "Solutions nulles et solutions non analytiques" J. Math. Kyoto Univ. , 1 (1962) pp. 271–302 MR0142873 Zbl 0106.29601 |
| [a7] | L. Nirenberg, "Lectures on linear partial differential equations" , CBMS Reg. Conf. , 17 , Amer. Math. Soc. (1973) MR0450755 Zbl 0267.35001 |
| [a8] | L. Nirenberg, F. Treves, "Solvability of a first-order linear partial differential equation" Commun. Pure Appl. Math. , 16 (1963) pp. 331–351 MR0163045 Zbl 0117.06104 |
| [a9] | J.P. Rosay, "CR functions vanishing on open sets. (Almost) complex structures and Cohen's example" Indag. Math. , 9 (1998) pp. 289–303 MR1691491 |
| [a10] | F. Treves, "Introduction to pseudodifferential and Fourier integral operators" , Plenum (1980) MR0597145 MR0597144 Zbl 0453.47027 |
| [a11] | F. Treves, "Hypoanalytic structures, local theory" , Princeton Univ. Press (1992) |
Lewy operator and Mizohata operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lewy_operator_and_Mizohata_operator&oldid=24492