Difference between revisions of "Polycyclic group"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 2: | Line 2: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> M.I. Kargapolov, J.I. [Yu.I. Merzlyakov] Merzljakov, "Fundamentals of the theory of groups" , Springer (1979) (Translated from Russian) {{MR|0551207}} {{ZBL|0549.20001}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> B.A.F. Wehrfritz, "Three lectures on polycyclic groups" , Queen Mary College London (1973)</TD></TR></table> |
| Line 12: | Line 12: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> A.I. [A.I. Mal'tsev] Mal'Avcev, "On certain classes of infinite soluble groups" ''Transl. Amer. Math. Soc. (2)'' , '''2''' (1956) pp. 1–21 ''Mat. Sb.'' , '''28''' (1951) pp. 567–588</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.A. Wolf, "Growth of finitely generated solvable groups and curvature of Riemannian manifolds" ''J. Diff. Geom.'' , '''2''' (1968) pp. 421–446 {{MR|}} {{ZBL|0207.51803}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J. Milnor, "Growth of finitely generated solvable groups" ''J. Diff. Geom.'' , '''2''' (1968) pp. 1–7 {{MR|0244899}} {{ZBL|0176.29803}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> D. Segal, "Polycyclic groups" , Cambridge Univ. Press (1983) {{MR|0713786}} {{ZBL|0516.20001}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> L. Auslander, "On a problem of Philip Hall" ''Ann. of Math.'' , '''86''' (1967) pp. 112–116 {{MR|0218454}} {{ZBL|0149.26904}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> D. Segal, "The general polycyclic group" ''Bull. London Math. Soc.'' , '''19''' (1987) pp. 49–56 {{MR|0865039}} {{ZBL|0606.20030}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> K.A. Hirsch, "On infinite soluble groups" ''Proc. London Math. Soc.'' , '''44''' (1938) pp. 53–60 {{MR|0061599}} {{MR|0044526}} {{MR|0017281}} {{ZBL|0019.15602}} {{ZBL|0018.14505}} {{ZBL|64.0066.01}} </TD></TR></table> |
Revision as of 21:55, 30 March 2012
A group possessing a polycyclic series, i.e. a subnormal series with cyclic factors (see Subgroup series). The class of polycyclic groups coincides with the class of solvable groups with the maximum condition for subgroups; it is closed under transition to subgroups, quotient groups and extensions. The number of infinite factors in any polycyclic series is an invariant of the polycyclic group (the polycyclic dimension). The holomorph of a polycyclic group (cf. Holomorph of a group) is isomorphic to a group of matrices over the ring of integers; this enables one to use methods from algebraic geometry, number theory and 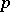 -adic analysis in the theory of polycyclic groups. If
-adic analysis in the theory of polycyclic groups. If 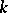 is an algebraic extension of a finite field and
is an algebraic extension of a finite field and 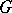 is a finite extension of a polycyclic group, then any simple
is a finite extension of a polycyclic group, then any simple  -module is finite-dimensional over
-module is finite-dimensional over 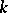 . In any group, the product of two locally polycyclic normal subgroups is a locally polycyclic subgroup.
. In any group, the product of two locally polycyclic normal subgroups is a locally polycyclic subgroup.
References
| [1] | M.I. Kargapolov, J.I. [Yu.I. Merzlyakov] Merzljakov, "Fundamentals of the theory of groups" , Springer (1979) (Translated from Russian) MR0551207 Zbl 0549.20001 |
| [2] | B.A.F. Wehrfritz, "Three lectures on polycyclic groups" , Queen Mary College London (1973) |
Comments
Every solvable linear group over the integers is polycyclic, [a1]. A solvable group is polycyclic if and only if every subgroup is finitely generated, [a2]. The Milnor–Wolf theorem says that a finitely-generated solvable group is either of polynomial or of exponential growth (cf. Polynomial and exponential growth in groups and algebras), and if it is of polynomial growth, then it is polycyclic and almost nilpotent (i.e. it contains a subgroup of finite index that is nilpotent) [a2], [a3]. If 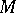 is a complete, connected, locally homogeneous Riemannian manifold, then every solvable subgroup of its homotopy group
is a complete, connected, locally homogeneous Riemannian manifold, then every solvable subgroup of its homotopy group 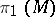 is polycyclic, [a2].
is polycyclic, [a2].
The theorem that every polycyclic group is isomorphic to a matrix group over the integers was first proved in [a5]. The theorem that the polycyclic groups are precisely the solvable groups satisfying the maximum condition for subgroups comes from [a7].
References
| [a1] | A.I. [A.I. Mal'tsev] Mal'Avcev, "On certain classes of infinite soluble groups" Transl. Amer. Math. Soc. (2) , 2 (1956) pp. 1–21 Mat. Sb. , 28 (1951) pp. 567–588 |
| [a2] | J.A. Wolf, "Growth of finitely generated solvable groups and curvature of Riemannian manifolds" J. Diff. Geom. , 2 (1968) pp. 421–446 Zbl 0207.51803 |
| [a3] | J. Milnor, "Growth of finitely generated solvable groups" J. Diff. Geom. , 2 (1968) pp. 1–7 MR0244899 Zbl 0176.29803 |
| [a4] | D. Segal, "Polycyclic groups" , Cambridge Univ. Press (1983) MR0713786 Zbl 0516.20001 |
| [a5] | L. Auslander, "On a problem of Philip Hall" Ann. of Math. , 86 (1967) pp. 112–116 MR0218454 Zbl 0149.26904 |
| [a6] | D. Segal, "The general polycyclic group" Bull. London Math. Soc. , 19 (1987) pp. 49–56 MR0865039 Zbl 0606.20030 |
| [a7] | K.A. Hirsch, "On infinite soluble groups" Proc. London Math. Soc. , 44 (1938) pp. 53–60 MR0061599 MR0044526 MR0017281 Zbl 0019.15602 Zbl 0018.14505 Zbl 64.0066.01 |
Polycyclic group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Polycyclic_group&oldid=23926