Difference between revisions of "Čech cohomology"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Čech cohomology to Cech cohomology: ascii title) |
(No difference)
| |
Revision as of 18:51, 24 March 2012
Aleksandrov–Čech cohomology, spectral cohomology
The direct limit
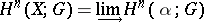 |
of the cohomology groups with coefficients in an Abelian group  of the nerves of all open coverings
of the nerves of all open coverings  of a topological space
of a topological space  . The cohomology group of a closed subset
. The cohomology group of a closed subset  can be defined analogously, using the subsystem
can be defined analogously, using the subsystem  of all such sets from
of all such sets from  that have non-empty intersection with
that have non-empty intersection with  . The limit of the groups of the pair
. The limit of the groups of the pair  defines the cohomology group
defines the cohomology group  of the pair
of the pair  . The cohomology sequence
. The cohomology sequence
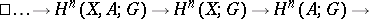 |
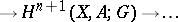 |
of the pair  is exact, being the limit of the exact cohomology sequences of the pairs of nerves of
is exact, being the limit of the exact cohomology sequences of the pairs of nerves of  .
.
Aleksandrov–Čech cohomology serves as a substitute for singular cohomology in general categories of topological spaces, and agrees with it whenever the applicability of the latter is not in doubt (specifically, in the case of homologically, locally connected spaces, in particular locally contractible spaces). It satisfies all Steenrod–Eilenberg axioms, and on the category of paracompact spaces it is uniquely determined by those axioms together with the following conditions: a)  for
for 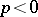 ; b) the cohomology group of a discrete union
; b) the cohomology group of a discrete union 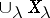 is naturally isomorphic to the direct product of the cohomology groups of the
is naturally isomorphic to the direct product of the cohomology groups of the 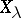 ; and c)
; and c) 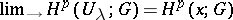 for the system of all neighbourhoods
for the system of all neighbourhoods 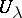 of an arbitrary point
of an arbitrary point 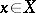 . Aleksandrov–Čech cohomology is isomorphic to Alexander–Spanier cohomology. The latter can be defined with coefficients in a sheaf, and for paracompact spaces it is isomorphic to the cohomology defined in sheaf theory.
. Aleksandrov–Čech cohomology is isomorphic to Alexander–Spanier cohomology. The latter can be defined with coefficients in a sheaf, and for paracompact spaces it is isomorphic to the cohomology defined in sheaf theory.
The approximability of spaces by polyhedra — by the nerves of closed coverings — was established by P.S. Aleksandrov (cf. [1]–[3]). For a particular case he has given the definition of an inverse limit of topological spaces, and, on the basis of the approximation, the definition of the Betti numbers of metrizable compacta. The homology groups of compacta are defined in terms of Vietoris cycles. L.S. Pontryagin [4] introduced direct and inverse spectra of groups, and applied these notions to the study of the homology groups of compacta. E. Čech began to consider nerves of finite open coverings of non-compact spaces and on this basis initiated the homology theory of arbitrary topological spaces. It turned out later that it is not justifiable to consider only finite open coverings (since this leads to the rather complicated homology of the Stone–Čech compactification). C.H. Dowker [5] demonstrated that it is fruitful to use arbitrary open coverings in the homology and cohomology theory of non-compact spaces.
References
| [1] | P.S. Aleksandrov, Math. Ann. , 96 (1927) pp. 489–511 |
| [2] | P.S. Aleksandrov, C.R. Acad. Sci. Paris , 184 (1927) pp. 317–320 |
| [3] | P.S. [P.S. Aleksandrov] Aleksandroff, "Untersuchungen über Gestalt und Lage abgeschlossener Mengen beliebiger Dimension" Ann. of Math. (2) , 30 (1929) pp. 101–187 |
| [4] | L.S. Pontryagin, Math. Ann. , 105 : 2 (1931) pp. 165–205 |
| [5] | C.H. Dowker, "Mapping theorems for non-compact spaces" Amer. J. Math. , 69 (1947) pp. 200–242 |
| [6] | N.E. Steenrod, S. Eilenberg, "Foundations of algebraic topology" , Princeton Univ. Press (1966) |
| [7] | E.G. Sklyarenko, "On homology theory associated with the Aleksandrov–Čech cohomology" Russian Math. Surveys , 34 : 6 (1979) pp. 103–137 Uspekhi Mat. Nauk , 34 : 6 (1979) pp. 90–118 |
| [8] | W.S. Massey, "Notes on homology and cohomology theory" , Yale Univ. Press (1964) pp. Chapt. 1 - 3; 8; Appendix to Chapt. 6 |
| [9] | E. Čech, "Théorie générale de l'homologie dans un espace quelconque" Fund. Math. , 19 (1932) pp. 149–183 |
Comments
See also Aleksandrov–Čech homology and cohomology.
Čech cohomology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=%C4%8Cech_cohomology&oldid=22275