Difference between revisions of "Absorbing state"
(newer MSC template) |
(newer MSC template) |
||
| Line 1: | Line 1: | ||
''of a Markov chain <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/a/a010/a010430/a0104301.png" />'' | ''of a Markov chain <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/a/a010/a010430/a0104301.png" />'' | ||
| − | {{ | + | [[Category:Markov chains]] |
| + | |||
| + | {{MSC|60J10}} | ||
A state <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/a/a010/a010430/a0104302.png" /> such that | A state <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/a/a010/a010430/a0104302.png" /> such that | ||
| Line 43: | Line 45: | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> W. Feller, "An introduction to probability theory and its applications" , '''1''' , Wiley (1968)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> W. Feller, "An introduction to probability theory and its applications" , '''1''' , Wiley (1968)</TD></TR></table> | ||
| − | |||
| − | |||
Revision as of 08:04, 27 January 2012
of a Markov chain 
2020 Mathematics Subject Classification: Primary: 60J10 [MSN][ZBL]
A state  such that
such that
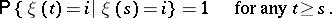 |
An example of a Markov chain with absorbing state  is a branching process.
is a branching process.
The introduction of additional absorbing states is a convenient technique that enables one to examine the properties of trajectories of a Markov chain that are associated with hitting some set.
Example. Consider the set  of states of a homogeneous Markov chain
of states of a homogeneous Markov chain  with discrete time and transition probabilities
with discrete time and transition probabilities
 |
in which a subset  is distinguished and suppose one has to find the probabilities
is distinguished and suppose one has to find the probabilities
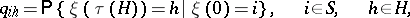 |
where 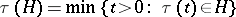 is the moment of first hitting the set
is the moment of first hitting the set  . If one introduces the auxiliary Markov chain
. If one introduces the auxiliary Markov chain 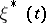 differing from
differing from 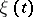 only in that all states
only in that all states  are absorbing in
are absorbing in 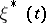 , then for
, then for 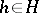 the probabilities
the probabilities
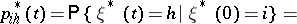 |
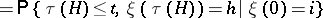 |
are monotonically non-decreasing for 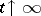 and
and
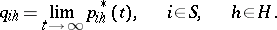 | (*) |
By virtue of the basic definition of a Markov chain
 |
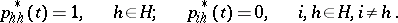 |
The passage to the limit for  taking into account (*) gives a system of linear equations for
taking into account (*) gives a system of linear equations for 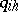 :
:
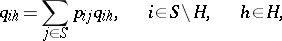 |
 |
References
| [1] | W. Feller, "An introduction to probability theory and its applications" , 1 , Wiley (1968) |
Absorbing state. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Absorbing_state&oldid=20568