Difference between revisions of "Branching process with a random medium"
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
(refs format) |
||
| Line 17: | Line 17: | ||
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756017.png" /></td> <td valign="top" style="width:5%;text-align:right;">(*)</td></tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756017.png" /></td> <td valign="top" style="width:5%;text-align:right;">(*)</td></tr></table> | ||
| − | (for a branching Galton–Watson process, i.e. for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756018.png" />, the right-hand side of (*) equals the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756019.png" />-fold iteration of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756020.png" />). Branching processes in a random medium may be subcritical, critical and supercritical: the "criticality parameter" here (see | + | (for a branching Galton–Watson process, i.e. for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756018.png" />, the right-hand side of (*) equals the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756019.png" />-fold iteration of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756020.png" />). Branching processes in a random medium may be subcritical, critical and supercritical: the "criticality parameter" here (see {{Cite|AN}}) is the variable |
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756021.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b017/b017560/b01756021.png" /></td> </tr></table> | ||
| Line 54: | Line 54: | ||
====References==== | ====References==== | ||
| − | + | {| | |
| − | + | |valign="top"|{{Ref|AN}}|| K.B. Athreya, P.E. Ney, "Branching processes" , Springer (1972) {{MR|0373040}} {{ZBL|0259.60002}} | |
| − | + | |} | |
====Comments==== | ====Comments==== | ||
Additional references can be found in the article [[Branching process|Branching process]]. | Additional references can be found in the article [[Branching process|Branching process]]. | ||
Revision as of 06:18, 11 May 2012
branching process in a random medium
2020 Mathematics Subject Classification: Primary: 60J80 [MSN][ZBL]
A time-inhomogeneous branching process in which the inhomogeneity is random. Let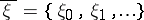 
be a stationary sequence of random variables (the value of 
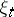is interpreted as the state of the "medium" at the moment of time 
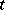), and let to each possible state of the medium 
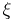correspond a probability distribution 
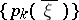of the number of descendants of a single particle:
 |
 |
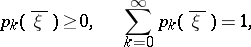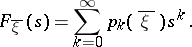In order to construct a trajectory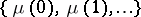 
of a branching process in a random medium the value of 
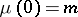and the trajectory 
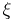of the states of the medium are fixed, and 
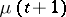is determined for each 
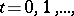as a sum of 
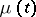independent random variables with distribution 
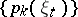. Such a complication of the branching Galton–Watson process is natural if, for example, the branching process in a random medium is regarded as a model of a biological population.
The properties of branching processes in a random medium are analogous to those of ordinary branching processes. For instance, the generating distribution function of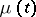 
, under the condition 
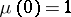, has the form
 | (*) |
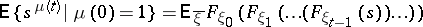(for a branching Galton–Watson process, i.e. for 
, the right-hand side of (*) equals the 
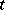-fold iteration of 
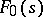). Branching processes in a random medium may be subcritical, critical and supercritical: the "criticality parameter" here (see [AN]) is the variable
 |
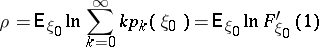(for ordinary branching processes, the "criticality parameter" is the mathematical expectation of the number of "descendants" of a single particle). If 
, the branching process in a random medium is said to be subcritical and, for the random variable
 |
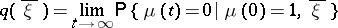which is the probability of extinction of the branching process in a random medium for a given trajectory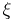 
, the relation
 |
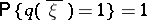is valid. There is also the analogue of the limit theorem of the subcritical Galton–Watson branching process: For almost all realizations of the sequence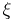 
the limits
 |
exist and satisfy
 |
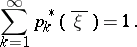If 
, the branching process in a random medium is said to be critical, and
 |
and, for almost all realizations of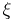 
,
 |
If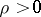 
, the branching process in a random medium is said to be supercritical; in such a case
 |
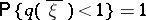and, if certain additional conditions are met, there exists for almost all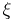 
a non-negative random variable 
,
 |
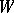References
| [AN] | K.B. Athreya, P.E. Ney, "Branching processes" , Springer (1972) MR0373040 Zbl 0259.60002 |
Comments
Additional references can be found in the article Branching process.
Branching process with a random medium. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Branching_process_with_a_random_medium&oldid=23587