Difference between revisions of "Von Kármán equations"
Ulf Rehmann (talk | contribs) m (moved Von Karman equations to Von Kármán equations over redirect: accented title) |
m (link) |
||
| Line 1: | Line 1: | ||
''for plates'' | ''for plates'' | ||
| − | A semi-linear elliptic system of two fourth-order partial differential equations with two independent spatial variables (cf. also [[Elliptic partial differential equation|Elliptic partial differential equation]]). They were proposed by T. von Kármán [[#References|[a10]]] to describe the equilibrium of thin elastic plates undergoing displacements of moderate size with small strain under the action of normal forces (cf. also [[Elasticity, mathematical theory of|Elasticity, mathematical theory of]]; [[Elasticity theory, planar problem of|Elasticity theory, planar problem of]]). For an isotropic, homogeneous plate with constant thickness <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100601.png" />, with constant elastic (Young) modulus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100602.png" />, and with constant Poisson ratio <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100603.png" />, these equations for the unknowns <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100604.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100605.png" /> have the form | + | A semi-linear elliptic system of two fourth-order partial differential equations with two independent spatial variables (cf. also [[Elliptic partial differential equation|Elliptic partial differential equation]]). They were proposed by T. von Kármán [[#References|[a10]]] to describe the equilibrium of thin elastic plates undergoing displacements of moderate size with small strain under the action of normal forces (cf. also [[Elasticity, mathematical theory of|Elasticity, mathematical theory of]]; [[Elasticity theory, planar problem of|Elasticity theory, planar problem of]]). For an isotropic, homogeneous plate with constant thickness <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100601.png" />, with constant elastic (Young) modulus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100602.png" />, and with constant [[Poisson ratio]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100603.png" />, these equations for the unknowns <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100604.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100605.png" /> have the form |
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100606.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v110/v110060/v1100606.png" /></td> </tr></table> | ||
Revision as of 17:19, 28 December 2017
for plates
A semi-linear elliptic system of two fourth-order partial differential equations with two independent spatial variables (cf. also Elliptic partial differential equation). They were proposed by T. von Kármán [a10] to describe the equilibrium of thin elastic plates undergoing displacements of moderate size with small strain under the action of normal forces (cf. also Elasticity, mathematical theory of; Elasticity theory, planar problem of). For an isotropic, homogeneous plate with constant thickness  , with constant elastic (Young) modulus
, with constant elastic (Young) modulus  , and with constant Poisson ratio
, and with constant Poisson ratio  , these equations for the unknowns
, these equations for the unknowns  and
and  have the form
have the form
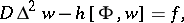 |
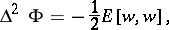 |
where
 |
is the two-dimensional biharmonic operator (cf. Biharmonic function) acting on a function  ,
,
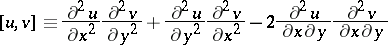 |
is the Monge–Ampère operator (cf. Monge–Ampère equation) acting on the functions  and
and  ,
,
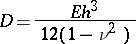 |
is a positive material constant, called the flexural rigidity, and 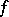 is a prescribed function. For non-homogeneous or anisotropic plates, the biharmonic operator is replaced by slightly more general operators with the same structure (see [a7], Sect. 64). The von Kármán equations are supplemented with any of a variety of boundary conditions, which express the way the plate is supported on its edge. (These conditions have the same nature as those for linear plate theories, which are discussed in many of the books listed in [a9].)
is a prescribed function. For non-homogeneous or anisotropic plates, the biharmonic operator is replaced by slightly more general operators with the same structure (see [a7], Sect. 64). The von Kármán equations are supplemented with any of a variety of boundary conditions, which express the way the plate is supported on its edge. (These conditions have the same nature as those for linear plate theories, which are discussed in many of the books listed in [a9].)
To interpret the variables of the von Kármán equations, one conceives of a plate of constant thickness 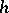 as a three-dimensional body whose natural (unstressed) state in Euclidean
as a three-dimensional body whose natural (unstressed) state in Euclidean 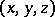 -space occupies a thin region of the form
-space occupies a thin region of the form 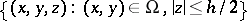 , where
, where  is a connected region in the
is a connected region in the  -plane. One identifies a material point of this body by its Cartesian coordinates
-plane. One identifies a material point of this body by its Cartesian coordinates 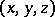 . Then
. Then 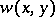 denotes the component of displacement of the material point
denotes the component of displacement of the material point  in the direction normal to the plane
in the direction normal to the plane 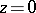 , and
, and 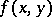 denotes the resultant of external force normal to this plane per unit of its area acting on material points with coordinates
denotes the resultant of external force normal to this plane per unit of its area acting on material points with coordinates 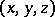 . Other components of force are regarded as negligible.
. Other components of force are regarded as negligible. 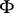 is a stress function; second derivatives of it give the components of average stress in the plane. By means of auxiliary relations inherent in the modelling process leading to the von Kármán equations, other geometric and mechanical variables can be expressed in terms of
is a stress function; second derivatives of it give the components of average stress in the plane. By means of auxiliary relations inherent in the modelling process leading to the von Kármán equations, other geometric and mechanical variables can be expressed in terms of  and
and 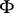 . The von Kármán equations can readily be characterized as the Euler–Lagrange equations (cf. Euler–Lagrange equation) for a suitable energy functional (cf. [a5]). Several dynamical versions of the von Kármán equations are available (see the references in [a9]).
. The von Kármán equations can readily be characterized as the Euler–Lagrange equations (cf. Euler–Lagrange equation) for a suitable energy functional (cf. [a5]). Several dynamical versions of the von Kármán equations are available (see the references in [a9]).
Von Kármán [a10] derived these equations by a heuristic method in which he discarded certain terms he regarded as physically or geometrically negligible. P.G. Ciarlet [a2] (in work generalized by J.-L. Davet [a6], cf. [a3]) showed that these equations could be systematically derived as the leading term of a formal asymptotic expansion in a thickness parameter of the exact equations of three-dimensional non-linear elasticity (away from the boundary), provided that the applied forces are suitably scaled in terms of the thickness parameter.
The beautiful mathematical structure of the von Kármán equations has attracted a considerable amount of mathematical analysis. Many of the major advances in steady-state bifurcation theory were stimulated and illustrated by studies of buckling of plates described by these equations. (See [a5], [a11] for examples and references. Global analyses of these equations are merely of mathematical interest because the assumptions underlying the equations lose their physical validity when the strains are large.)
The von Kármán equations are typical of many linear and semi-linear models for plates and shells (a comprehensive bibliography for which is given in [a9]; see also [a4] and Shell theory). These models may be contrasted with geometrically exact theories, for which the equilibrium of elastic plates is governed by quasi-linear elliptic systems (cf. [a1], [a8] and Quasi-linear equation). These theories account for large deformations and non-linear constitutive laws for bodies that need not be exceedingly thin. The analytic difficulties posed by the quasi-linear systems have so far (1996) prevented the treatment of concrete problems other than those governed by ordinary differential equations.
References
| [a1] | S.S. Antman, "Nonlinear problems of elasticity" , Springer (1995) |
| [a2] | P.G. Ciarlet, "A justification of the von Kármán equations" Arch. Rational Mech. Anal. , 73 (1980) pp. 349–389 |
| [a3] | P.G. Ciarlet, "Plates and junctions in elastic multi-structures" , Masson (1990) |
| [a4] | P.G. Ciarlet, V. Lods, "Asymptotic analysis of linearly elastic shells I. Justification of membrane shell equations" Arch. Rational Mech. Anal. , 136 (1996) pp. 119–161 |
| [a5] | P.G. Ciarlet, P. Rabier, "Les équations de von Kármán" , Springer (1980) |
| [a6] | J.-L. Davet, "Justification de modèles de plaques nonlinéaires pour des lois de comportment générales" Mod. Math. Anal. Num. , 20 (1986) pp. 147–192 |
| [a7] | S.G. Lekhnitskii, "Anisotropic plates" , GITTL (1957) (In Russian) (Edition: Second) |
| [a8] | P.M. Naghdi, "Theory of shells" C. Truesdell (ed.) , Handbuch der Physik , VIa/2 , Springer (1972) pp. 425–640 |
| [a9] | A.K. Noor, "List of books, monographs, conference proceedings and survey papers on shells" A.K. Noor (ed.) T. Belytschko (ed.) J.C. Simo (ed.) , Analytic and Computational Models of Shells , Amer. Soc. Mech. Engrs. (1989) pp. vii–xxxiii |
| [a10] | T. von Kármán, "Festigkeitsprobleme in Maschinenbau" F. Klein (ed.) C. Müller (ed.) , Encyklopädie der Mathematischen Wissenschaften , IV/4 , Teubner (1910) pp. 311–385 |
| [a11] | I.I. Vorovich, "Mathematical problems of the nonlinear theory of shallow shells" , Nauka (1989) (In Russian) |
Von Kármán equations. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Von_K%C3%A1rm%C3%A1n_equations&oldid=23552