Bateman-Horn conjecture

A conjecture on the asymptotic behaviour of a polynomial satisfying the Bunyakovskii condition (cf. also Bunyakovskii conjecture).

Let 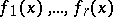be polynomials (cf. Polynomial) with integer coefficients, of degrees 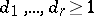, irreducible (cf. Irreducible polynomial), and with positive leading coefficients. Let

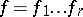be their product.

V. Bunyakovskii considered the case 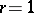and asked whether 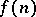could represent infinitely many prime numbers as ranges over the positive integers. An obvious necessary condition is that all coefficients of be relatively prime. However, that is not sufficient. He conjectured that, in addition, the following Bunyakovskii condition is sufficient: there is no prime number 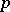dividing all the values 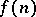for the positive integers (cf. Bunyakovskii conjecture).

Assuming the Bunyakovskii condition, let

where is the number of solutions of the congruence equation 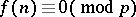(for 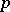prime). The Bateman–Horn conjecture asserts that

where 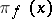is the number of positive integers such that all 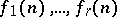are prime.

This formula gives the density of primes in an arithmetic progression (cf. Dirichlet theorem), using the polynomial 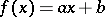. After some computations, it gives the asymptotic behaviour conjectured by G.H. Hardy and J.E. Littlewood for the number of primes representable by the polynomial . It also gives the Hardy–Littlewood conjecture for the behaviour of the number of twin primes, by applying the formula to the polynomials and (cf. also Twins). Similarly, it implies many other conjectures of Hardy and Littlewood stated in [a2].

See also Distribution of prime numbers.

References

[a1]	P.T. Bateman, R. Horn, "A heuristic formula concerning the distribution of prime numbers" Math. Comp. , 16 (1962) pp. 363–367
[a2]	G.H. Hardy, J.E. Littlewood, "Some problems of Partitio Numerorum III" Acta Math. , 44 (1922) pp. 1–70
[a3]	H. Halberstam, H.-E. Richert, "Sieve methods" , Acad. Press (1974)