Difference between revisions of "Saddle point"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 11: | Line 11: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> M.W. Hirsch, S. Smale, "Differential equations, dynamical systems, and linear algebra" , Acad. Press (1974) pp. 190ff</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> D.R.J. Chillingworth, "Differential topology with a view to applications" , Pitman (1976) pp. 150ff</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> M.W. Hirsch, S. Smale, "Differential equations, dynamical systems, and linear algebra" , Acad. Press (1974) pp. 190ff {{MR|0486784}} {{ZBL|0309.34001}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> D.R.J. Chillingworth, "Differential topology with a view to applications" , Pitman (1976) pp. 150ff {{MR|0646088}} {{ZBL|0336.58001}} </TD></TR></table> |
Revision as of 12:12, 27 September 2012
A point on a smooth surface such that the surface near the point lies on different sides of the tangent plane. If a point on a twice continuously-differentiable surface is a saddle point, then the Gaussian curvature of the surface at the point is non-positive. A saddle point is a generalization of a hyperbolic point.
Comments
A surface all of whose points are saddle points is a saddle surface.
A saddle point of a differentiable function 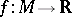 is a point
is a point  of the differentiable manifold
of the differentiable manifold 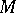 which is critical, i.e.
which is critical, i.e.  , non-degenerate, i.e. the Hessian matrix
, non-degenerate, i.e. the Hessian matrix 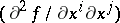 is non-singular, and such that
is non-singular, and such that  is not a local maximum or a local minimum, i.e. the Hessian matrix is indefinite. Thus, a non-degenerate critical point of
is not a local maximum or a local minimum, i.e. the Hessian matrix is indefinite. Thus, a non-degenerate critical point of  is a saddle point if its index (the number of negative eigenvalues of the Hessian matrix at that point) is
is a saddle point if its index (the number of negative eigenvalues of the Hessian matrix at that point) is  . (The index does not depend on the local coordinates chosen.) The graph of a real-valued function of two variables
. (The index does not depend on the local coordinates chosen.) The graph of a real-valued function of two variables 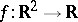 near a saddle point looks like a saddle. See also Saddle point in game theory.
near a saddle point looks like a saddle. See also Saddle point in game theory.
A saddle of a differential equation on 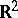 is also often called a saddle point of that differential equation. More generally, given a dynamical system
is also often called a saddle point of that differential equation. More generally, given a dynamical system  on
on 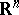 (or on a differentiable manifold) one considers the eigenvalues of
(or on a differentiable manifold) one considers the eigenvalues of  at an equilibrium point
at an equilibrium point 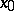 . If both positive and negative real parts occur,
. If both positive and negative real parts occur, 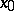 is called a saddle, a saddle point or, sometimes, a Poincaré saddle point.
is called a saddle, a saddle point or, sometimes, a Poincaré saddle point.
References
| [a1] | M.W. Hirsch, S. Smale, "Differential equations, dynamical systems, and linear algebra" , Acad. Press (1974) pp. 190ff MR0486784 Zbl 0309.34001 |
| [a2] | D.R.J. Chillingworth, "Differential topology with a view to applications" , Pitman (1976) pp. 150ff MR0646088 Zbl 0336.58001 |
Saddle point. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Saddle_point&oldid=13423