Galois field

finite field

A field with a finite number of elements. First considered by E. Galois [1].

The number of elements of any finite field is a power $p^n$ of a prime number $p$, which is the characteristic of this field. For any prime number $p$ and any natural number $n$ there exists a (unique up to an isomorphism) field of $p^n$ elements. It is denoted by $\mathrm{GF}(p^n)$ or by $\mathbb{F}_{p^n}$. The field $\mathrm{GF}(p^m)$ contains the field $\mathrm{GF}(p^n)$ as a subfield if and only if $m$ is divisible by $n$. In particular, any field $\mathrm{GF}(p^n)$ contains the field $\mathrm{GF}(p)$, which is called the prime field of characteristic $p$. The field $\mathrm{GF}(p)$ is isomorphic to the field $\mathbb{Z}/p\mathbb{Z}$ of residue classes of the ring of integers modulo $p$. In any fixed algebraic closure $\Omega$ of $\mathrm{GF}(p)$ there exists exactly one subfield $\mathrm{GF}(p^n)$ for each $n$. The correspondence $n \leftrightarrow \mathrm{GF}(p^n)$ is an isomorphism between the lattice of natural numbers with respect to division and the lattice of finite algebraic extensions (in $\Omega$) of $\mathrm{GF}(p)$ with respect to inclusion. The lattice of finite algebraic extensions of any Galois field within its fixed algebraic closure is such a lattice.

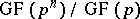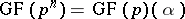The algebraic extension is simple, i.e. there exists a primitive element such that . Such an 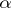will be any root of any irreducible polynomial of degree from the ring 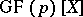. The number of primitive elements of the extension equals

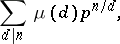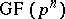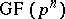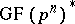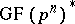where is the Möbius function. The additive group of the field is naturally endowed with the structure of an -dimensional vector space over . As a basis one may take . The non-zero elements of form a multiplicative group, , of order , i.e. each element of is a root of the polynomial 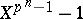. The group 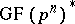is cyclic, and its generators are the primitive roots of unity of degree 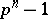, the number of which is 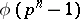, where is the Euler function. Each primitive root of unity of degree 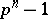is a primitive element of the extension , but the converse is not true. More exactly, out of the

irreducible unitary polynomials of degree over there are polynomials of which the roots are generators of .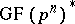

The set of elements of 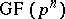coincides with the set of roots of the polynomial in , i.e. 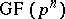is characterized as the subfield of elements from that are invariant with respect to the automorphism 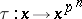, which is known as the Frobenius automorphism. If 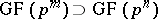, the extension 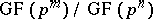is normal (cf. Extension of a field), and its Galois group 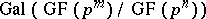is cyclic of order . The automorphism may be taken as the generator of .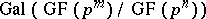

References

[1]	E. Galois, "Écrits et mémoires d'E. Galois" , Gauthier-Villars (1962)
[2]	B.L. van der Waerden, "Algebra" , 1–2 , Springer (1967–1971) (Translated from German)
[3]	N.G. [N.G. Chebotarev] Tschebotaröw, "Grundzüge der Galois'schen Theorie" , Noordhoff (1950) (Translated from Russian)
[4]	N. Bourbaki, "Algebra" , Elements of mathematics , 1 , Springer (1989) pp. Chapt. 1–3 (Translated from French)