Fredholm solvability

Let 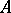be a real 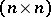-matrix and a vector.

The Fredholm alternative in states that the equation has a solution if and only if for every vector satisfying .

This alternative has many applications, e.g. in bifurcation theory. It can be generalized to abstract spaces. So, let 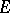and 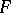be Banach spaces (cf. Banach space) and let 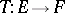be a continuous linear operator. Let 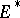, respectively 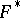, denote the topological dual of , respectively , and let 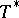denote the adjoint of 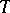(cf. also Duality; Adjoint operator). Define

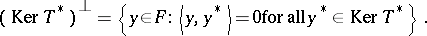An equation 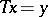is said to be normally solvable (in the sense of F. Hausdorff) if it has a solution whenever 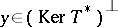(cf. also Normal solvability). A classical result states that 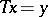is normally solvable if and only if 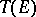is closed in .

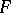In non-linear analysis, this latter result is used as definition of normal solvability for non-linear operators.

The phrase "Fredholm solvability" refers to results and techniques for solving differential and integral equations via the Fredholm alternative and, more generally, the Fredholm-type properties of the operator involved.

References

[a1]	F. Hausdorff, "Zur Theorie der linearen metrischen Räume" J. Reine Angew. Math. , 167 (1932) pp. 265 Zbl 0003.33104 Zbl 58.1113.05
[a2]	V.A. Kozlov, V.G. Maz'ya, J. Rossmann, "Elliptic boundary value problems in domains with point singularities" , Amer. Math. Soc. (1997) MR1469972 Zbl 0947.35004
[a3]	A.T. Prilepko, D.G. Orlovsky, I.A. Vasin, "Methods for solving inverse problems in mathematical physics" , M. Dekker (2000) MR1748236 Zbl 0947.35173
[a4]	D.G. Orlovskij, "The Fredholm solvability of inverse problems for abstract differential equations" A.N. Tikhonov (ed.) et al. (ed.) , Ill-Posed Problems in the Natural Sciences , VSP (1992) Zbl 0789.35178