Difference between revisions of "Markov inequality"
From Encyclopedia of Mathematics
(Importing text file) |
(link) |
||
| Line 9: | Line 9: | ||
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m062/m062470/m0624706.png" /></td> <td valign="top" style="width:5%;text-align:right;">(*)</td></tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m062/m062470/m0624706.png" /></td> <td valign="top" style="width:5%;text-align:right;">(*)</td></tr></table> | ||
| − | Inequality (*) was obtained by A.A. Markov in 1889 (see [[#References|[1]]]). The Markov inequality is exact (best possible). Thus, | + | Inequality (*) was obtained by A.A. Markov in 1889 (see [[#References|[1]]]). The Markov inequality is exact (best possible). Thus, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m062/m062470/m0624707.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m062/m062470/m0624708.png" />, considering the [[Chebyshev polynomials]] |
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m062/m062470/m0624709.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m062/m062470/m0624709.png" /></td> </tr></table> | ||
Revision as of 20:33, 16 January 2016
for derivatives of algebraic polynomials
An equality giving an estimate of the uniform norm of the derivative in terms of the uniform norm of the polynomial itself. Let 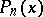 be an algebraic polynomial of degree not exceeding
be an algebraic polynomial of degree not exceeding  and let
and let
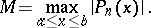 |
Then for any  in
in 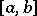 ,
,
 | (*) |
Inequality (*) was obtained by A.A. Markov in 1889 (see [1]). The Markov inequality is exact (best possible). Thus, for  ,
,  , considering the Chebyshev polynomials
, considering the Chebyshev polynomials
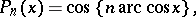 |
then
 |
and inequality (*) becomes an equality.
For derivatives of arbitrary order 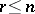 , Markov's inequality implies that
, Markov's inequality implies that
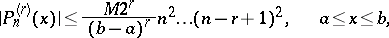 |
which already for 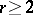 is not exact. An exact inequality for
is not exact. An exact inequality for  was obtained by V.A. Markov [2]:
was obtained by V.A. Markov [2]:
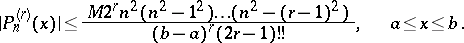 |
References
| [1] | A.A. Markov, "Selected works" , Moscow-Leningrad (1948) (In Russian) |
| [2] | W.A. [V.A. Markov] Markoff, "Ueber die Funktionen, die in einem gegebenen Intervall möglichst wenig von Null abweichen" Math. Ann. , 77 (1916) pp. 213–258 |
| [3] | I.P. Natanson, "Constructive theory of functions" , 1–2 , F. Ungar (1964–1965) (Translated from Russian) |
Comments
References
| [a1] | E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) |
| [a2] | R.J. Duffin, A.C. Schaeffer, "A refinement of an inequality of the brothers Markoff" Trans. Amer. Math. Soc. , 50 (1941) pp. 517–528 |
| [a3] | A. Schönhage, "Approximationstheorie" , de Gruyter (1971) |
How to Cite This Entry:
Markov inequality. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_inequality&oldid=18236
Markov inequality. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_inequality&oldid=18236
This article was adapted from an original article by N.P. KorneichukV.P. Motornyi (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article