Difference between revisions of "Banach indicatrix"
m |
m |
||
| Line 6: | Line 6: | ||
N(y,f) = +\infty, | N(y,f) = +\infty, | ||
$$ | $$ | ||
| − | |||
and if it has no roots, then | and if it has no roots, then | ||
| − | + | $$ | |
| + | N(y,f) = 0. | ||
| + | $$ | ||
The function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b0151509.png" /> was defined by S. Banach [[#References|[1]]] (see also [[#References|[2]]]). He proved that the indicatrix <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b01515010.png" /> of any continuous function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b01515011.png" /> in the interval <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b01515012.png" /> is a function of Baire class not higher than 2, and | The function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b0151509.png" /> was defined by S. Banach [[#References|[1]]] (see also [[#References|[2]]]). He proved that the indicatrix <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b01515010.png" /> of any continuous function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b01515011.png" /> in the interval <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b015/b015150/b01515012.png" /> is a function of Baire class not higher than 2, and | ||
Revision as of 03:07, 15 May 2015
multiplicity function, of a continuous function $y=f(x)$, $a\leq x\leq b$
An integer-valued function $N(y,f)$, $-\infty < y < \infty$, equal to the number of roots of the equation $f(x)=y$. If, for a given value of $y$, this equation has an infinite number of roots, then $$ N(y,f) = +\infty, $$
and if it has no roots, then
$$ N(y,f) = 0. $$
The function 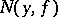 was defined by S. Banach [1] (see also [2]). He proved that the indicatrix
was defined by S. Banach [1] (see also [2]). He proved that the indicatrix 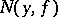 of any continuous function
of any continuous function 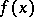 in the interval
in the interval 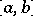 is a function of Baire class not higher than 2, and
is a function of Baire class not higher than 2, and
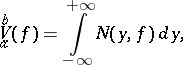 | (*) |
where 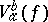 is the variation of
is the variation of 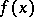 on
on  . Thus, equation (*) can be considered as the definition of the variation of a continuous function
. Thus, equation (*) can be considered as the definition of the variation of a continuous function 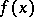 . The Banach indicatrix is also defined (preserving equation (*)) for functions with discontinuities of the first kind [3]. The concept of a Banach indicatrix was employed to define the variation of functions in several variables [4], [5].
. The Banach indicatrix is also defined (preserving equation (*)) for functions with discontinuities of the first kind [3]. The concept of a Banach indicatrix was employed to define the variation of functions in several variables [4], [5].
References
| [1] | S. Banach, "Sur les lignes rectifiables et les surfaces dont l'aire est finie" Fund. Math. , 7 (1925) pp. 225–236 |
| [2] | I.P. Natanson, "Theorie der Funktionen einer reellen Veränderlichen" , H. Deutsch , Frankfurt a.M. (1961) (Translated from Russian) |
| [3] | S.M. Lozinskii, "On the Banach indicatrix" Vestnik Leningrad. Univ. Math. Mekh. Astr. , 7 : 2 pp. 70–87 (In Russian) |
| [4] | A.S. Kronrod, "On functions of two variables" Uspekhi Mat. Nauk , 5 : 1 (1950) pp. 24–134 (In Russian) |
| [5] | A.G. Vitushkin, "On higher-dimensional variations" , Moscow (1955) (In Russian) |
Comments
More generally, for any mapping 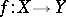 define
define 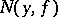 analogously. Then, let
analogously. Then, let 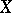 be a separable metric space and let
be a separable metric space and let  be
be 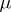 -measurable for all Borel subsets
-measurable for all Borel subsets 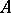 of
of  . Let
. Let 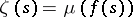 for
for 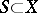 and let
and let 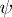 be the measure on
be the measure on 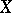 defined by the Carathéodory construction from
defined by the Carathéodory construction from 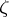 . Then
. Then
 |
for every Borel set 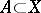 . Cf. [a1], p. 176 ff. For significant extension of (*), cf. [a2].
. Cf. [a1], p. 176 ff. For significant extension of (*), cf. [a2].
References
| [a1] | H. Federer, "Geometric measure theory" , Springer (1969) |
| [a2] | H. Federer, "An analytic characterization of distributions whose partial derivatives are representable by measures" Bull. Amer. Math. Soc. , 60 (1954) pp. 339 |
Banach indicatrix. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Banach_indicatrix&oldid=36407