Difference between revisions of "Projective module"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 4: | Line 4: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Cartan, S. Eilenberg, "Homological algebra" , Princeton Univ. Press (1956) {{MR|0077480}} {{ZBL|0075.24305}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J. Kaplansky, "Projective modules" ''Ann. of Math.'' , '''68''' : 2 (1958) pp. 372–377 {{MR|0100017}} {{ZBL|0083.25802}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A.A. Suslin, "Projective modules over a polynomial ring are free" ''Soviet Math. Dokl.'' , '''17''' : 4 (1976) pp. 1160–1164 ''Dokl. Akad. Nauk SSSR'' , '''229''' : 5 (1976) pp. 1063–1066 {{MR|469905}} {{ZBL|0354.13010}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> D. Quillen, "Projective modules over polynomial rings" ''Invent. Math.'' , '''36''' (1976) pp. 167–171 {{MR|0427303}} {{ZBL|0337.13011}} </TD></TR></table> |
| Line 24: | Line 24: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> H. Bass, "Algebraic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p075/p075280/p07528077.png" />-theory" , Benjamin (1968) {{MR|249491}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.-P. Serre, "Faisceaux algébriques cohérents" ''Ann. of Math.'' , '''61''' (1975) pp. 197–278 {{MR|0068874}} {{ZBL|0067.16201}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> T.Y. Lam, "Serre's conjecture" , Springer (1978)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> M. Ojanguran, R. Sridharan, "Cancellation of Azumaya algebras" ''J. of Algebra'' , '''18''' (1971) pp. 501–505 {{MR|276271}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> E. Kunz, "Introduction to commutative algebra and algebraic geometry" , Birkhäuser (1985) {{MR|0789602}} {{ZBL|0563.13001}} </TD></TR></table> |
Revision as of 21:55, 30 March 2012
A module 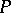 satisfying any of the following equivalent conditions: 1) for any epimorphism
satisfying any of the following equivalent conditions: 1) for any epimorphism 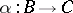 of modules and any homomorphism
of modules and any homomorphism  there is a homomorphism
there is a homomorphism 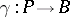 such that
such that 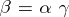 ; 2) the module
; 2) the module 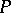 is a direct summand of a free module; 3) the functor
is a direct summand of a free module; 3) the functor 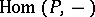 is exact (cf. Exact functor); or 4) any epimorphism
is exact (cf. Exact functor); or 4) any epimorphism 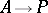 of modules splits.
of modules splits.
Kaplansky's theorem [2], asserting that every projective module is a direct sum of projective modules with countably many generators, reduces the study of the structure of projective modules to the countable case. Projective modules with finitely many generators are studied in algebraic 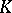 -theory. The simplest example of a projective module is a free module. Over rings decomposable into a direct sum there always exist projective modules different from free ones. The coincidence of the class of projective modules and that of free modules has been proved for local rings [2], and for rings of polynomials in several variables over a field (see [3], [4]).
-theory. The simplest example of a projective module is a free module. Over rings decomposable into a direct sum there always exist projective modules different from free ones. The coincidence of the class of projective modules and that of free modules has been proved for local rings [2], and for rings of polynomials in several variables over a field (see [3], [4]).
References
| [1] | H. Cartan, S. Eilenberg, "Homological algebra" , Princeton Univ. Press (1956) MR0077480 Zbl 0075.24305 |
| [2] | J. Kaplansky, "Projective modules" Ann. of Math. , 68 : 2 (1958) pp. 372–377 MR0100017 Zbl 0083.25802 |
| [3] | A.A. Suslin, "Projective modules over a polynomial ring are free" Soviet Math. Dokl. , 17 : 4 (1976) pp. 1160–1164 Dokl. Akad. Nauk SSSR , 229 : 5 (1976) pp. 1063–1066 MR469905 Zbl 0354.13010 |
| [4] | D. Quillen, "Projective modules over polynomial rings" Invent. Math. , 36 (1976) pp. 167–171 MR0427303 Zbl 0337.13011 |
Comments
The theorem that over a ring 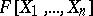 of polynomials in several variables over a field every finitely-generated projective module is free is known as the Quillen–Suslin theorem. The question was raised by J.P. Serre in 1955, [a2], and the statement is also still known as Serre's conjecture. For a complete and detailed discussion, cf. [a3].
of polynomials in several variables over a field every finitely-generated projective module is free is known as the Quillen–Suslin theorem. The question was raised by J.P. Serre in 1955, [a2], and the statement is also still known as Serre's conjecture. For a complete and detailed discussion, cf. [a3].
In [a5], the Quillen–Suslin theorem is formulated as: If 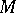 is a finitely-generated projective
is a finitely-generated projective 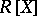 -module and
-module and 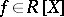 is a monic polynomial such that
is a monic polynomial such that 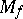 is a free
is a free 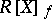 -module, then
-module, then 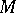 is a free
is a free 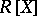 -module.
-module.
Quillen's proof of the Quillen–Suslin theorem uses Horrock's theorem: Let 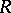 be a commutative local ring and
be a commutative local ring and 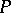 a finitely-generated projective module over
a finitely-generated projective module over 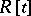 . Then if
. Then if 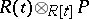 is a free
is a free 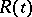 -module,
-module, 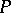 is a free
is a free  -module. A second main ingredient is Quillen's patching theorem. Let
-module. A second main ingredient is Quillen's patching theorem. Let  be a ring. An
be a ring. An  -module
-module 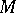 is extended (from
is extended (from 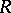 ) if there exists an
) if there exists an 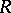 -module
-module 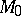 such that
such that  . The patching theorem now says that if
. The patching theorem now says that if 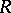 is a commutative ring and
is a commutative ring and 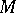 is a finitely-presented
is a finitely-presented 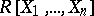 -module, then
-module, then  is extended from
is extended from 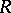 if and only if for every maximal ideal
if and only if for every maximal ideal 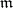 of
of 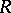 the localization
the localization 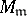 is extended from
is extended from 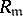 . In this terminology one has a generalized Quillen–Suslin theorem: If
. In this terminology one has a generalized Quillen–Suslin theorem: If 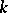 is a commutative regular ring of Krull dimension 2, then every finitely-generated projective module over
is a commutative regular ring of Krull dimension 2, then every finitely-generated projective module over  is extended from
is extended from 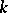 .
.
The Murthy–Horrock theorem says that every finitely-generated projective module over 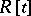 is free if
is free if 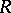 is a commutative regular local ring of Krull dimension 2.
is a commutative regular local ring of Krull dimension 2.
The Suslin monic polynomial theorem played a major role in the study of cancellation theorems over 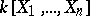 . (Cancellation theorems are theorems of the type: If
. (Cancellation theorems are theorems of the type: If 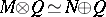 , then
, then 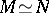 . For instance, there is the Bass cancellation theorem, which says that if
. For instance, there is the Bass cancellation theorem, which says that if 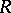 is a commutative Noetherian ring of Krull dimension
is a commutative Noetherian ring of Krull dimension  and
and 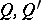 are finitely-generated projective modules which are stably isomorphic, i.e.
are finitely-generated projective modules which are stably isomorphic, i.e. 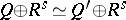 for some
for some  , and the rank of
, and the rank of  is
is 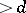 , then
, then 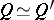 .) The monic polynomial theorem says that if
.) The monic polynomial theorem says that if 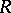 is a commutative Noetherian ring of Krull dimension
is a commutative Noetherian ring of Krull dimension 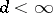 and
and  is an ideal in
is an ideal in 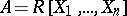 of height
of height 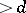 , then there exist new variables
, then there exist new variables  in
in 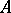 such that
such that 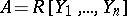 and such that
and such that  contains a polynomial which is monic as a polynomial in
contains a polynomial which is monic as a polynomial in  . For
. For 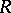 a field this essentially becomes the Noether normalization theorem.
a field this essentially becomes the Noether normalization theorem.
A commutative ring 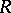 is said to be a Hermite ring if every finitely-generated stably free module
is said to be a Hermite ring if every finitely-generated stably free module 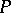 (i.e.
(i.e. 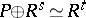 for some
for some 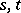 ) is free.
) is free.
Serre's conjecture does not necessarily hold for 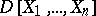 if
if  and
and  is a (non-commutation) division ring, [a4]. The quadratic analogue of Serre's conjecture asks whether a finitely-generated projective module over
is a (non-commutation) division ring, [a4]. The quadratic analogue of Serre's conjecture asks whether a finitely-generated projective module over 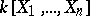 equipped with a quadratic, symmetric bilinear, or symplectic form is necessarily extended from a similar object over
equipped with a quadratic, symmetric bilinear, or symplectic form is necessarily extended from a similar object over 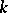 . This is not always the case, cf. [a3], Chapt. VI, for more details.
. This is not always the case, cf. [a3], Chapt. VI, for more details.
References
| [a1] | H. Bass, "Algebraic 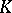 -theory" , Benjamin (1968) MR249491 -theory" , Benjamin (1968) MR249491 |
| [a2] | J.-P. Serre, "Faisceaux algébriques cohérents" Ann. of Math. , 61 (1975) pp. 197–278 MR0068874 Zbl 0067.16201 |
| [a3] | T.Y. Lam, "Serre's conjecture" , Springer (1978) |
| [a4] | M. Ojanguran, R. Sridharan, "Cancellation of Azumaya algebras" J. of Algebra , 18 (1971) pp. 501–505 MR276271 |
| [a5] | E. Kunz, "Introduction to commutative algebra and algebraic geometry" , Birkhäuser (1985) MR0789602 Zbl 0563.13001 |
Projective module. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Projective_module&oldid=12996