Hitchin system

An algebraically completely integrable Hamiltonian system defined on the cotangent bundle to the moduli space of stable vector bundles (of fixed rank and degree; cf. also Vector bundle) over a given Riemann surface 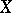of genus 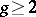. Hitchin's definition of the system [a9] greatly enhanced the theory of spectral curves [a8], which underlies the discovery of a multitude of algebraically completely integrable systems in the 1970s. Such systems are given by a Lax-pair equation: 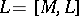with 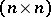-matrices 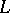, 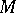depending on a parameter , the spectral curve is an -fold covering of the parameter space and the system lives on a co-adjoint orbit in a loop algebra, by the Adler–Kostant–Symes method of symplectic reduction, cf. [a1]. N.J. Hitchin defines the curve of eigenvalues on the total space of the canonical bundle of 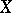, and linearizes the flows on the Jacobi variety of this curve.

The idea gave rise to a great amount of algebraic geometry: moduli spaces of stable pairs [a12]; meromorphic Hitchin systems [a3] and [a4]; Hitchin systems for principal 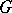-bundles [a5]; and quantized Hitchin systems with applications to the geometric Langlands program [a2].

Moreover, by moving the curve 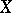in moduli, Hitchin [a10] achieved geometric quantization by constructing a projective connection over the spaces of bundles, whose associated heat operator generalizes the heat equation that characterizes the Riemann theta-function for the case of rank-one bundles. The coefficients of the heat operator are given by the Hamiltonians of the Hitchin systems.

Explicit formulas for the Hitchin Hamiltonian and connection were produced for the genus-two case [a7], [a6]. A connection of Hitchin's Hamiltonians with KP-flows (cf. also KP-equation) is given in [a4] and [a11].

References

[a1]	M.R. Adams, J. Harnad, J. Hurtubise, "Integrable Hamiltonian systems on rational coadjoint orbits of loop algebras, Hamiltonian systems, transformation groups and spectral transform methods" , Proc. CRM Workshop, Montreal 1989 (1990) pp. 19–32
[a2]	A.A. Beilinson, V.G. Drinfel'd, "Quantization of Hitchin's fibration and Langlands program" A. Boutet de Monvel (ed.) et al. (ed.) , Algebraic and Geometric Methods in Math. Physics. Proc. 1st Ukrainian–French–Romanian Summer School, Kaciveli, Ukraine, Sept. 1-14 1993 , Math. Phys. Stud. , 19 , Kluwer Acad. Publ. (1996) pp. 3–7 MR1385674
[a3]	F. Bottacin, "Symplectic geometry on moduli spaces of stable pairs" Ann. Sci. Ecole Norm. Sup. 4 , 28 (1995) pp. 391–433 MR1334607 Zbl 0864.14004
[a4]	R. Donagi, E. Markman, "Spectral covers, algebraically completely integrable, Hamiltonian systems, and moduli of bundles" M. Francaviglia (ed.) et al. (ed.) , Integrable Systems and Quantum Groups. Lectures at the 1st session of the Centro Internaz. Mat. Estivo (CIME), Montecatini Terme, Italy, June 14-22 1993 , Lecture Notes Math. , 1620 , Springer (1996) pp. 1–119 MR1397273 Zbl 0853.35100
[a5]	G. Faltings, "Stable G-bundles and projective connections" J. Alg. Geometry , 2 (1993) pp. 507–568 MR1211997 Zbl 0790.14019
[a6]	B. van Geemen, A.J. de Jong, "On Hitchin's connection" J. Amer. Math. Soc. , 11 (1998) pp. 189–228
[a7]	B. van Geemen, E. Previato, "On the Hitchin system" Duke Math. J. , 85 : 3 (1996) pp. 659–683 MR1422361 Zbl 0879.14010
[a8]	N.J. Hitchin, "The self-duality equations on a Riemann surface" Proc. London Math. Soc. , 55 (1987) pp. 59–126 MR0887284 Zbl 0634.53045
[a9]	N.J. Hitchin, "Stable bundles and integrable systems" Duke Math. J. , 54 (1987) pp. 91–114 MR0885778 Zbl 0627.14024
[a10]	N.J. Hitchin, "Flat connections and geometric quantization" Comm. Math. Phys. , 131 : 2 (1990) pp. 347–380 MR1065677 Zbl 0718.53021
[a11]	Yingchen Li, M. Mulase, "Hitchin systems and KP equations" Internat. J. Math. , 7 : 2 (1996) pp. 227–244 MR1382724 Zbl 0863.58036
[a12]	C.T. Simpson, "Moduli of representations of the fundamental group of a smooth projective variety I–II" Publ. Math. IHES , 79/80 (1994/5) pp. 47–129;5–79