Difference between revisions of "Continuous lattice"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 11: | Line 11: | ||
In the lattice of all open sets of a [[Topological space|topological space]], it is easy to see that the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570050.png" /> holds if there is a compact set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570051.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570052.png" />; from this it follows that the open-set lattice of a [[Locally compact space|locally compact space]] (or of a locally quasi-compact space) is continuous. Conversely, the continuous lattices which are (finitely) distributive are exactly the open-set lattices of locally compact topological spaces [[#References|[a4]]]. The continuity of the open-set lattices of locally compact spaces is closely related to the good behaviour of function spaces when the domain space is locally compact [[#References|[a5]]], [[#References|[a6]]]. | In the lattice of all open sets of a [[Topological space|topological space]], it is easy to see that the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570050.png" /> holds if there is a compact set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570051.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570052.png" />; from this it follows that the open-set lattice of a [[Locally compact space|locally compact space]] (or of a locally quasi-compact space) is continuous. Conversely, the continuous lattices which are (finitely) distributive are exactly the open-set lattices of locally compact topological spaces [[#References|[a4]]]. The continuity of the open-set lattices of locally compact spaces is closely related to the good behaviour of function spaces when the domain space is locally compact [[#References|[a5]]], [[#References|[a6]]]. | ||
| − | Iterating a natural morphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570053.png" /> and using an inverse limit construction, Scott imbedded any continuous lattice in a continuous lattice <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570054.png" /> which is naturally isomorphic to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570055.png" />. Every element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570056.png" /> in such a continuous lattice <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570057.png" /> may thus be regarded as a self-map of this domain and vice versa — provided that | + | Iterating a natural morphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570053.png" /> and using an inverse limit construction, Scott imbedded any continuous lattice in a continuous lattice <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570054.png" /> which is naturally isomorphic to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570055.png" />. Every element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570056.png" /> in such a continuous lattice <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570057.png" /> may thus be regarded as a self-map of this domain and vice versa — provided that "self-maps" are morphisms in the category. As a consequence, self-application of the type <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570058.png" /> is well-defined. Therefore, the continuous lattices <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570059.png" /> are models of the [[Lambda-calculus|<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570060.png" />-calculus]]. |
A generalization of the concept of a continuous lattice is that of a continuous poset [[#References|[a7]]]. A further generalization, to continuous categories, has also been studied, [[#References|[a8]]]. A continuous poset is defined by replacing in the definition of continuous lattice the requirement that all subsets of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570061.png" /> have suprema by the weaker assumption that all non-empty upwards directed sets have suprema and that all sets <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570062.png" /> are upwards directed. | A generalization of the concept of a continuous lattice is that of a continuous poset [[#References|[a7]]]. A further generalization, to continuous categories, has also been studied, [[#References|[a8]]]. A continuous poset is defined by replacing in the definition of continuous lattice the requirement that all subsets of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570061.png" /> have suprema by the weaker assumption that all non-empty upwards directed sets have suprema and that all sets <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c025/c025700/c02570062.png" /> are upwards directed. | ||
| Line 21: | Line 21: | ||
A detailed account of continuous lattice theory is given in [[#References|[a9]]], and a more concise one in [[#References|[a10]]], Chapt. 7. Reference [[#References|[a11]]] discusses some more recent developments in the subject and contains an extensive bibliography on continuous lattices up to 1985. | A detailed account of continuous lattice theory is given in [[#References|[a9]]], and a more concise one in [[#References|[a10]]], Chapt. 7. Reference [[#References|[a11]]] discusses some more recent developments in the subject and contains an extensive bibliography on continuous lattices up to 1985. | ||
| − | Continuous lattices were introduced by Dana S. Scott [[#References|[a1]]], [[#References|[a12]]] as a basis for an abstract theory of computation by which a | + | Continuous lattices were introduced by Dana S. Scott [[#References|[a1]]], [[#References|[a12]]] as a basis for an abstract theory of computation by which a "computation" is approximated better and better by "finite approximations" . |
Continuous lattices should not be confused with continuous geometries, which are complete lattices of an entirely different type. Cf. [[Orthomodular lattice|Orthomodular lattice]] for the notion of continuous geometry. | Continuous lattices should not be confused with continuous geometries, which are complete lattices of an entirely different type. Cf. [[Orthomodular lattice|Orthomodular lattice]] for the notion of continuous geometry. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> D.S. Scott, "Continuous lattices" F.W. Lawvere (ed.) , ''Toposes, algebraic geometry and logic (Dalhousic Univ., Jan. 1971)'' , ''Lect. notes in math.'' , '''274''' , Springer (1972) pp. 97–136 {{MR|0404073}} {{ZBL|0239.54006}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.D. Lawson, "Topological semilattices with small semilattices" ''J. Lond. Math. Soc. (2)'' , '''1''' (1969) pp. 719–724 {{MR|0253301}} {{ZBL|0185.03801}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> K.H. Hofmann, A.R. Stralka, "The algebraic theory of Lawson semilattices" ''Diss. Math.'' , '''137''' (1976) pp. 1–54 {{MR|}} {{ZBL|0359.06016}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> K.H. Hofmann, J.D. Lawson, "The spectral theory of distributive continuous lattices" ''Trans. Amer. Math. Soc.'' , '''246''' (1978) pp. 285–310 {{MR|0515540}} {{ZBL|0402.54043}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> B.J. Day, G.M. Kelly, "On topological quotient maps preserved by pullbacks or products" ''Proc. Cambridge Philos. Soc.'' , '''67''' (1970) pp. 553–558 {{MR|}} {{ZBL|0191.20801}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> J.R. Isbell, "General function spaces, products and continuous lattices" ''Math. Proc. Cambridge Philos. Soc.'' , '''100''' (1986) pp. 193–205 {{MR|0848846}} {{ZBL|0609.54010}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> G. Markowsky, "A motivation and generalization of Scott's notion of a continuous lattice" , ''Continuous lattices'' , ''Lect. notes in math.'' , '''871''' , Springer (1981) pp. 298–307</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> P.T. Johnstone, A. Joyal, "Continuous categories and exponentiable toposes" ''J. Pure Appl. Alg.'' , '''25''' (1982) pp. 255–296 {{MR|0666021}} {{ZBL|0487.18003}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> G. Gierz, K.H. Hofmann, K. Keimel, J.D. Lawson, M.V. Mislove, D.S. Scott, "A compendium of continuous lattices" , Springer (1980) {{MR|0614752}} {{ZBL|0452.06001}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> P.T. Johnstone, "Stone spaces" , Cambridge Univ. Press (1982) {{MR|0698074}} {{ZBL|0499.54001}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> R.-E. Hoffman (ed.) K.H. Hofmann (ed.) , ''Continuous lattices and their applications'' , M. Dekker (1985)</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> D.S. Scott, "Outline of a mathematical theory of computations" , ''Proc. 4-th Annual Princeton Conf. Information Sc. and Systems'' , Princeton Univ. Press (1970) pp. 169–176</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> B. Banaschewski (ed.) R.-E. Hoffmann (ed.) , ''Continuous lattices'' , ''Lect. notes in math.'' , '''871''' , Springer (1981) {{MR|0646068}} {{ZBL|0469.06007}} {{ZBL|0452.00011}} </TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> D. Schmidt, "Denotational semantics: An introduction" , Allyn & Bacon (1986)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> J.E. Stoy, "Denotational semantics: The Scott–Strachey approach to programming language theory" , M.I.T. (1979) {{MR|0629830}} {{MR|0488969}} {{ZBL|0503.68059}} </TD></TR></table> |
Revision as of 21:51, 30 March 2012
A type of complete lattice, first studied under this name by D.S. Scott [a1], [a12], examples of which occur in many areas of algebra, analysis and topology. Continuous lattices are usually defined in terms of an auxiliary relation, the way-below relation, which is definable in any complete lattice 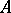 : One says that an element
: One says that an element  is way below an element
is way below an element  (and writes
(and writes  ) if, whenever
) if, whenever  is a directed subset of
is a directed subset of  with
with 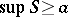 , there exists an
, there exists an  with
with 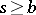 . The elements way below a given element
. The elements way below a given element  form an ideal
form an ideal 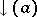 (in fact the intersection of all those ideals
(in fact the intersection of all those ideals 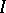 with
with  ); one says that
); one says that 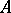 is continuous if
is continuous if 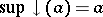 for every
for every 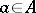 . Thus, a continuous lattice is a partially ordered set in which every subset has a least upper bound and in which every element is the least upper bound of all elements way below it. An element
. Thus, a continuous lattice is a partially ordered set in which every subset has a least upper bound and in which every element is the least upper bound of all elements way below it. An element  in a complete lattice is compact, or finite, if and only if
in a complete lattice is compact, or finite, if and only if 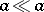 ; from this it follows that an algebraic lattice is continuous, and in fact continuous lattices may be characterized as those complete lattices which are retracts of algebraic lattices by mappings preserving directed sups. Continuous lattices may also be characterized equationally: they are precisely those complete lattices which satisfy all those instances of the complete distributive law
; from this it follows that an algebraic lattice is continuous, and in fact continuous lattices may be characterized as those complete lattices which are retracts of algebraic lattices by mappings preserving directed sups. Continuous lattices may also be characterized equationally: they are precisely those complete lattices which satisfy all those instances of the complete distributive law
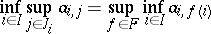 |
(where 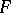 is the set of choice functions
is the set of choice functions  ) for which the set
) for which the set  is (upwards) directed for each
is (upwards) directed for each 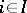 . In particular, a completely distributive lattice is continuous; conversely, one can show that if
. In particular, a completely distributive lattice is continuous; conversely, one can show that if 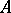 is a (finitely) distributive complete lattice such that both
is a (finitely) distributive complete lattice such that both  and
and 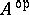 are continuous, then
are continuous, then  is completely distributive.
is completely distributive.
If 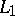 and
and 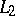 are continuous lattices, then the set
are continuous lattices, then the set  of all functions from
of all functions from 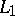 to
to 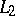 preserving suprema of upwards directed sets is a continuous lattice with respect to the pointwise partial order. The category of continuous lattices and such functions is a Cartesian-closed category (cf. Closed category).
preserving suprema of upwards directed sets is a continuous lattice with respect to the pointwise partial order. The category of continuous lattices and such functions is a Cartesian-closed category (cf. Closed category).
There are two intrinsic topologies which are of importance in the study of continuous lattices. The Scott topology on a complete lattice  has for its open sets those
has for its open sets those  which are upper sets (i.e.
which are upper sets (i.e. 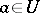 and
and 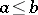 implies
implies 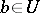 ) and inaccessible by directed sups (i.e.
) and inaccessible by directed sups (i.e.  directed and
directed and  implies
implies 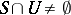 ). A function between complete lattices is continuous for their Scott topologies if and only if it preserves directed sups; thus the Scott-open subsets of
). A function between complete lattices is continuous for their Scott topologies if and only if it preserves directed sups; thus the Scott-open subsets of 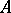 are precisely those whose characteristic functions are Scott-continuous mappings
are precisely those whose characteristic functions are Scott-continuous mappings 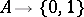 . Equipped with their Scott topologies, continuous lattices are precisely the injective objects (with respect to subspace inclusions, cf. Injective object) in the category of
. Equipped with their Scott topologies, continuous lattices are precisely the injective objects (with respect to subspace inclusions, cf. Injective object) in the category of 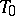 topological spaces [a1]; equivalently, they are precisely the retracts of powers of the Sierpiński space (the latter being the two-element lattice
topological spaces [a1]; equivalently, they are precisely the retracts of powers of the Sierpiński space (the latter being the two-element lattice  equipped with its Scott topology). The second intrinsic topology, the Lawson topology, has as a subbase of open sets the Scott-open sets together with the complements of principal filters (i.e. the sets
equipped with its Scott topology). The second intrinsic topology, the Lawson topology, has as a subbase of open sets the Scott-open sets together with the complements of principal filters (i.e. the sets  ,
, 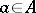 ). It is Hausdorff (unlike the Scott topology) and compact, and the binary meet operation
). It is Hausdorff (unlike the Scott topology) and compact, and the binary meet operation  is continuous for it; moreover, continuous lattices equipped with their Lawson topologies may be characterized as those compact Hausdorff topological meet semi-lattices for which the continuous semi-lattice of homomorphisms into the unit interval
is continuous for it; moreover, continuous lattices equipped with their Lawson topologies may be characterized as those compact Hausdorff topological meet semi-lattices for which the continuous semi-lattice of homomorphisms into the unit interval  separate points (these are sometimes called (compact) Lawson semi-lattices) [a2], [a3]. From this, one obtains yet another, purely lattice-theoretic, characterization of continuous lattices: up to isomorphism, they are precisely the subsets of powers of
separate points (these are sometimes called (compact) Lawson semi-lattices) [a2], [a3]. From this, one obtains yet another, purely lattice-theoretic, characterization of continuous lattices: up to isomorphism, they are precisely the subsets of powers of  which are closed under directed sups and arbitrary infs.
which are closed under directed sups and arbitrary infs.
In the lattice of all open sets of a topological space, it is easy to see that the relation  holds if there is a compact set
holds if there is a compact set  with
with  ; from this it follows that the open-set lattice of a locally compact space (or of a locally quasi-compact space) is continuous. Conversely, the continuous lattices which are (finitely) distributive are exactly the open-set lattices of locally compact topological spaces [a4]. The continuity of the open-set lattices of locally compact spaces is closely related to the good behaviour of function spaces when the domain space is locally compact [a5], [a6].
; from this it follows that the open-set lattice of a locally compact space (or of a locally quasi-compact space) is continuous. Conversely, the continuous lattices which are (finitely) distributive are exactly the open-set lattices of locally compact topological spaces [a4]. The continuity of the open-set lattices of locally compact spaces is closely related to the good behaviour of function spaces when the domain space is locally compact [a5], [a6].
Iterating a natural morphism  and using an inverse limit construction, Scott imbedded any continuous lattice in a continuous lattice
and using an inverse limit construction, Scott imbedded any continuous lattice in a continuous lattice 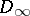 which is naturally isomorphic to
which is naturally isomorphic to  . Every element
. Every element 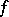 in such a continuous lattice
in such a continuous lattice 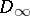 may thus be regarded as a self-map of this domain and vice versa — provided that "self-maps" are morphisms in the category. As a consequence, self-application of the type
may thus be regarded as a self-map of this domain and vice versa — provided that "self-maps" are morphisms in the category. As a consequence, self-application of the type 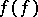 is well-defined. Therefore, the continuous lattices
is well-defined. Therefore, the continuous lattices 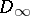 are models of the
are models of the 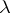 -calculus.
-calculus.
A generalization of the concept of a continuous lattice is that of a continuous poset [a7]. A further generalization, to continuous categories, has also been studied, [a8]. A continuous poset is defined by replacing in the definition of continuous lattice the requirement that all subsets of  have suprema by the weaker assumption that all non-empty upwards directed sets have suprema and that all sets
have suprema by the weaker assumption that all non-empty upwards directed sets have suprema and that all sets 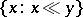 are upwards directed.
are upwards directed.
Continuous posets allow a theory of the type of Pontryagin duality. The lattice 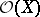 of open subsets of a Hausdorff space is a continuous lattice if and only if
of open subsets of a Hausdorff space is a continuous lattice if and only if 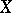 is locally compact. In this case its Pontryagin dual is the set of compact subsets with reversed inclusion as order relation.
is locally compact. In this case its Pontryagin dual is the set of compact subsets with reversed inclusion as order relation.
A domain is a continuous poset in which, first, every element is the supremum of compact elements, secondly, there are countably many compact elements, and, finally, every set which has an upper bound has a supremum. Domains have applications in the semantics of high-level programming languages; introductions into this aspect can be found in [a14] and [a15].
A detailed account of continuous lattice theory is given in [a9], and a more concise one in [a10], Chapt. 7. Reference [a11] discusses some more recent developments in the subject and contains an extensive bibliography on continuous lattices up to 1985.
Continuous lattices were introduced by Dana S. Scott [a1], [a12] as a basis for an abstract theory of computation by which a "computation" is approximated better and better by "finite approximations" .
Continuous lattices should not be confused with continuous geometries, which are complete lattices of an entirely different type. Cf. Orthomodular lattice for the notion of continuous geometry.
References
| [a1] | D.S. Scott, "Continuous lattices" F.W. Lawvere (ed.) , Toposes, algebraic geometry and logic (Dalhousic Univ., Jan. 1971) , Lect. notes in math. , 274 , Springer (1972) pp. 97–136 MR0404073 Zbl 0239.54006 |
| [a2] | J.D. Lawson, "Topological semilattices with small semilattices" J. Lond. Math. Soc. (2) , 1 (1969) pp. 719–724 MR0253301 Zbl 0185.03801 |
| [a3] | K.H. Hofmann, A.R. Stralka, "The algebraic theory of Lawson semilattices" Diss. Math. , 137 (1976) pp. 1–54 Zbl 0359.06016 |
| [a4] | K.H. Hofmann, J.D. Lawson, "The spectral theory of distributive continuous lattices" Trans. Amer. Math. Soc. , 246 (1978) pp. 285–310 MR0515540 Zbl 0402.54043 |
| [a5] | B.J. Day, G.M. Kelly, "On topological quotient maps preserved by pullbacks or products" Proc. Cambridge Philos. Soc. , 67 (1970) pp. 553–558 Zbl 0191.20801 |
| [a6] | J.R. Isbell, "General function spaces, products and continuous lattices" Math. Proc. Cambridge Philos. Soc. , 100 (1986) pp. 193–205 MR0848846 Zbl 0609.54010 |
| [a7] | G. Markowsky, "A motivation and generalization of Scott's notion of a continuous lattice" , Continuous lattices , Lect. notes in math. , 871 , Springer (1981) pp. 298–307 |
| [a8] | P.T. Johnstone, A. Joyal, "Continuous categories and exponentiable toposes" J. Pure Appl. Alg. , 25 (1982) pp. 255–296 MR0666021 Zbl 0487.18003 |
| [a9] | G. Gierz, K.H. Hofmann, K. Keimel, J.D. Lawson, M.V. Mislove, D.S. Scott, "A compendium of continuous lattices" , Springer (1980) MR0614752 Zbl 0452.06001 |
| [a10] | P.T. Johnstone, "Stone spaces" , Cambridge Univ. Press (1982) MR0698074 Zbl 0499.54001 |
| [a11] | R.-E. Hoffman (ed.) K.H. Hofmann (ed.) , Continuous lattices and their applications , M. Dekker (1985) |
| [a12] | D.S. Scott, "Outline of a mathematical theory of computations" , Proc. 4-th Annual Princeton Conf. Information Sc. and Systems , Princeton Univ. Press (1970) pp. 169–176 |
| [a13] | B. Banaschewski (ed.) R.-E. Hoffmann (ed.) , Continuous lattices , Lect. notes in math. , 871 , Springer (1981) MR0646068 Zbl 0469.06007 Zbl 0452.00011 |
| [a14] | D. Schmidt, "Denotational semantics: An introduction" , Allyn & Bacon (1986) |
| [a15] | J.E. Stoy, "Denotational semantics: The Scott–Strachey approach to programming language theory" , M.I.T. (1979) MR0629830 MR0488969 Zbl 0503.68059 |
Continuous lattice. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Continuous_lattice&oldid=17410