Wiener-Hopf method

A method for solving functional equations of the type:

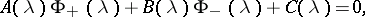(1)

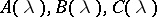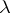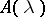where are given functions of a complex variable , analytic in a strip , and and are non-zero in this strip. The functions and 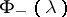are unknown functions of the complex variable 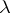which tend to zero as and are to be determined, 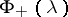being analytic for 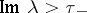and 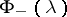being analytic for 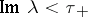. Equation (1) must be satisfied in the entire strip of analyticity .

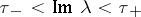The Wiener–Hopf method is based on the following two theorems.

1) A function 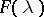which is analytic in the strip 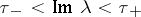and uniformly tends to zero as 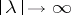can be represented inside this strip as a sum

where is analytic in the half-plane , while is analytic in the half-plane .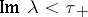

2) A function 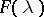which is analytic and non-zero in the strip 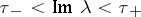and which uniformly tends to one in this strip as 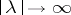is representable in the given strip as a product:

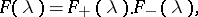(2)

where 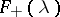and 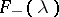are analytic and non-zero in the half-planes 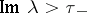and 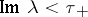, respectively. The representation (2) is often called a factorization of the function .

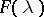The fundamental idea of the Wiener–Hopf method is that it is possible to factorize the function 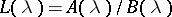; in other words, the method is based on the assumption that a representation

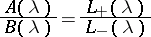(3)

is possible. Using (3), equation (1) may be written as:

Since 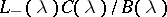is analytic inside the strip, one has

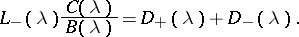(4)

Using (4), one finally obtains equation (1) in the form

(5)

The left-hand side of (5) represents a function which is analytic for , while the right-hand side is a function which is analytic for 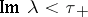. Since they have a common strip of analyticity in which condition (5) is satisfied, there exists a unique entire function 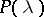which is identical with the left-hand and right-hand sides of (5), respectively, in their domains of analyticity. Hence

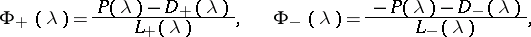i.e. the solution of (1) is unique up to an entire function. If the order of growth of and 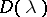is bounded at infinity, must be a polynomial. The functions sought are then determined uniquely up to constants, which are calculated by imposing additional conditions.

The Wiener–Hopf method was developed in [1] to solve special type integral equations (cf. Wiener–Hopf equation). It subsequently found extensive use in various problems of mathematical physics [2].

References

[1]	N. Wiener, E. Hopf, "Ueber eine Klasse singulärer Integralgleichungen" Sitzungber. Akad. Wiss. Berlin (1931) pp. 696–706
[2]	B. Noble, "Methods based on the Wiener–Hopf technique for the solution of partial differential equations" , Pergamon (1958)

Comments

Theorem 2) as stated above is wrong; it requires an additional condition, namely that the winding number of the curve parametrized by 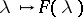, where 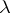runs over the line in the given strip, is equal to zero. So the Wiener–Hopf method described above works only under the additional requirement that the winding number condition is met for 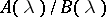. A detailed analysis of the Wiener–Hopf method for various classes of functions (not necessarily analytic on a strip) may be found in [a1]. The matrix-valued version of this theory, which is due to [a2] (see also [a3]), is more complicated and explicit solutions can only obtained in special cases. The case when the functions 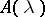and 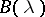appearing in equation (1) are rational matrix functions is of special interest and can be solved explicitly by employing a state-space method which is connected to mathematical systems theory (see [a4], [a5] and Integral equation of convolution type).

References

[a1]	M.G. Krein, "Integral equations on a half-line with kernel depending upon the difference of the arguments" Transl. Amer. Math. Soc. (2) , 22 (1962) pp. 163–288 Uspekhi Mat. Nauk , 13 : 5 (1958) pp. 3–120
[a2]	I.Ts. Gokhberg, M.G. Krein, "Systems of integral equations on a half line with kernels depending on the difference of arguments" Transl. Amer. Math. Soc. (2) , 14 (1960) pp. 217–287 Uspekhi Mat. Nauk , 13 : 2 (80) (1958)
[a3]	I.C. [I.Ts. Gokhberg] Gohberg, I.A. Feld'man, "Convolution equations and projection methods for their solution" , Transl. Math. Monogr. , 41 , Amer. Math. Soc. (1974) (Translated from Russian)
[a4]	H. Bart, I. Gohberg, M.A. Kaashoek, "Minimal factorization of matrix and operation functions" , Birkhäuser (1979)
[a5]	I. Gohberg, M.A. Kaashoek, "The state space method for solving singular integral equations" A.C. Antoulas (ed.) , Mathematical System Theory. The influence of Kalman , Springer (1991) pp. 509–523
[a6]	H. Hochstadt, "Integral equations" , Wiley (1973)